Intro to Statistics Final Exam Answers Guide

Preparing for an assessment in any subject requires a solid understanding of core principles and problem-solving strategies. Whether you’re reviewing complex theories or practicing different types of questions, knowing how to approach the material can make all the difference.

Focus on mastering the fundamentals that are most commonly tested. Gaining clarity in areas like data analysis, probability, and hypothesis testing will allow you to tackle a wide variety of challenges with confidence. Understanding these essential topics helps you build a strong foundation, which is crucial for success.

When you face challenges in applying concepts, it’s important to break them down into manageable parts. By practicing different methods and reviewing key formulas, you can refine your skills and improve your speed and accuracy during the assessment. The goal is not just to memorize, but to truly understand how to use each tool effectively.

Intro to Statistics Final Exam Answers

Successfully completing an assessment in this field requires more than just knowledge; it requires the ability to apply critical thinking to various problems. Whether you’re tasked with analyzing data sets or interpreting research findings, there are a variety of techniques to help you navigate the questions with confidence.

Key Topics to Focus On

To excel, it’s essential to concentrate on the areas most frequently covered. The following concepts are likely to appear and should be thoroughly understood:

Data Analysis – Knowing how to interpret and summarize numerical data is fundamental.
Probability Theory – Be prepared to calculate probabilities and apply different probability distributions.
Hypothesis Testing – Understanding p-values, significance levels, and confidence intervals is key.
Regression Analysis – The ability to analyze and interpret trends through regression models is crucial.
Sampling Methods – Make sure you’re familiar with different sampling techniques and their significance.

Effective Preparation Techniques

To prepare efficiently for the test, it’s essential to use a variety of strategies:

Practice Regularly – Solve as many sample problems as possible to gain familiarity with various question types.
Review Key Formulas – Memorize essential formulas, but focus on understanding how and when to apply them.
Time Management – Practice completing questions within time limits to ensure you can manage your time during the actual test.
Study in Groups – Collaborating with classmates can help clarify difficult concepts and provide new perspectives.

Understanding Key Statistical Concepts

Mastering fundamental ideas in this subject is essential for solving a wide range of problems. A strong grasp of core concepts such as data collection, interpretation, and analysis allows you to approach questions with clarity and precision. These principles are the building blocks for understanding more complex topics and applying them effectively during assessments.

Focus on the following key ideas to strengthen your understanding:

Measures of Central Tendency – The mean, median, and mode provide a way to summarize data in a single value that represents the “center” of a data set.
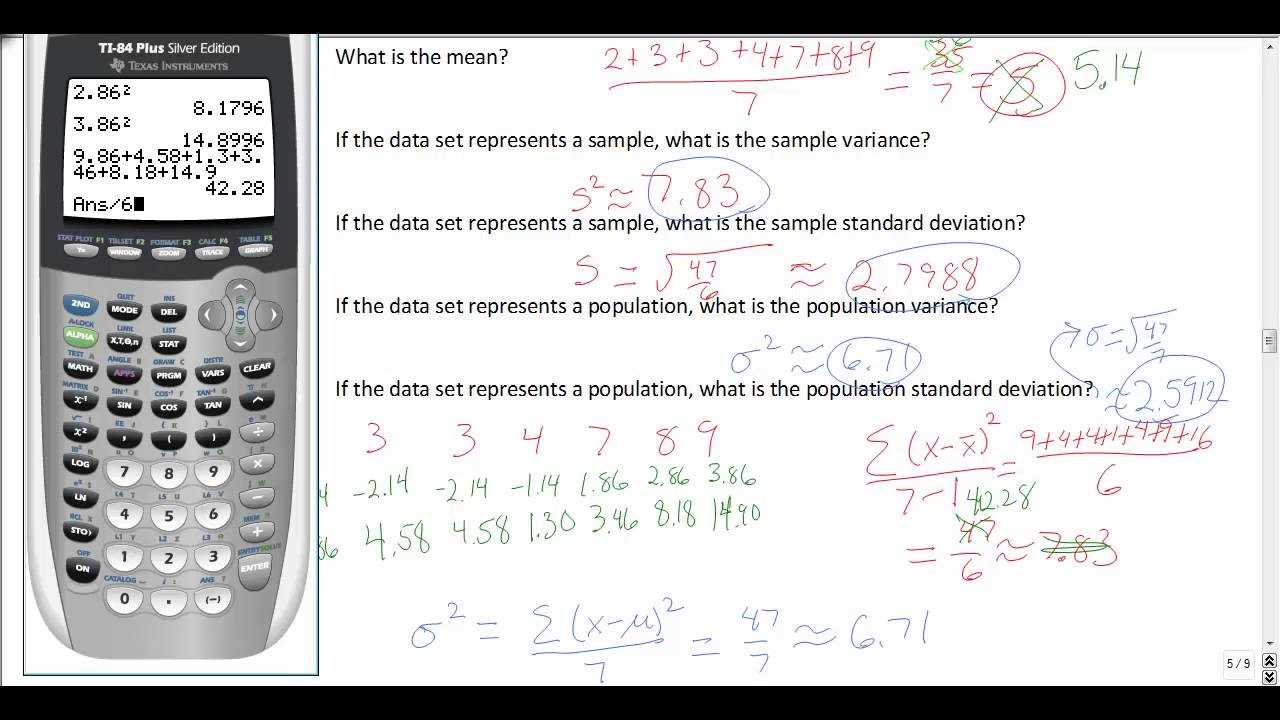
Dispersion – Understanding how data varies or spreads out is crucial. Key measures include range, variance, and standard deviation.
Probability – The study of likelihood helps in predicting outcomes and understanding the distribution of data.
Sampling – Grasping how samples are drawn from populations and the impact of sample size on results is essential.
Correlation and Causation – Recognizing the relationship between variables and distinguishing correlation from causality is vital for interpretation.

By gaining a deeper understanding of these concepts, you’ll be better prepared to interpret data, apply appropriate methods, and analyze results with accuracy and confidence.

Common Challenges in Statistics Exams

Students often encounter several obstacles when dealing with assessments in this subject, ranging from difficulty in applying formulas to interpreting complex data sets. Understanding and addressing these common issues can significantly improve performance and reduce test anxiety. Below are some of the typical challenges faced during such assessments.

Challenge	Description
Complex Problem-Solving	Many questions require the application of multiple concepts in a single problem, making it difficult to know where to start.
Time Pressure	Limited time often forces students to rush through questions, leading to careless mistakes or incomplete answers.
Formula Application	Remembering and correctly applying various formulas, especially in scenarios with intricate calculations, can be overwhelming.
Data Interpretation	Understanding how to read graphs, tables, and other data representations can be a barrier for many students.
Misunderstanding Questions	Misinterpreting a question’s requirements, such as mixing up correlation with causation or overlooking crucial details, is a frequent mistake.

Being aware of these challenges allows students to better prepare by practicing specific techniques, managing their time effectively, and refining their approach to data analysis and interpretation.

Effective Study Strategies for Success

Achieving success in any assessment requires more than just passive reading of material. A focused, organized approach to studying is key. Whether reviewing concepts, practicing problems, or refining test-taking strategies, each step contributes to building a strong foundation and boosting confidence.

Here are some proven techniques to help maximize your preparation:

Active Learning – Engage with the material through active problem solving, summarizing key points, and teaching concepts to others.
Practice Regularly – Solve practice questions and work through sample problems to familiarize yourself with different question formats and types.
Break Down Complex Topics – Divide larger, challenging subjects into smaller, manageable sections for easier comprehension.
Use Spaced Repetition – Review material at increasing intervals to reinforce retention and prevent cramming.
Group Study – Collaborate with classmates to discuss difficult concepts and clarify doubts, as teaching each other can deepen understanding.

By following these strategies, you will be better prepared to face challenging questions and apply your knowledge effectively under time constraints.

Tips for Solving Probability Questions

Solving probability-related problems requires a clear understanding of the underlying principles and a systematic approach to breaking down each question. The key to success lies in accurately applying formulas and logically working through the steps to determine the likelihood of different outcomes.

Here are some helpful tips to guide you when approaching probability problems:

Understand the Basics – Familiarize yourself with key probability concepts, including outcomes, events, and sample spaces.
Identify the Type of Problem – Determine whether the problem involves independent or dependent events, and whether you’re dealing with conditional probability or combinations and permutations.
Use the Right Formula – Make sure you’re applying the correct formula for the given situation, whether it’s for basic probability, compound events, or Bayes’ theorem.
Draw Diagrams – Visual aids like probability trees or Venn diagrams can help you better understand complex relationships between events.
Check for Completeness – Always verify that you have considered all possible outcomes and events before finalizing your answer.

By practicing these techniques and refining your understanding, you’ll be better equipped to solve any probability-based problem with confidence and accuracy.

Mastering Hypothesis Testing Techniques

Hypothesis testing is a crucial method used to assess the validity of claims or assumptions based on data. The ability to correctly apply testing techniques is essential for determining whether observed results are due to chance or if they reflect a true pattern. Understanding the steps involved and recognizing potential pitfalls will significantly improve your accuracy in applying these methods.

Here are some key strategies to help you master hypothesis testing:

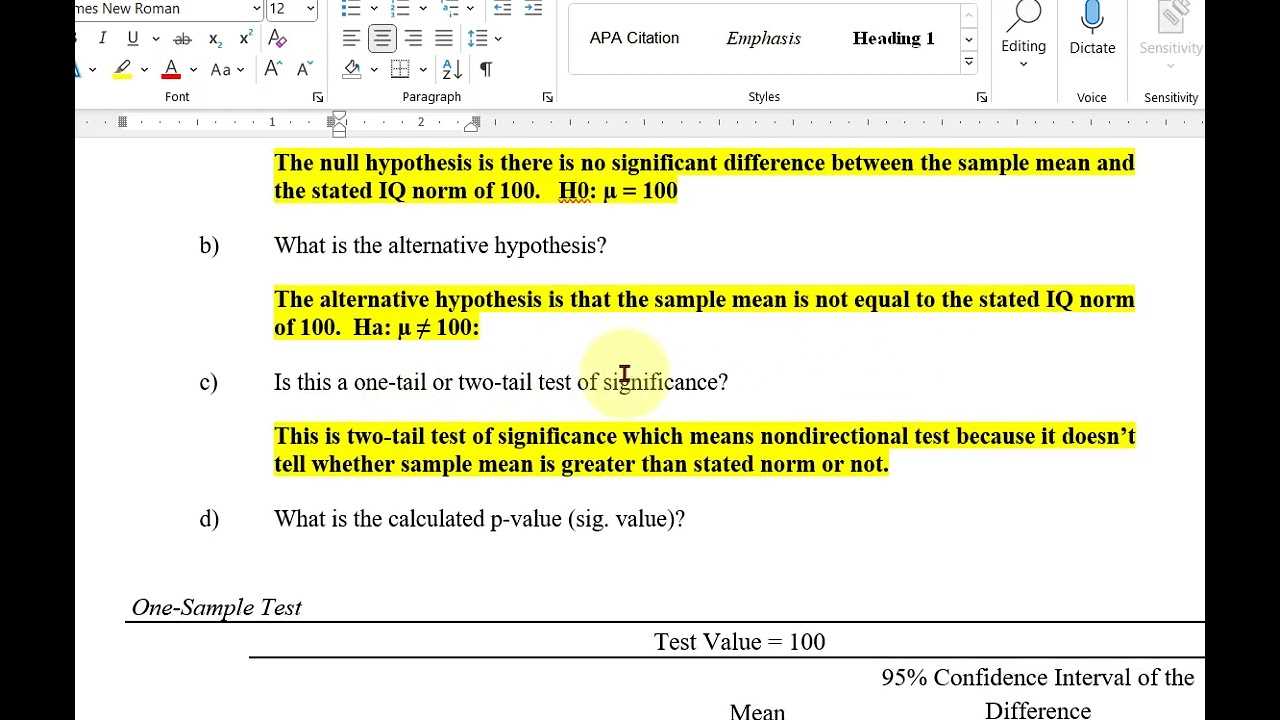
Clearly Define the Hypotheses – Always start by stating both the null hypothesis (H₀) and the alternative hypothesis (H₁) clearly. These will form the basis of your testing.
Choose the Right Test – Select the appropriate test based on your data type and research question, whether it’s a t-test, chi-square test, or ANOVA.
Understand Significance Levels – Be familiar with the significance level (α), typically set at 0.05, which indicates the threshold for rejecting the null hypothesis.
Calculate and Interpret p-Values – The p-value helps determine the strength of evidence against the null hypothesis. A p-value below your chosen significance level suggests a statistically significant result.
Evaluate Assumptions – Each test comes with assumptions (e.g., normality, independence), so check whether your data meets these conditions before proceeding.

By mastering these techniques, you’ll be better equipped to conduct accurate and reliable hypothesis tests, ultimately improving your ability to draw meaningful conclusions from data.

How to Interpret Statistical Data

Understanding and making sense of data is essential for drawing valid conclusions. The ability to interpret numbers, graphs, and charts allows you to extract meaningful insights from raw information. Whether you’re working with a simple data set or complex results, knowing how to analyze and interpret findings is key to making informed decisions.

Here are some steps to effectively interpret data:

Understand the Context – Before diving into the data, consider the source and purpose of the study. Knowing the context helps you interpret the results more accurately.
Examine Descriptive Statistics – Look at measures like the mean, median, and standard deviation to get an overall sense of the data’s central tendency and variability.
Analyze Visualizations – Graphs such as histograms, bar charts, and scatter plots can provide a quick and clear picture of data trends and relationships between variables.
Identify Outliers – Pay attention to data points that deviate significantly from the rest. These outliers can indicate errors or important trends that require further investigation.
Draw Conclusions from Results – Interpret the findings in relation to the hypothesis or research question. Make sure to evaluate whether the results are statistically significant and whether they support the initial claim.

By following these steps, you can ensure that you’re interpreting data in a thoughtful and methodical manner, leading to more accurate insights and conclusions.

Key Formulas to Remember for Exams

Mastering important equations and concepts is critical for solving problems efficiently during assessments. Familiarity with key formulas allows you to quickly apply the correct techniques and reduce the risk of making errors. Below are several essential formulas that you should remember and understand in depth.

Basic Formulas

Mean (Average): μ = (ΣX) / N – The sum of all data points divided by the total number of data points.
Variance: σ² = Σ(X - μ)² / N – The average of the squared differences from the mean.
Standard Deviation: σ = √(σ²) – The square root of the variance, which provides a measure of data spread.
Probability of an Event: P(A) = (Number of favorable outcomes) / (Total number of possible outcomes) – The likelihood of an event occurring.

Advanced Formulas

Normal Distribution: Z = (X - μ) / σ – A formula used to find the Z-score, representing the number of standard deviations a data point is from the mean.
Binomial Distribution: P(X = k) = (n choose k) * p^k * (1-p)^(n-k) – The probability of k successes in n trials for a binomial distribution.
Confidence Interval: CI = μ ± Z * (σ / √n) – A range of values used to estimate the population mean based on sample data.

Being well-versed in these formulas and understanding when to apply each one will help you perform confidently in any problem-solving scenario during assessments.

Breaking Down Regression Analysis Questions

Regression analysis is a powerful tool for understanding relationships between variables. When faced with these types of questions, it’s important to carefully identify the variables involved and select the correct approach to quantify their relationship. By breaking down each part of the problem, you can accurately interpret the results and make informed predictions.

Understanding the Variables

The first step in solving a regression problem is to clearly identify the dependent and independent variables. The dependent variable is what you are trying to predict or explain, while the independent variables are those that influence or predict the dependent variable. Understanding these relationships is key to selecting the correct regression model.

Interpreting the Results

Coefficient Interpretation: The coefficients represent the change in the dependent variable for each one-unit change in an independent variable. Pay attention to whether these coefficients are positive or negative, as they indicate the direction of the relationship.
R-Squared: This value indicates how well the independent variables explain the variation in the dependent variable. A higher R-squared value suggests a better fit.
p-Values: These values help determine the statistical significance of each independent variable. A p-value less than 0.05 typically indicates that the variable significantly contributes to the model.

By following these steps and carefully interpreting the coefficients, R-squared, and p-values, you will be able to accurately solve regression analysis questions and understand the relationships between variables.

Tips for Time Management on the Exam

Effective time management during an assessment is essential to ensure you complete all tasks while maintaining accuracy. Planning your time wisely allows you to allocate enough attention to each section and avoid rushing through difficult problems. Below are some practical strategies to help you manage your time efficiently.

Start by familiarizing yourself with the structure of the test and how much time you have for each section. Prioritize questions based on their difficulty and point value. By doing this, you can allocate enough time to tackle the more complex problems while ensuring you answer the simpler ones first.

Time Allocation Strategy	Suggested Action
First 10 Minutes	Skim through the entire test to understand the structure and mark easy questions to answer first.
Next 60 Minutes	Work on questions you find easy and fast to complete, aiming to build confidence and ensure maximum points.
Last 30 Minutes	Focus on more complex problems. Break them into smaller steps and double-check your answers.

By following a clear time management plan, you can maximize your performance and reduce stress, ensuring you make the most of your assessment time.

Understanding Descriptive Statistics Questions

Questions related to summarizing and analyzing data often test your ability to interpret and describe key characteristics of a data set. These types of questions typically focus on measures of central tendency, variability, and distribution, all of which help provide a comprehensive overview of the data. A solid understanding of these concepts will allow you to quickly extract meaningful insights from raw numbers.

Measures of Central Tendency

In many problems, you will be asked to calculate and interpret the mean, median, or mode of a data set. The mean provides the average value, while the median represents the middle value when the data is ordered, and the mode refers to the most frequently occurring value. Each of these measures gives you a different perspective on the central location of the data.

Measures of Spread and Variability

Another critical aspect of summarizing data is understanding how it varies. The range, variance, and standard deviation are common measures used to describe the spread or dispersion within a data set. These values help you understand how much the data points differ from the mean and how consistent or diverse the data is.

When answering questions on this topic, make sure to carefully analyze the given data, calculate the necessary measures, and interpret the results in the context of the problem. Being familiar with these concepts will help you answer descriptive questions accurately and efficiently.

How to Tackle Graph and Chart Problems

Interpreting visual data representations such as graphs and charts is a key skill for many assessments. These types of questions often require you to extract information from a visual format and convert it into meaningful insights. By breaking down the components of each graph or chart, you can easily identify trends, relationships, and key data points.

When approaching these questions, it’s important to first understand the structure of the visual. Focus on the axes, labels, scales, and units to ensure you’re interpreting the data correctly. Pay attention to any titles or legends that provide context for the information being presented.

Steps to Solve Graph and Chart Problems

Examine the Title: Begin by reading the title to understand what the graph or chart represents. This gives you the context of the data.
Analyze the Axes: Check the labels on both axes, paying attention to the units and scales. This will help you determine the variables being compared.
Identify Trends or Patterns: Look for trends, such as increasing or decreasing values, and any noticeable patterns. This could provide insight into the relationships between variables.
Check for Outliers: Be sure to identify any data points that fall far outside the general trend, as these may be important or could indicate errors in the data.

Common Graph Types

Bar Graphs: Useful for comparing discrete categories. Pay attention to the height of each bar to determine relative values.
Line Graphs: Ideal for showing changes over time. Look for upward or downward trends and fluctuations.
Pie Charts: Show proportions or percentages of a whole. Ensure that the segments are correctly labeled to interpret the data accurately.
Scatter Plots: Display relationships between two continuous variables. Identify clusters or patterns of data points to understand correlations.

By following these steps, you can approach graph and chart-related questions with confidence and extract the correct information for your answers.

Dealing with Variance and Standard Deviation

Variance and standard deviation are essential tools for understanding how spread out or dispersed a set of data is. These concepts help to quantify the degree of variation in a dataset, indicating how much individual data points differ from the average. Mastering these measures is crucial for accurately interpreting the reliability and consistency of data.

Variance is calculated by finding the average squared difference between each data point and the mean. This gives you a sense of how far the data points are from the mean, though it’s expressed in squared units, which can sometimes be less intuitive. On the other hand, standard deviation is simply the square root of the variance, providing a more interpretable measure in the original units of the data.

To calculate variance and standard deviation, follow these basic steps:

Find the mean: Add all the data points together and divide by the total number of points.
Calculate squared differences: For each data point, subtract the mean and square the result.
Average the squared differences: Sum all the squared differences and divide by the number of data points (for population variance) or by the number of data points minus one (for sample variance).
Take the square root: For standard deviation, take the square root of the variance to return to the original units of measurement.

By following these steps, you can effectively calculate and interpret both variance and standard deviation, gaining valuable insights into the spread and consistency of any dataset. Understanding these measures is fundamental for analyzing the reliability of any data-driven conclusion or prediction.

Approaching Sampling and Distribution Problems

When tackling problems related to sample selection and data distribution, the goal is to understand how data points are chosen and how they behave across different samples. The primary objective is to gain insights into the overall population by analyzing smaller subsets, or samples, and understanding how variations in the data can influence predictions and decisions. These problems often require knowledge of different sampling techniques and the characteristics of distributions to draw accurate conclusions.

For most of these problems, it’s essential to recognize that different types of samples can yield different results. Whether you are working with a simple random sample, stratified sample, or cluster sample, the methodology impacts the outcome and interpretation. In addition, understanding key concepts such as the central limit theorem and the normal distribution plays a crucial role in handling these types of problems effectively.

Key Concepts in Sampling

Random Sampling: Ensures that each member of the population has an equal chance of being selected, helping to avoid bias in data collection.
Stratified Sampling: Divides the population into distinct groups and then randomly samples from each group to ensure all segments are represented.
Cluster Sampling: Involves dividing the population into groups or clusters, then randomly selecting entire clusters for study.
Systematic Sampling: Involves selecting every nth data point from a list, which can help simplify large datasets.

Understanding Distributions

Distributions describe how values in a dataset are spread out. When working with distribution-related problems, it’s important to understand the shape, center, and spread of the data. A common assumption in many problems is that data follows a normal distribution, but this is not always the case. Some data may exhibit skewness, while others may be bimodal or uniform.

Distribution Type	Description	Example
Normal Distribution	Symmetrical, bell-shaped curve with most values near the mean.	Heights of individuals in a population
Skewed Distribution	Asymmetrical distribution with a longer tail on one side.	Income distribution in a population
Bimodal Distribution	Two peaks or modes in the dataset.	Test scores with two distinct groups of students
Uniform Distribution	All values occur with equal frequency.	Rolling a fair die

Mastering sampling techniques and understanding the characteristics of distributions are essential for solving related problems. The better you can identify the type of distribution and the appropriate sampling method, the more accurate and meaningful your analysis will be.

Common Mistakes to Avoid in Exams

When preparing for and taking any kind of assessment, it’s easy to fall into common traps that can negatively impact performance. Often, the errors made are not due to lack of knowledge but from poor habits or mismanagement of time and resources. Being aware of these mistakes can help ensure a smoother and more successful experience during the assessment.

One key to avoiding errors is to thoroughly understand the instructions and not rush through questions. Many mistakes happen when students misinterpret the requirements or overlook important details. In addition, a lack of proper preparation and stress management can also lead to unnecessary confusion and mistakes.

Common Pitfalls to Watch Out For

Misunderstanding Instructions: Always take time to carefully read the questions. Misinterpreting what is being asked can lead to answering incorrectly, even if you know the material.
Rushing Through the Test: Speed is important, but hasty decisions often result in careless errors. Avoid rushing through problems without double-checking your work.
Neglecting Time Management: Failing to allocate enough time for each section can lead to incomplete answers. It’s essential to pace yourself throughout the assessment.
Overlooking Small Details: Pay attention to things like decimal points, signs, or units, which are often overlooked but can lead to significant errors.
Leaving Questions Unanswered: If unsure, try to make an educated guess or at least leave a note to return to later instead of skipping it entirely.

How to Avoid These Mistakes

Practice Time Management: Use mock tests to simulate the real test environment. This will help you get used to the time constraints and reduce stress.
Review Your Work: After completing a question, take a moment to review your response. Double-check for calculation errors or missing elements.
Stay Calm and Focused: Anxiety can cloud judgment and lead to errors. Stay calm, focus on one question at a time, and trust in your preparation.
Read Carefully: Avoid skimming through questions. Pay attention to every word, especially the parts that outline specific requirements for each task.

By staying mindful of these common mistakes and taking proactive steps to avoid them, you can improve your performance and reduce the likelihood of errors during any type of assessment.

How to Review Before the Final Exam

Preparing for a major assessment requires more than just cramming information at the last minute. Effective revision involves reviewing key concepts, practicing problems, and understanding the material in-depth. To maximize your performance, it’s essential to approach the review process strategically and methodically.

One of the most important steps in the review process is identifying the areas that need the most attention. By focusing on challenging topics or areas where you’ve struggled in the past, you can ensure that you are well-prepared to tackle those questions. Additionally, practicing problems under time constraints can help improve both speed and accuracy.

Effective Review Techniques

Create a Study Schedule: Break down your study time into manageable blocks and assign specific topics to each session. This will help you stay organized and focused.
Review Key Concepts: Go over your notes, textbooks, and any other materials to reinforce the core principles. Make sure you understand the “why” behind each concept, not just the “how.”
Practice with Past Papers: Working through past assessments will help familiarize you with the format and types of questions that may appear. It also provides valuable practice in managing time.
Teach Someone Else: Explaining concepts to a friend or classmate can reinforce your understanding and highlight any areas where you may need further clarification.
Take Breaks: Don’t overload yourself with continuous study. Take short breaks to recharge and avoid burnout, allowing your brain to absorb and retain information more effectively.

How to Stay Focused During Review

Avoid Multitasking: Focus on one topic at a time rather than jumping between subjects. Multitasking can reduce efficiency and retention.
Set Realistic Goals: Set achievable targets for each study session. This will help you stay motivated and make steady progress.
Stay Positive: Confidence plays a big role in exam preparation. Maintain a positive mindset, and trust that your hard work will pay off.

By employing these strategies, you can feel more confident and prepared going into the assessment, ensuring that you’ve covered all necessary material and are ready to perform at your best.