
Achieving success in advanced mathematics courses requires more than just understanding the core concepts; it demands the ability to apply those concepts in a timed and structured environment. A well-planned approach to preparation can significantly improve performance and build confidence. By familiarizing yourself with the types of questions and challenges you’ll encounter, you can sharpen both your problem-solving abilities and your test-taking strategies.
One of the most effective ways to prepare for these assessments is by engaging in mock evaluations that simulate the real test conditions. These assessments are invaluable tools that allow students to track their progress, identify weak areas, and refine their approach. Emphasizing timing, accuracy, and strategic problem-solving is crucial for maximizing your score and achieving optimal results.
In this guide, we will explore essential methods for mastering the challenges of advanced mathematics tests, focusing on improving both theoretical knowledge and practical application. By taking a systematic approach to practice, you will gain the confidence needed to perform at your best when it matters most.
Advanced Mathematics Assessment Preparation
Preparing for a rigorous mathematics evaluation requires careful planning and strategic practice. Understanding the structure of the test, along with the types of questions typically presented, is crucial for achieving a high score. Simulating real testing conditions through mock assessments helps students improve both their knowledge and time management skills, ultimately leading to greater success on the actual test day.
Key Areas to Focus On
Familiarize yourself with the core topics that will be covered, including functions, rates of change, integration, and limits. These concepts are essential to tackling the most challenging questions. In-depth practice with problems that focus on these areas will help you refine your skills and ensure you’re ready for any problem type that could appear in the test.
Improving Time Management and Strategy
When simulating test conditions, focus on managing your time efficiently. Each section of the assessment has a specific time limit, and learning to pace yourself ensures you can complete all tasks without rushing. Developing strategies for handling more difficult questions–such as skipping and returning to harder problems–can prevent you from running out of time and missing easier questions.
Overview of the AP Calculus AB Exam

The assessment for advanced mathematics aims to evaluate students’ understanding of fundamental concepts and their ability to apply these concepts in real-world scenarios. It is structured to test both theoretical knowledge and practical problem-solving skills, providing a comprehensive measure of a student’s readiness for further studies in mathematics and related fields.
Test Structure and Format
The evaluation consists of two main parts: multiple-choice questions and free-response sections. Each section focuses on different skill sets, with the multiple-choice segment assessing quick thinking and the free-response portion emphasizing detailed, step-by-step problem-solving. Both sections are designed to test students’ knowledge across a range of topics, from basic functions to more complex integrals and derivatives.
Key Topics Covered
The core content areas of the assessment include the study of limits, derivatives, integrals, and the fundamental theorem of calculus. Understanding these core concepts, along with their applications, is critical for success. The test also requires familiarity with problem-solving techniques and the ability to apply them under time constraints, making preparation essential for achieving a high score.
Key Concepts Tested in AP Calculus AB
The assessment evaluates a broad range of mathematical concepts that are fundamental to higher-level studies in mathematics and science. Mastery of these principles is crucial for solving complex problems and demonstrating a deep understanding of how mathematical theories apply to real-world situations. The test covers a variety of topics, from foundational theories to advanced techniques used in problem-solving.
Limits and Continuity
One of the primary concepts assessed is the understanding of limits and continuity. These foundational ideas are essential for grasping how functions behave as they approach specific values or points. Questions in this area often focus on calculating limits, determining the continuity of functions, and understanding the behavior of functions near points of interest.
Derivatives and Their Applications
The assessment also tests students’ knowledge of derivatives and their use in solving real-world problems. This includes understanding how rates of change are represented mathematically, applying derivative rules, and interpreting the meaning of derivatives in various contexts. Problems in this section often involve optimization, motion analysis, and determining the behavior of functions using derivatives.
Effective Study Strategies for Calculus AB
Success in advanced mathematics requires more than just reviewing notes; it demands a structured approach to learning and continuous practice. By focusing on understanding core concepts, applying them through problem-solving, and refining your skills regularly, you can significantly improve your performance. Strategic preparation allows you to tackle even the most challenging problems with confidence and efficiency.
One of the most effective study strategies is to break down complex topics into smaller, manageable sections. This method helps build a strong foundation and ensures that each concept is fully understood before moving on to more advanced material. Regular self-assessment through mock problems and tests is also essential for tracking progress and identifying areas for improvement.
In addition, collaborating with peers or seeking help from instructors can offer new perspectives on difficult problems. Engaging in discussions and explaining concepts to others enhances retention and deepens understanding. Consistency is key–devoting time each day to studying ensures that the material becomes second nature and reduces stress as the assessment approaches.
How to Use Practice Exams for Success

Simulating real test conditions through mock assessments is one of the most effective ways to prepare for a major evaluation. These exercises allow students to gauge their readiness, familiarize themselves with the test format, and identify areas that need improvement. By using these tools strategically, students can enhance their time management skills, reduce test anxiety, and improve overall performance on the actual day.
Maximizing the Value of Practice Sessions
To get the most out of mock tests, it’s important to approach them with a focused mindset. Begin by setting a time limit that mirrors the actual test. This will help you develop an accurate sense of pacing and prevent you from spending too much time on any one problem. After completing the assessment, take time to thoroughly review your answers, paying special attention to the mistakes you made and understanding why they occurred.
Tracking Your Progress
Tracking your performance over multiple practice sessions will give you valuable insight into your strengths and weaknesses. By analyzing your results, you can pinpoint recurring problem areas and focus your studies accordingly. This ongoing evaluation also helps you build confidence, as you can see your improvements over time.
| Test Date | Score | Areas of Focus |
|---|---|---|
| March 15 | 75% | Limits, Derivatives |
| March 22 | 80% | Optimization, Integrals |
| March 29 | 85% | Application Problems, Rates of Change |
Time Management Tips for AP Calculus AB
Effectively managing your time during a rigorous assessment is essential for maximizing your performance. With a limited amount of time to answer a wide range of questions, having a strategy in place can help you remain calm, focused, and ensure that you complete all sections within the allotted time. A well-thought-out approach to time management can also reduce stress and improve the quality of your responses.
One key strategy is to quickly assess the test as soon as you begin. Skim through all the questions to get an idea of which ones you can answer confidently and which might require more time. Begin with the problems you find easiest, and save the more challenging ones for later. This allows you to accumulate points early on, boosting your confidence as you move through the test.
Another essential tactic is to avoid spending too much time on any single question. If you get stuck, move on and return to the difficult questions later. Setting time limits for each section or problem helps ensure that you don’t linger too long on any one area, giving you ample time to address all questions. Practice pacing yourself during mock tests so that you develop an instinct for how much time to allocate for each task.
Understanding AP Calculus AB Scoring
The scoring system for an advanced mathematics assessment is designed to reflect not only the correctness of answers but also the quality and method of problem-solving. Understanding how your performance is evaluated can help you approach the test with greater awareness and strategize accordingly. The test is typically divided into multiple-choice questions and free-response sections, each contributing to your overall score in different ways.
Each section of the assessment is weighted differently, and the points you earn are combined to form your final score. The free-response section, which tests deeper problem-solving abilities, often carries more weight than the multiple-choice section. However, each part plays a crucial role in determining your overall performance, so balancing your focus between both areas is essential for achieving a strong score.
| Section | Weight | Points |
|---|---|---|
| Multiple Choice | 50% | 45 points |
| Free Response | 50% | 45 points |
| Overall Score | 100% | 90 points |
Your score on the free-response section is based not only on the correctness of your answers but also on the clarity and completeness of your explanations. It’s important to show all of your work and document your reasoning step-by-step. The multiple-choice section, while focusing more on the speed and accuracy of your answers, still rewards students who demonstrate a strong understanding of concepts.
Common Mistakes on the AP Calculus Exam
Even the most prepared students can make errors during a rigorous assessment, often due to time pressure, misinterpretation of questions, or overlooking essential details. Identifying common mistakes and being aware of them can help you avoid falling into these traps during the test. Being mindful of these pitfalls will ensure a more accurate and efficient performance on test day.
Below are some of the most frequent errors students make during this type of evaluation:
- Misunderstanding the Question: Sometimes, students focus too heavily on the math without fully interpreting the problem. It’s crucial to carefully read the question and ensure you understand exactly what is being asked before diving into calculations.
- Skipping Steps in Solutions: Rushing through the problems can lead to missed steps in the solution process, especially in multi-step problems. Always show all work, as this not only helps in grading but ensures you don’t skip critical elements of the problem.
- Not Using Units Appropriately: In problems involving real-world applications, forgetting to include or convert units is a common mistake. Pay attention to units of measurement and ensure they are consistent throughout your calculations.
- Overlooking Domain Restrictions: When working with functions, especially in derivatives and integrals, be sure to consider domain restrictions. Ignoring these can lead to incorrect answers or interpretations.
- Not Checking for Reasonableness: After solving a problem, always take a moment to check if your solution makes sense in the context of the question. This step can help you identify simple calculation errors or logical mistakes.
Being mindful of these common mistakes and consistently practicing effective strategies can significantly improve your performance and help you avoid pitfalls during the test.
Top Resources for AP Calculus AB Preparation
To succeed in advanced mathematics assessments, it’s essential to utilize a variety of study tools that help reinforce key concepts and test-taking strategies. From textbooks and online platforms to study guides and instructional videos, there are numerous resources available to enhance your understanding and boost your performance. The right mix of materials can provide a comprehensive approach to mastering the material and building confidence.
Recommended Textbooks and Study Guides
Textbooks offer in-depth explanations of key concepts, while study guides distill essential information for quick revision. Both can be helpful in preparing for the evaluation, but it’s important to choose the ones that best match your learning style. Some resources focus on practice problems, while others explain the theory behind the concepts.
Online Platforms and Tools
In addition to physical study materials, online platforms provide interactive learning experiences. These platforms often include practice questions, video tutorials, and virtual study groups. They allow you to review content at your own pace and seek assistance when needed.
| Resource | Type | Key Features |
|---|---|---|
| AP Classroom | Online Platform | Official AP resources with interactive questions and videos |
| Khan Academy | Online Platform | Free video lessons and exercises for concept reinforcement |
| 5 Steps to a 5 | Study Guide | Comprehensive review and practice tests with expert tips |
| CliffNotes AP Study Guide | Study Guide | Concise summaries and practice problems for quick revision |
By using a combination of these resources, you can create a tailored study plan that suits your needs and helps you prepare thoroughly for the test.
How to Approach Multiple Choice Questions
Multiple-choice questions are designed to test your understanding of concepts quickly and efficiently. The key to tackling these types of questions is to read carefully, eliminate incorrect answers, and make sure that your final choice is well-supported by your knowledge. Proper strategy is essential to ensure that you maximize your score without wasting time on tricky or ambiguous questions.
Effective Strategies for Answering Multiple Choice Questions
Here are some effective strategies for handling multiple-choice questions with confidence:
- Read Each Question Carefully: Before considering the options, make sure you fully understand what the question is asking. Look for keywords that specify the operation or concept being tested.
- Eliminate Clearly Incorrect Answers: If you’re unsure about the answer, start by eliminating obviously wrong options. This will increase your chances of selecting the correct one from the remaining choices.
- Consider All Answer Choices: Even if you think you know the correct answer right away, be sure to review all the options. Sometimes, a seemingly obvious answer might not be the best choice after all.
- Look for Traps and Common Mistakes: Be aware of common traps, such as answers that seem similar to the correct one but contain small errors. These can be designed to test attention to detail.
- Don’t Overthink: Once you’ve chosen an answer, don’t second-guess yourself too much. If you’ve followed your strategy and feel confident in your decision, trust your instincts and move on.
Time Management in Multiple Choice Questions
Time management is crucial when answering multiple-choice questions. If you find a question difficult or time-consuming, move on and return to it later. This allows you to avoid getting stuck and ensures that you have time to attempt all questions.
- Set Time Limits: Try to spend no more than 1–2 minutes on each question. If you can’t answer it within that time, make an educated guess and move on.
- Use Process of Elimination: Even if you’re unsure, eliminating one or two wrong answers will often leave you with a better chance of guessing correctly.
- Review Your Answers: If time permits, go back and check your answers, especially for questions you were uncertain about.
By following these strategies, you can increase your accuracy and confidence when tackling multiple-choice questions. This approach ensures that you’re not only answering questions correctly but also managing your time effectively throughout the test.
Mastering Free Response Questions in Calculus
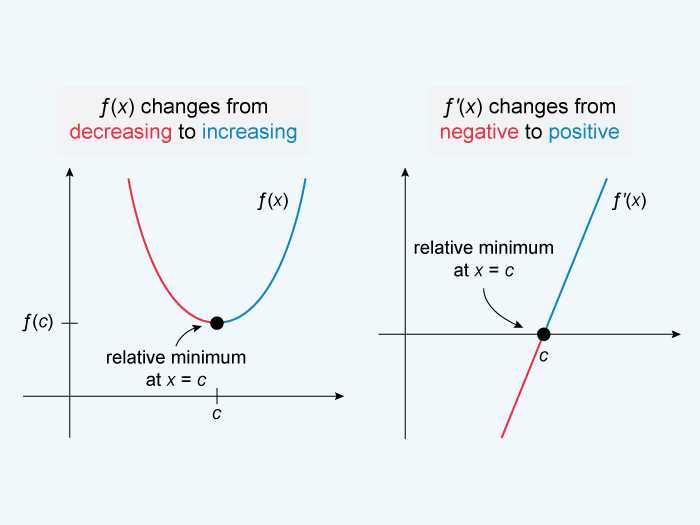
Free response questions require more than just selecting the correct answer from a list–they test your ability to demonstrate your understanding and solve problems step by step. Success in these types of questions lies in presenting your reasoning clearly, applying mathematical principles effectively, and showcasing your problem-solving skills. By developing a structured approach, you can ensure that you fully address each part of the problem and maximize your score.
Key Strategies for Free Response Questions
Here are some strategies to help you excel in free response sections:
- Understand the Problem: Read each question carefully and identify the key elements. Look for the given information, what is being asked, and the mathematical tools you’ll need to use.
- Break the Problem Into Steps: Complex problems can often be tackled more easily by dividing them into smaller, manageable parts. Write out each step clearly, showing your work and reasoning along the way.
- Show All Work: Unlike multiple-choice questions, free response problems often reward you for demonstrating the process, even if you don’t arrive at the final answer. Be sure to show every step of your work, as partial credit can make a significant difference.
- Use Proper Notation: Neatness and clarity matter. Ensure that your mathematical symbols, variables, and operations are correctly written and easy to follow. Proper notation helps the grader understand your thought process.
Managing Time and Accuracy in Free Response
Time management is just as important in free response questions as it is in multiple-choice sections. You need to allocate time wisely to avoid spending too long on one problem.
- Set a Time Limit per Question: Before starting, decide how much time you’ll allocate to each problem based on its complexity. Stick to this limit to ensure you have time for all questions.
- Prioritize Questions: If you come across a particularly difficult question, skip it temporarily and return to it later. This ensures that you don’t spend too much time on a single problem.
- Review Your Work: If time permits, review your answers before submitting. Double-check your calculations and make sure your reasoning is clear.
By focusing on clear problem-solving steps, accurate notation, and effective time management, you’ll increase your chances of succeeding in free response questions. This approach will allow you to showcase your full understanding and tackle even the most challenging problems with confidence.
Calculus AB Practice Tests: What to Expect
When preparing for a high-level mathematics assessment, practice tests play a crucial role in gauging your understanding and readiness. These tests simulate the actual assessment conditions and help you familiarize yourself with the format, question types, and timing. Knowing what to expect can significantly reduce anxiety and enhance your performance, giving you the confidence to tackle each section effectively.
Structure and Content of the Test
The assessment is typically divided into two main sections: multiple-choice and free response. Here’s a breakdown of each component:
- Multiple-Choice Section: This section tests your ability to recognize and apply mathematical concepts. It covers a wide range of topics, requiring you to select the correct answer from several options. Speed and accuracy are essential in this section.
- Free Response Section: These questions require a more detailed approach, as you’ll need to show your work step by step. This section evaluates your problem-solving abilities, logical reasoning, and clarity in presenting mathematical arguments.
Types of Questions and Key Concepts
While the test format may vary slightly, you can generally expect questions on the following core topics:
- Functions and Graphs: Understanding different types of functions and their graphs is crucial. You may be asked to analyze or interpret the behavior of functions at certain points or over intervals.
- Derivatives: You’ll need to apply derivative rules to solve problems involving rates of change, slopes of tangents, and optimization.
- Integrals: Integration is another fundamental topic, with questions related to area, volume, and solving real-world problems through integration techniques.
- Limits and Continuity: Expect questions that test your understanding of limits, continuity, and the concept of approaching values in various functions.
Preparing for these tests involves both mastering theoretical concepts and developing practical problem-solving skills. By familiarizing yourself with the structure and types of questions, you can approach each practice test with the right mindset and improve your performance in the actual assessment.
Reviewing Key Formulas for AP Calculus AB
Mastering essential mathematical formulas is crucial for solving complex problems efficiently and accurately. Whether you are tackling derivatives, integrals, or optimization problems, knowing the right formulas allows you to approach each challenge with confidence. This section reviews the most important formulas that you should have at your fingertips for the test, ensuring you’re well-prepared for a variety of questions.
Essential Derivative and Integration Formulas
The following formulas are fundamental for solving differentiation and integration problems:
- Power Rule for Derivatives: d/dx (x^n) = n * x^(n-1)
- Product Rule for Derivatives: d/dx (u * v) = u’ * v + u * v’
- Quotient Rule for Derivatives: d/dx (u / v) = (v * u’ – u * v’) / v^2
- Chain Rule for Derivatives: d/dx (f(g(x))) = f'(g(x)) * g'(x)
- Fundamental Theorem of Calculus: ∫ from a to b f(x) dx = F(b) – F(a)
- Integration by Parts: ∫ u dv = u * v – ∫ v du
Limits, Continuity, and Trigonometric Identities
In addition to differentiation and integration, understanding limits and continuity is essential for handling more advanced topics. Here are some key formulas and concepts related to limits and trigonometric functions:
- Limit of a Rational Function: lim (x -> a) (f(x)/g(x)) = f(a) / g(a), if g(a) ≠ 0
- L’Hopital’s Rule: lim (x -> a) f(x) / g(x) = lim (x -> a) f'(x) / g'(x) (if the limit results in 0/0 or ∞/∞)
- Trigonometric Limits: lim (x -> 0) sin(x)/x = 1
- Sum and Difference of Sines: sin(A ± B) = sin(A) cos(B) ± cos(A) sin(B)
- Double Angle Identity: sin(2θ) = 2sin(θ)cos(θ)
By regularly reviewing and practicing these formulas, you will be better prepared to tackle problems quickly and accurately. Having a solid grasp of these core equations is essential for success in higher-level mathematics assessments.
Using AP Calculus AB Study Guides Effectively
Study guides are essential tools that help you consolidate your understanding of key concepts and prepare effectively for assessments. When used properly, these guides can streamline your revision process, focusing your efforts on the most important material and improving your problem-solving skills. In this section, we will explore how to make the most of these resources to enhance your study sessions.
The first step in using study guides effectively is to identify the areas where you need the most improvement. Start by reviewing the guide’s table of contents or overview to get an understanding of the topics covered. Once you know the areas that need more focus, allocate more time to those sections. This strategy ensures that your study efforts are aligned with your current understanding and the topics most likely to appear in assessments.
Next, it’s important to work through the problems and examples provided in the guide. These exercises are designed to reinforce the concepts you’ve learned and provide hands-on practice. Take your time to solve each problem and review the solutions thoroughly. Pay special attention to any mistakes you make and ensure you understand the correct approach for similar problems in the future.
Finally, supplement your study guide with additional resources such as online tutorials or practice tests to reinforce what you’ve learned. This multi-faceted approach will ensure that you’re well-rounded in your preparation, allowing you to approach the material from different angles and solidify your understanding even further.
How to Deal with Exam Stress
Feeling anxious before a major test is a common experience, but managing that stress effectively can significantly improve your performance. The key is to stay calm, stay organized, and maintain a healthy balance between studying and relaxation. In this section, we’ll discuss strategies to help you manage stress and stay focused throughout your preparation and on the test day itself.
1. Develop a Study Plan
One of the most effective ways to reduce anxiety is by creating a structured study schedule. Break your study time into manageable sessions, focusing on specific topics during each one. Avoid cramming the night before by spreading your preparation over several days or weeks. A clear plan gives you a sense of control and helps you avoid feeling overwhelmed.
2. Practice Mindfulness and Relaxation Techniques
Incorporating relaxation techniques into your routine can be a powerful tool in managing stress. Simple practices such as deep breathing exercises, meditation, or light physical activities like stretching can help calm your mind. Taking short breaks during your study sessions can also help refresh your mental state, ensuring that you stay sharp and focused.
Remember: Stress is a normal part of the process, but with the right strategies, you can manage it effectively. Stay positive, trust your preparation, and approach the test with confidence.
Benefits of Taking AP Calculus AB Practice Exams
Completing mock tests before a major assessment offers a variety of advantages that can enhance both your confidence and your understanding of the material. These simulated tests mirror the real testing environment and can help you identify areas that need improvement while also familiarizing you with the structure and timing of the actual assessment.
1. Identifying Strengths and Weaknesses
Taking a mock test allows you to pinpoint areas where you excel and areas that may require more attention. By recognizing your weaknesses early on, you can allocate your study time more efficiently, focusing on topics that need more practice. This targeted approach maximizes the effectiveness of your study sessions and helps you make progress faster.
2. Building Time Management Skills
One of the biggest challenges during any timed test is managing time effectively. Simulated assessments give you the opportunity to practice pacing yourself, ensuring that you can complete all questions within the given time frame. Learning to manage time efficiently will help reduce anxiety and increase your chances of performing well under real test conditions.
In conclusion: Taking mock tests is a valuable tool for exam preparation. It not only enhances your understanding of the subject matter but also helps you build essential test-taking skills, such as time management and strategic thinking.
Improving Your Speed and Accuracy

Mastering both speed and accuracy is essential for success in any timed assessment. By developing strategies to enhance these two aspects, you can perform better under pressure, ensuring that you complete all questions with precision and within the allocated time. Focusing on improving these skills not only helps you answer more questions correctly but also boosts your confidence during the test.
One key method for improving speed is regular timed practice. By replicating test conditions during your study sessions, you can learn to work efficiently without sacrificing the quality of your answers. Over time, this will help you get faster without losing accuracy.
Another effective strategy is focusing on minimizing mistakes. Avoid rushing through questions; instead, develop a habit of double-checking your work when possible. Using shortcuts and memorizing key concepts can also save time, but make sure to balance speed with thoroughness to avoid errors.
In conclusion: Combining speed with accuracy requires consistent practice and the use of efficient strategies. Focus on improving both aspects gradually, and you’ll find yourself answering questions faster and more accurately when it matters most.
What to Do the Week Before the Exam
The final week before a major assessment is crucial for consolidation and fine-tuning your skills. During this period, it’s important to focus on reviewing key concepts, practicing targeted problems, and ensuring you’re mentally prepared for the test. The goal is to build confidence and avoid unnecessary stress, while still ensuring you’re ready to tackle any challenge on the test day.
One of the most effective approaches during the last week is to focus on weaknesses. Identify areas where you have the most difficulty, whether it’s certain types of problems or specific concepts, and dedicate time to working through them. Practice problems and review solutions to ensure you fully understand the steps involved.
Additionally, review the structure of the test. Familiarize yourself with the types of questions you may encounter, and get comfortable with the format. While you shouldn’t cram new information at this point, solidifying what you’ve already learned will help reduce anxiety and increase performance on test day.
| Day | Focus Area |
|---|---|
| Monday | Review all key concepts and formulas |
| Tuesday | Work on timed practice sets |
| Wednesday | Identify and target weak areas for improvement |
| Thursday | Review notes and practice problems |
| Friday | Take a full-length mock test |
| Saturday | Review test performance and refine strategies |
| Sunday | Relax and review light materials |
Key Takeaways: The week before the test should be a mix of review, practice, and relaxation. Focus on reinforcing what you already know, tackle your weaker areas, and maintain a calm mindset as the test approaches.