As you approach the end of the academic term, it’s crucial to consolidate your understanding of key mathematical concepts. This section aims to guide you through the essential topics that will be tested, helping you refresh and reinforce your knowledge. Whether you are reviewing fundamental equations or exploring more complex problems, a thorough preparation plan can significantly boost your confidence.
Focus on mastering the core principles that frequently appear in tests. From solving equations to working with different types of functions, these core topics are the building blocks of more advanced material. Focusing on understanding the process rather than just memorizing formulas will give you a deeper grasp of how to approach problems effectively.
By reviewing key areas and practicing a variety of questions, you’ll ensure that you are well-equipped for any challenges that may arise. This preparation will not only help you succeed in the test but also strengthen your overall mathematical skills for future learning.
Mathematics Test Preparation Plan
A well-structured approach to preparing for your upcoming assessment is key to success. This plan will guide you through a focused study strategy that targets the most important topics you’ll encounter. Organizing your time and efforts efficiently will allow you to master the concepts and perform confidently when it’s time to test your knowledge.
Start by identifying the primary areas of focus, such as solving equations, graphing linear relationships, and working with inequalities. These are foundational topics that frequently appear in assessments and are crucial to understanding more complex material. Allocating time to each subject based on its difficulty and importance ensures you cover all the essential points.
Next, incorporate a variety of practice problems to reinforce what you’ve learned. Hands-on practice is essential for mastering any mathematical concept. As you solve problems, take note of any areas where you feel less confident and spend extra time on those. Consistent practice will help solidify your skills and improve your problem-solving speed.
Finally, as you approach the final days before the assessment, focus on reviewing your mistakes and clarifying any lingering uncertainties. A targeted review of challenging areas will boost your readiness. Stay positive and focused, and you’ll be prepared to tackle the test with confidence.
Key Topics to Focus On

When preparing for an upcoming assessment in mathematics, it’s essential to concentrate on the core concepts that are most likely to appear. Understanding these foundational topics will help you build confidence and perform well. Focusing your efforts on the areas that require the most attention ensures a comprehensive understanding of the material.
The following areas should be prioritized as they are commonly tested and fundamental for mastering more complex topics:
- Solving linear equations and inequalities
- Understanding and applying properties of exponents
- Graphing and interpreting linear relationships
- Factoring polynomials and quadratic expressions
- Working with systems of equations
- Understanding functions and their behaviors
By thoroughly revisiting these topics and ensuring you can solve related problems with ease, you’ll be better prepared to handle any questions related to them. A strong grasp of these key areas is crucial for success and will serve as the foundation for more advanced studies.
Understanding Mathematical Expressions
Mastering mathematical expressions is a crucial step in developing a strong foundation in mathematics. These expressions form the basis of more complex problems and are frequently encountered in various forms. Being able to interpret and manipulate them accurately is essential for solving a wide range of equations and inequalities.
Components of an Expression
An expression consists of variables, constants, and mathematical operations. The variables represent unknown values, while constants are fixed numbers. Operations such as addition, subtraction, multiplication, and division link these components together to form a complete expression. Understanding the role of each element in an expression is key to simplifying or solving it.
Simplifying and Evaluating Expressions

Once an expression is understood, the next step is simplifying or evaluating it. Simplification involves combining like terms and applying the correct order of operations to make the expression easier to work with. Evaluation involves substituting specific values for the variables and performing the operations to calculate the result.
Simplifying Polynomial Equations
Simplifying polynomial equations is a key skill for solving complex mathematical problems. This process involves reducing an equation to its simplest form, making it easier to solve or analyze. By combining like terms and applying basic operations, you can simplify polynomials and solve them more efficiently.
Combining Like Terms
One of the first steps in simplifying a polynomial equation is identifying and combining like terms. Like terms have the same variable raised to the same power. For example, in the expression 3x² + 5x + 2x² – 4x, the terms 3x² and 2x² are like terms, as are 5x and -4x. Simplifying these results in 5x² + x.
Applying the Distributive Property
Another important technique is using the distributive property to eliminate parentheses and simplify the expression. For example, in the equation 2(x + 3), you distribute the 2 to each term inside the parentheses, resulting in 2x + 6. This helps eliminate unnecessary grouping and simplifies the overall equation.
Solving Linear Equations Efficiently
Solving linear equations is a fundamental skill that allows you to find unknown values quickly and accurately. The process involves isolating the variable on one side of the equation using a series of systematic steps. With the right approach, you can solve these equations efficiently, ensuring that your solutions are both correct and easy to find.
Isolating the Variable
The key to solving a linear equation is isolating the variable. This involves moving all terms with the variable to one side of the equation and the constant terms to the other side. For example, in the equation 2x + 3 = 7, you subtract 3 from both sides to get 2x = 4, and then divide both sides by 2 to find x = 2.
Handling Fractions and Decimals
When dealing with equations that contain fractions or decimals, it’s often helpful to eliminate them early on. Multiply through by the least common denominator (LCD) or multiply both sides by 10 or 100 to clear decimal points. This makes the equation simpler to solve and reduces the risk of errors.
Mastering Factoring Techniques
Factoring is a critical skill that simplifies complex expressions and makes solving equations much easier. By breaking down polynomials into simpler factors, you can more effectively find solutions or simplify further work. Mastering these techniques allows you to approach problems with greater confidence and speed.
Factoring Trinomials
Factoring trinomials, particularly those in the form ax² + bx + c, requires finding two numbers that multiply to ac and add to b. For example, in the expression x² + 5x + 6, you need to find two numbers that multiply to 6 and add to 5, which are 2 and 3. The factored form is (x + 2)(x + 3).
Difference of Squares
Another common factoring technique is factoring the difference of squares. This applies to expressions in the form a² – b², which factors as (a + b)(a – b). For instance, x² – 9 can be factored as (x + 3)(x – 3). Recognizing and applying this pattern can significantly speed up solving equations that involve squared terms.
Graphing Linear Functions and Slopes
Graphing straight-line equations and understanding their slopes are essential skills for visualizing relationships between variables. By plotting these functions on a coordinate plane, you can easily interpret how one variable changes in relation to another. The slope represents the rate of change, and learning to calculate it accurately helps in understanding the behavior of the graph.
When graphing a linear function, the general form is often written as y = mx + b, where m represents the slope and b is the y-intercept. The slope determines how steep the line is, and the y-intercept shows where the line crosses the y-axis. Let’s take a closer look at how to interpret and plot this information.
Example of Slope Calculation
The slope of a line can be calculated using the formula: m = (y₂ – y₁) / (x₂ – x₁). This formula gives the ratio of the vertical change to the horizontal change between two points on the line. Below is a simple table that shows how to compute the slope from two points on a line:
| Point 1 (x₁, y₁) | Point 2 (x₂, y₂) | Slope (m) |
|---|---|---|
| (2, 3) | (4, 7) | (7 – 3) / (4 – 2) = 4 / 2 = 2 |
In this case, the slope of the line is 2, meaning that for every 1 unit increase in the x-direction, the y-value increases by 2 units.
Solving Systems of Equations
Solving systems of equations involves finding the values of variables that satisfy multiple equations simultaneously. This process is essential for solving real-world problems where multiple conditions must be met at once. There are several methods available to tackle such systems, each offering a unique approach to finding the solution efficiently.
Below are three common methods for solving systems of equations:
- Substitution Method: In this method, you solve one equation for one variable and substitute that expression into the other equation. This eliminates one variable, allowing you to solve for the remaining one.
- Elimination Method: This method involves adding or subtracting the equations to eliminate one variable. After simplifying the resulting equation, you can solve for the remaining variable.
- Graphing Method: By graphing both equations on a coordinate plane, the solution is the point where the two lines intersect. This method is particularly useful for visualizing the solution.
Each method has its advantages depending on the nature of the system, and practicing these techniques will help you choose the most efficient method for different types of problems.
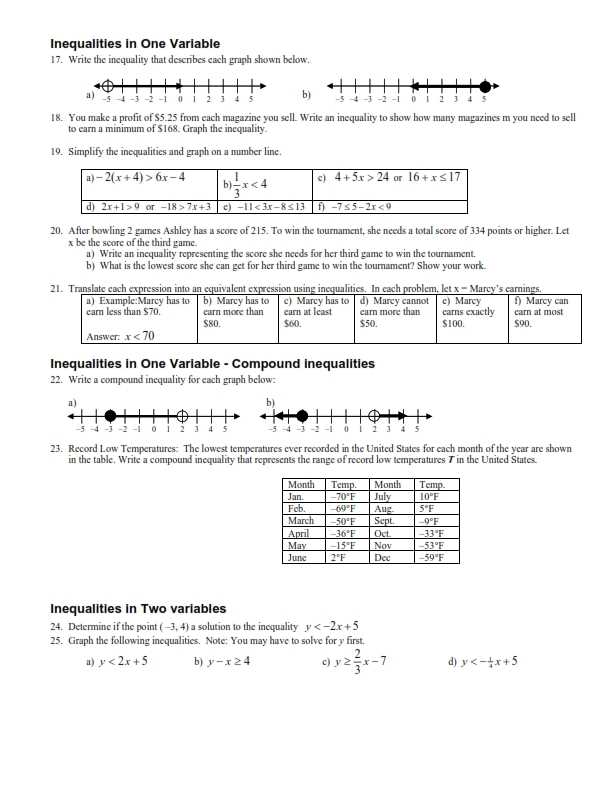
Exploring Inequalities and Their Solutions
Inequalities represent relationships where one value is greater than, less than, or not equal to another. Unlike equations, where the goal is to find exact values for the variables, inequalities provide a range of possible solutions. Understanding how to solve and graph these relationships is crucial for analyzing situations where exact equality is not required.
There are several types of inequalities, and each requires a specific approach for finding solutions:
- Linear Inequalities: These involve expressions where the variable is raised to the first power. Solving linear inequalities is similar to solving linear equations, with the important difference being that the inequality sign may flip when multiplying or dividing by a negative number.
- Compound Inequalities: These are two or more inequalities combined into one statement, often using “and” or “or” to describe the relationship between the solutions. Understanding how to handle these combined inequalities is key to finding the correct solution set.
- Quadratic Inequalities: In these cases, the variable appears with a power of 2. Solving these requires factoring or using the quadratic formula, followed by analyzing the sign of the expression to determine the solution range.
When solving inequalities, it is important to remember how the inequality sign behaves, especially when multiplying or dividing by negative numbers. Additionally, solutions are often expressed in interval notation or graphed on a number line to represent the full range of possible answers.
Working with Exponents and Powers
Understanding exponents and powers is fundamental for simplifying expressions and solving equations efficiently. When working with powers, you deal with repeated multiplication, where the base is multiplied by itself a specified number of times. Knowing the rules for handling exponents allows you to manipulate complex expressions and solve problems more effectively.
There are several key concepts to keep in mind when dealing with exponents:
- Product of Powers: When multiplying two expressions with the same base, add the exponents. For example, am × an = am+n.
- Power of a Power: When raising a power to another power, multiply the exponents. For example, (am)n = am×n.
- Quotient of Powers: When dividing two expressions with the same base, subtract the exponents. For example, am / an = am-n.
- Negative Exponents: A negative exponent indicates the reciprocal of the base raised to the positive exponent. For example, a-n = 1/an.
- Zero Exponent: Any nonzero number raised to the power of zero equals 1. For example, a0 = 1.
By mastering these rules, you can simplify and manipulate expressions that involve exponents, making it easier to solve more complex problems. Whether dealing with small numbers or larger equations, understanding exponents helps you work more efficiently and accurately.
Understanding Quadratic Functions
Quadratic functions are a type of mathematical relationship where the highest power of the variable is squared. These functions describe parabolic curves when graphed, and they are common in many areas of mathematics and science. Understanding how to work with these functions is crucial for solving problems that involve curved relationships between variables.
The general form of a quadratic function is:
f(x) = ax2 + bx + c
Where a, b, and c are constants, and x is the variable. The coefficient a determines the direction and width of the parabola, while b and c affect the position of the curve.
Key Features of Quadratic Functions
There are several key features to understand when working with quadratic functions:
- Vertex: The vertex is the highest or lowest point on the parabola, depending on the direction it opens. The x-coordinate of the vertex can be found using the formula x = -b / 2a.
- Axis of Symmetry: This is a vertical line that passes through the vertex and divides the parabola into two equal halves. The equation for the axis of symmetry is x = -b / 2a.
- Roots or Zeros: These are the points where the quadratic function intersects the x-axis. They can be found by solving the equation ax2 + bx + c = 0, either by factoring, completing the square, or using the quadratic formula.
Graphing Quadratic Functions
When graphing a quadratic function, it’s important to plot the vertex, axis of symmetry, and any intercepts. The shape of the graph will be a parabola, opening upwards if a is positive and downwards if a is negative. This visual representation helps in understanding the behavior of the function and finding its solutions.
Solving Quadratic Equations by Factoring
Factoring is a powerful method for solving equations where the highest power of the variable is squared. This technique involves rewriting the equation as a product of two binomials, making it easier to find the values of the variable that satisfy the equation. Understanding how to factor quadratic expressions is essential for solving many algebraic problems quickly and effectively.
To solve a quadratic equation by factoring, follow these steps:
- Move all terms to one side: Ensure the equation is in the standard form, ax2 + bx + c = 0. If necessary, rearrange the terms.
- Factor the quadratic expression: Look for two numbers that multiply to give ac and add up to b. Use these numbers to break up the middle term and factor the expression.
- Set each factor equal to zero: After factoring, set each binomial equal to zero and solve for the variable.
- Find the solutions: Solve the resulting linear equations to find the values of x.
Example of Factoring a Quadratic Equation
Consider the equation:
x2 + 5x + 6 = 0
First, factor the quadratic expression. We need two numbers that multiply to 6 and add up to 5. These numbers are 2 and 3. So, the factored form is:
(x + 2)(x + 3) = 0
Now, set each factor equal to zero:
x + 2 = 0 or x + 3 = 0
Solving these gives:
x = -2 or x = -3
The solutions to the equation are x = -2 and x = -3.
When Factoring is Not Possible
Sometimes, factoring may not be straightforward, or the quadratic expression may not factor neatly. In these cases, other methods like completing the square or using the quadratic formula may be more appropriate for finding the solutions.
Utilizing the Quadratic Formula

When faced with an equation involving a variable raised to the second power, solving for the unknown can be done in a variety of ways. One of the most efficient methods for finding the solutions is through the use of a formula specifically designed for this type of equation. This formula allows us to quickly find the values of the variable that satisfy the equation, even when factoring is not an option.
To use this formula, the equation must first be in the standard form:
ax2 + bx + c = 0
Where a, b, and c are constants, and x represents the unknown. The quadratic formula is written as:
x = (-b ± √(b² – 4ac)) / 2a
This formula involves substituting the values of a, b, and c from the equation into the expression. By following through the necessary steps, the two possible values for x can be found, as indicated by the ± symbol.
For instance, consider the equation:
2x2 + 4x – 6 = 0
Here, a = 2, b = 4, and c = -6. Substituting these values into the formula:
x = (-(4) ± √((4)2 – 4(2)(-6))) / 2(2)
Simplifying the expression inside the square root:
x = (-4 ± √(16 + 48)) / 4
x = (-4 ± √64) / 4
x = (-4 ± 8) / 4
Now we can solve for the two possible values of x:
- x = (-4 + 8) / 4 = 4 / 4 = 1
- x = (-4 – 8) / 4 = -12 / 4 = -3
The solutions to this equation are x = 1 and x = -3.
Using the quadratic formula is a reliable and straightforward method for solving second-degree equations. Whether the equation has real or complex solutions, this formula can be applied universally, ensuring accurate results every time.
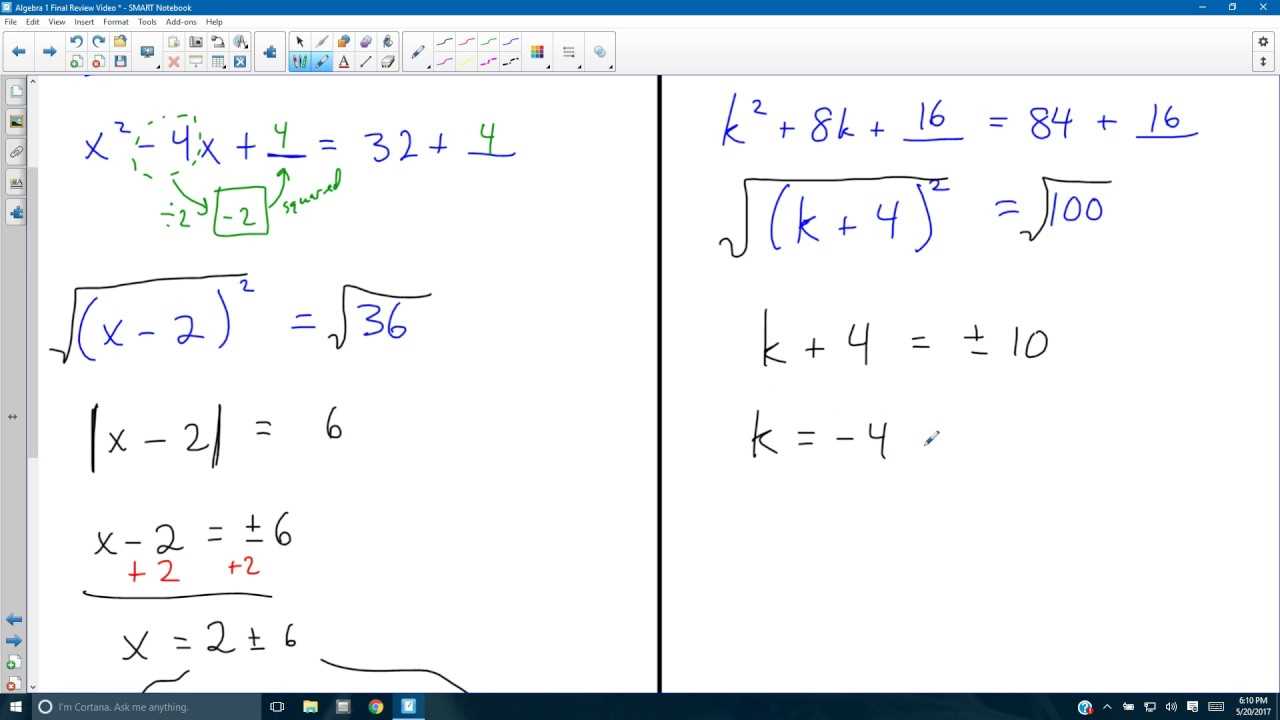
Understanding Absolute Value Equations
When working with mathematical equations, there are certain scenarios where the magnitude of a number is more important than its sign. This concept is captured by absolute value, which measures the distance of a number from zero on the number line, without considering whether the number is positive or negative. Absolute value equations present a unique challenge because they can have two possible solutions, one positive and one negative, for each given scenario.
The Basics of Absolute Value

Absolute value is denoted by two vertical bars surrounding a number or expression. For example, the absolute value of -3 is written as | -3 |, which equals 3. Likewise, the absolute value of 3, written as | 3 |, is also 3. This tells us that the distance of both -3 and 3 from zero is 3 units, but their signs are ignored in the calculation.
Solving Absolute Value Equations
When solving equations that include absolute values, it is essential to consider both the positive and negative possibilities for the variable. For example, if we have the equation:
| x + 4 | = 7
To solve this, we recognize that the expression inside the absolute value brackets could either be 7 or -7 because both values would have an absolute value of 7. This leads to two separate equations:
- x + 4 = 7
- x + 4 = -7
Now, solving each equation:
- For x + 4 = 7, subtract 4 from both sides to get x = 3.
- For x + 4 = -7, subtract 4 from both sides to get x = -11.
Thus, the solutions to the equation | x + 4 | = 7 are x = 3 and x = -11.
It’s important to remember that not all absolute value equations will have real solutions. For example, if we had an equation like:
| x + 2 | = -5
There would be no solution, because absolute values cannot be negative. This highlights the importance of considering the properties of absolute values when solving such equations.
Exploring Radical Expressions
In mathematics, some problems require dealing with numbers that are not simple whole numbers or decimals. These situations often involve the concept of extracting roots or working with expressions that include these roots. Such expressions are essential in various fields of study, from geometry to calculus, and understanding them is crucial for solving more complex problems. Radical expressions are those that contain a root symbol, typically indicating square roots or higher roots, and they can often be simplified or manipulated for easier problem solving.
What is a Radical Expression?
A radical expression typically includes a root sign (√) and an expression under the sign. For example, the square root of a number 9 is written as √9, which simplifies to 3. The number inside the radical is called the radicand, and the root symbol indicates that you are looking for a number that, when multiplied by itself, gives the radicand. Radical expressions can also represent cube roots, fourth roots, and so on, depending on the index of the radical.
Basic Operations with Radical Expressions

When working with radical expressions, there are several key operations that can simplify or combine expressions for easier handling. These include simplifying radicals, adding or subtracting like radicals, and multiplying or dividing radicals. Below is a table illustrating these basic operations.
| Operation | Example | Result |
|---|---|---|
| Simplifying | √18 | √9 × √2 = 3√2 |
| Adding/Subtracting | 3√2 + 4√2 | 7√2 |
| Multiplying | √3 × √12 | √36 = 6 |
| Dividing | √8 ÷ √2 | √4 = 2 |
As seen in the table, simplifying square roots involves factoring the radicand to find perfect squares. Adding and subtracting radicals is only possible when the radicands are identical. For multiplication and division, radical expressions follow the same rules as regular algebraic terms, where the product or quotient of square roots is simply the square root of the product or quotient of the radicands.
Mastering these operations allows for easier manipulation and simplification of radical expressions, which is essential for solving more complex equations and problems efficiently.
Mastering Word Problems in Algebra
Word problems are a key challenge in mathematics, requiring the translation of real-life scenarios into mathematical expressions and equations. To solve these types of problems, it is essential to understand how to break down the situation into manageable parts. By identifying the variables, relationships, and required calculations, you can form equations that represent the problem and find the solution step by step. Mastering this process involves practicing how to interpret the language of the problem and applying appropriate mathematical techniques to arrive at the correct answer.
When approaching word problems, start by carefully reading the problem to determine what is being asked. Look for key phrases or numbers that provide hints about the relationships between different quantities. Often, word problems will describe a situation involving rates, amounts, or other quantities that can be represented with variables and equations.
Once the variables are identified, the next step is to write an equation based on the relationships described in the problem. For example, a problem may describe a situation involving distance, time, and speed, where the relationship between these quantities is expressed with a linear equation. After forming the equation, solve for the unknown variable using appropriate mathematical operations, such as addition, subtraction, multiplication, or division.
As you continue practicing, you will develop the ability to recognize common patterns in word problems, which will help you solve them more efficiently. With enough experience, translating a word problem into a solvable equation becomes a natural skill that significantly improves problem-solving confidence.
Common Mistakes to Avoid During the Test
When preparing for and taking a test, it’s important to be aware of common errors that can cost valuable points. These mistakes often arise from a lack of attention to detail, hasty calculations, or misunderstanding key concepts. Recognizing and avoiding these pitfalls can significantly improve your performance. To avoid these missteps, it’s essential to stay focused, take your time, and double-check your work to ensure accuracy.
One of the most frequent mistakes is rushing through the problems without fully reading the instructions. Skimming through questions can lead to misinterpretation of what is being asked, which may result in incorrect solutions. Always read each question carefully and identify the key components before attempting to solve it.
Another common error is neglecting to check your work. After completing a problem, it’s crucial to go back and verify the calculations. Simple arithmetic mistakes, like adding or subtracting incorrectly, can have a significant impact on the outcome. A quick review can help catch these small but important errors.
Also, remember not to overlook units or details in word problems. Ignoring the context of a question can lead to choosing the wrong formula or applying the wrong method. Always ensure that you’re using the correct approach for each type of problem.
By staying focused, managing your time effectively, and thoroughly reviewing your work, you can avoid these common mistakes and boost your overall performance. Confidence in your problem-solving process, combined with careful attention to detail, is key to success.
Effective Time Management Strategies
Efficiently managing your time is crucial for tackling tasks and ensuring success in any academic setting. By planning and prioritizing your workload, you can reduce stress and enhance productivity. Time management is not just about working harder but also working smarter. Developing a solid strategy allows you to allocate your time wisely, helping you stay organized and focused.
Here are some strategies that can help you manage your time more effectively:
- Prioritize Tasks: Identify the most important tasks and tackle them first. Focus on what needs to be completed today and what can wait. Breaking down complex tasks into smaller, manageable steps helps maintain focus.
- Create a Schedule: Plan out your day or week in advance. Set aside specific time slots for studying, breaks, and other activities. Having a structured schedule can keep you on track and prevent last-minute rushes.
- Avoid Multitasking: While multitasking may seem efficient, it often leads to mistakes and reduced focus. Instead, dedicate your attention to one task at a time to ensure higher quality work.
- Set Realistic Goals: Make sure your goals are achievable within the given time frame. Setting unrealistic expectations can lead to frustration. Break large projects into smaller tasks and celebrate each accomplishment.
- Take Regular Breaks: Avoid burnout by taking short breaks during study sessions. A 5–10 minute break every hour can help refresh your mind and improve concentration.
By implementing these strategies, you can manage your time more effectively, reduce stress, and increase your chances of success. Time management isn’t about getting everything done in one go but about organizing your efforts and balancing priorities effectively.