Algebra 1 Semester 2 Final Exam Review Answers

Preparing for a challenging academic assessment requires a solid understanding of the core principles and methods that will be tested. This guide focuses on equipping you with the necessary knowledge and strategies to approach various topics effectively. Whether you’re revisiting essential concepts or refining your problem-solving techniques, this resource will help you tackle even the most complex questions with confidence.

Mastering key mathematical concepts is crucial for achieving success. This guide delves into the foundational topics that you will encounter, ensuring that you not only grasp theoretical knowledge but also apply it practically. From simplifying expressions to solving complex equations, every step is designed to enhance your understanding and ability to perform well under pressure.

With focused practice and a clear approach, you can overcome common hurdles that many face during assessments. By breaking down each concept into manageable sections, you will gain a clearer understanding of how to navigate through various problems efficiently. This resource aims to simplify the learning process, helping you feel prepared and confident when it’s time to test your skills.

Algebra 1 Semester 2 Exam Overview

In the second part of the academic year, students are expected to demonstrate their proficiency in key mathematical concepts that have been covered. The focus shifts to more advanced problem-solving techniques and the application of mathematical principles learned earlier. This section provides an overview of the critical topics you need to understand and the skills required to excel in your assessment.

Core Areas of Focus

Linear equations and inequalities
Working with functions and their graphs
Systems of equations and methods of solving them
Polynomial expressions and factoring techniques
Quadratic equations and their properties
Rational expressions and simplifying techniques

Important Skills to Master

Solving complex word problems using systematic methods
Graphing equations and interpreting their results
Manipulating expressions involving fractions, exponents, and radicals
Identifying and correcting common mistakes
Time management and strategic problem-solving

Having a strong grasp of these areas is essential for achieving success. Make sure to allocate sufficient time to practice each concept, focusing on both theoretical understanding and practical application. A well-rounded preparation will help you approach any question with confidence and clarity.

Key Topics for Final Exam Success

Success in any assessment depends on a deep understanding of the main concepts that will be tested. Focusing on the most important areas and practicing key problem-solving methods can make all the difference. This section highlights the essential topics that you should concentrate on to ensure you’re well-prepared and ready to tackle the most challenging questions with ease.

Critical Mathematical Concepts

Linear relationships and their graphical representations
Solving and interpreting systems of equations
Understanding functions and their behavior
Factoring and simplifying polynomial expressions
Working with rational and radical expressions
Solving quadratic equations using different methods

Problem-Solving Techniques

Breaking down complex problems into manageable steps
Using substitution and elimination methods in systems of equations
Graphing equations accurately to interpret solutions
Identifying patterns and applying them to solve unknowns
Mastering time management during problem-solving

By thoroughly understanding and practicing these key topics, you’ll be able to approach the assessment with confidence and clarity. Make sure to review each area systematically and ensure you’re comfortable solving a variety of problems in each category.

How to Tackle Word Problems

Word problems often pose a unique challenge because they require translating a narrative into mathematical expressions and solving them. Approaching these problems systematically is key to finding the correct solution. By breaking down each problem into smaller, manageable steps, you can improve your ability to solve even the most complex scenarios.

Steps to Solve Word Problems Effectively

Read the problem carefully: Understand the context and identify the key information.
Identify the unknown: Determine what needs to be solved for and represent it with a variable.
Translate words into equations: Convert the narrative into mathematical expressions or equations that represent the relationships described.
Organize the information: List the known values and what needs to be found. This helps to clarify your approach.
Choose the right method: Decide whether to use methods like substitution, elimination, or direct calculation based on the problem.
Check your work: Once a solution is found, verify it by substituting it back into the original context to ensure it makes sense.

Common Pitfalls to Avoid

Skipping important details in the problem statement
Misinterpreting the relationships between quantities
Overcomplicating the solution process by neglecting simpler methods
Forgetting to double-check answers before finalizing the solution

By following a clear process and avoiding common mistakes, you can develop the confidence to tackle any word problem that comes your way. Practice and familiarity with different problem types will enhance your ability to recognize patterns and apply the appropriate methods quickly and accurately.

Common Mistakes in Algebra Exams

When facing a mathematical assessment, even the most prepared students can fall into certain traps. Mistakes often occur due to misunderstandings of concepts, careless calculations, or overlooking key details in the problem. Being aware of these common errors can help you avoid them and improve your overall performance.

Frequent Errors to Watch Out For

Misinterpreting the problem or missing critical information
Skipping steps in multi-step calculations, leading to incorrect answers
Forgetting to distribute terms properly when solving equations
Incorrectly applying the rules of operations, especially with exponents and negative numbers
Rushing through word problems and not carefully setting up equations

How to Avoid These Mistakes

Read each problem thoroughly and identify all relevant details before starting
Write out each step in the solution process to avoid skipping crucial steps
Double-check calculations, especially when working with negative numbers and fractions
Take time to solve word problems carefully, translating the language into correct equations
Review solutions after completing them to ensure consistency and accuracy

By remaining vigilant and methodical, you can reduce the likelihood of making these common mistakes. With practice, you will become more adept at recognizing potential pitfalls and ensuring your approach is clear and precise.

Mastering Equations and Inequalities

To excel in problem-solving, it is essential to have a strong command of equations and inequalities. These concepts form the foundation of many mathematical challenges, requiring a systematic approach to isolate variables and determine solutions. By mastering these techniques, you can efficiently handle a variety of mathematical problems, whether dealing with simple equations or more complex inequalities.

Steps to Solve Equations

Identify the equation type: Determine whether the equation is linear, quadratic, or involves other operations.
Isolate the variable: Perform inverse operations to move all terms involving the variable to one side.
Simplify the equation: Combine like terms and simplify fractions to make the equation easier to solve.
Check the solution: Substitute the solution back into the original equation to ensure it satisfies both sides.

Approaching Inequalities

Understand the inequality symbol: Pay attention to whether the inequality is , ≤, or ≥ as this affects the solution set.
Follow similar steps as for equations: Isolate the variable and simplify, but be mindful that when multiplying or dividing by a negative number, the inequality sign must be reversed.
Graph the solution: For inequalities, graphing the solution on a number line can help visualize the range of possible values.

Consistent practice with these techniques will help you become more comfortable solving both equations and inequalities. With time, you’ll develop the ability to approach these problems efficiently and accurately, ensuring success in various types of challenges.

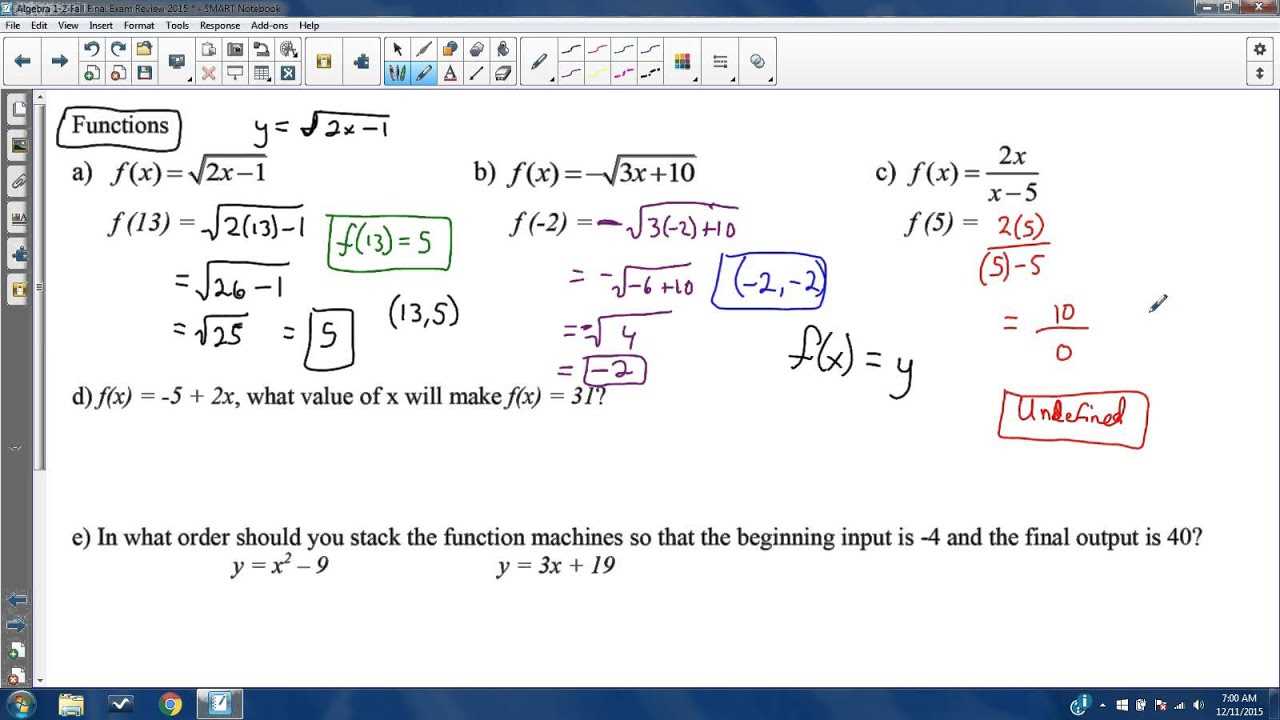
Understanding Functions in Algebra

Functions are a fundamental concept in mathematics that describe the relationship between two sets of values. Essentially, a function assigns exactly one output for every input, establishing a predictable pattern. Gaining a deep understanding of functions is key to solving a wide range of problems, from simple relationships to more complex expressions and equations. Whether working with linear functions or more advanced types, the basic principles remain the same.

Key Elements of Functions

Term	Description
Domain	The set of all possible inputs (x-values) for which the function is defined.
Range	The set of all possible outputs (y-values) resulting from the function.
Function Notation	Typically written as f(x), where “f” represents the function and “x” is the input value.
One-to-One Relationship	Each input corresponds to exactly one output, ensuring a clear and predictable mapping.

Types of Functions

There are many types of functions, each with its own characteristics and applications. Some of the most common include:

Linear Functions: Represented by a straight line when graphed, these functions follow the form y = mx + b, where m is the slope and b is the y-intercept.
Quadratic Functions: Represented by a parabola, these functions have the form y = ax² + bx + c.
Exponential Functions: These functions grow or decay at a constant rate, typically written as y = ab^x.
Absolute Value Functions: These create V-shaped graphs and are defined by y = |x|.

Understanding the different types of functions and their properties allows you to interpret data, solve equations, and graph relationships accurately. Mastery of this concept is essential for tackling a wide range of problems in higher mathematics and real-world applications.

Solving Systems of Equations

When faced with multiple relationships between variables, solving a system of equations allows you to find the values that satisfy all equations simultaneously. The goal is to determine a common solution that works for all given conditions. There are several methods available for solving these systems, each suited to different types of problems.

Methods for Solving Systems

Three common techniques can be used to solve a system of equations: substitution, elimination, and graphing. Each approach has its strengths and can be applied depending on the nature of the equations.

Substitution Method: This method involves solving one equation for a variable and substituting the expression into the other equation. This creates a simpler equation with one unknown, making it easier to solve.
Elimination Method: By adding or subtracting the equations in such a way that one variable is eliminated, you can solve for the remaining variable. This method is particularly useful when the coefficients of one variable are the same or opposites.
Graphing Method: This method involves plotting both equations on a coordinate plane. The point where the lines intersect is the solution to the system. While useful for visualization, it may not always yield an exact solution unless the lines intersect at integer values.

Tips for Success

Double-check calculations: Small mistakes in substitution or elimination can lead to incorrect solutions, so be sure to review each step carefully.
Choose the best method: Some systems are easier to solve using one method over another. If the coefficients are easy to manipulate, elimination may be the fastest approach. If one equation is already solved for a variable, substitution is often the best choice.
Verify the solution: Once a solution is found, substitute the values of the variables back into the original equations to ensure they hold true for all conditions.

By mastering these methods, you can confidently solve any system of equations that comes your way. With practice, you will develop the skills needed to handle even the most complex systems efficiently.

Polynomials and Their Properties

Polynomials are expressions that consist of variables raised to various powers, combined with coefficients. These mathematical objects are fundamental in many areas of mathematics, as they describe a wide variety of relationships. Understanding their structure and characteristics is essential for solving problems that involve more complex equations and expressions.

At the core of working with polynomials is the ability to recognize their terms, degrees, and how to manipulate them. Polynomials can be added, subtracted, multiplied, and factored, each operation revealing different aspects of their properties. The degree of a polynomial, which is determined by the highest power of the variable, plays a crucial role in determining its behavior and how it can be solved.

Key Properties of Polynomials

Degree: The degree of a polynomial is the highest exponent of the variable in the expression. It indicates the polynomial’s overall behavior and the number of roots it may have.
Leading Coefficient: The coefficient of the term with the highest degree is called the leading coefficient. It helps determine the end behavior of the polynomial function.
Constant Term: The constant term is the part of the polynomial that does not involve the variable. It represents the value of the polynomial when the variable is set to zero.
Roots or Zeros: The roots of a polynomial are the values of the variable that make the polynomial equal to zero. Finding these roots is often the goal when solving polynomial equations.

Operations with Polynomials

Addition and Subtraction: Polynomials can be added or subtracted by combining like terms, which are terms that have the same variable and exponent.
Multiplication: When multiplying polynomials, distribute each term of one polynomial to every term of the other. This process can result in higher-degree terms.
Factoring: Factoring polynomials involves expressing the polynomial as a product of simpler polynomials. This is often useful for solving equations or simplifying expressions.

With a strong grasp of polynomials and their properties, you will be well-equipped to handle a variety of problems, from basic operations to more advanced techniques involving factoring and solving polynomial equations. The key to mastering this area is practice and familiarity with the different forms and behaviors of polynomials.

Working with Rational Expressions

Rational expressions are fractions where both the numerator and the denominator are polynomials. These expressions are encountered frequently in various mathematical problems and require specific techniques for simplification, manipulation, and solving. Understanding how to work with them efficiently is essential for tackling more complex equations and systems that involve ratios of polynomial functions.

When handling rational expressions, one key task is simplifying them by factoring both the numerator and the denominator. This often allows you to cancel out common factors, reducing the expression to its simplest form. Additionally, it is important to recognize restrictions on the variable, as certain values can make the denominator zero, leading to undefined expressions.

Key Steps in Simplifying Rational Expressions

Factor the Numerator and Denominator: Start by factoring both the top and bottom parts of the fraction completely. This makes it easier to identify common factors that can be canceled.
Cancel Common Factors: If the numerator and denominator share any factors, these can be eliminated from the expression to simplify it.
Rewrite the Expression: After factoring and canceling common terms, rewrite the simplified form of the rational expression.

Operations with Rational Expressions

Multiplication: When multiplying rational expressions, multiply the numerators together and the denominators together. Be sure to factor and cancel any common terms before multiplying.
Division: To divide by a rational expression, multiply by its reciprocal. As with multiplication, factor and cancel any common terms first.
Addition and Subtraction: To add or subtract rational expressions, find a common denominator. Once the denominators are the same, you can combine the numerators, then simplify the result.

By mastering the techniques for simplifying and manipulating rational expressions, you can efficiently solve problems involving fractions and equations that include polynomial terms. Practice and familiarity with the rules of operations will help you gain confidence in working with these expressions.

Graphing Linear Equations Efficiently

Graphing linear equations is a fundamental skill in mathematics that allows you to visualize the relationship between variables. Efficiently plotting these equations can save time and help you understand the behavior of the function. The key to graphing quickly and accurately is mastering the slope and intercept form, which provides a direct way to plot a line using just two points.

By focusing on the key elements–slope and y-intercept–you can easily sketch the graph without requiring extensive calculations. The slope indicates the angle of the line, while the y-intercept gives the point where the line crosses the vertical axis. With these two values, you can plot the line and extend it in both directions to complete the graph.

Key Steps for Efficient Graphing

Identify the Slope: The slope of a line is determined by the ratio of the change in y (vertical direction) to the change in x (horizontal direction). In the equation y = mx + b, m represents the slope.
Find the Y-Intercept: The y-intercept is the point where the line crosses the y-axis. In the equation y = mx + b, b represents this value.
Plot the Y-Intercept: Start by marking the y-intercept on the graph, which is the point (0, b) on the vertical axis.
Use the Slope to Find Another Point: From the y-intercept, use the slope to determine the second point on the line. For example, a slope of 2 means you move up 2 units and to the right 1 unit to find the next point.
Draw the Line: After plotting the two points, draw a straight line through them, extending it in both directions.

Tips for Quick and Accurate Graphing

Use Graph Paper: Graph paper helps maintain accuracy when plotting points and drawing lines, ensuring that your graph is neat and proportional.
Check Your Work: Always check that your line passes through the y-intercept and follows the slope correctly.
Practice Different Slopes: Get comfortable with both positive and negative slopes, as well as horizontal and vertical lines (where the slope is zero or undefined).

By practicing these steps, you can graph linear equations more efficiently, saving time and increasing your accuracy. With experience, you’ll develop the ability to visualize and graph equations quickly, helping you tackle problems with confidence.

Exploring Quadratic Equations

Quadratic equations are a crucial part of understanding how relationships between variables can create curves instead of straight lines. These equations often appear in many real-world situations, such as calculating the trajectory of objects or modeling certain types of growth. The general form of a quadratic equation is a polynomial where the highest exponent of the variable is squared, creating a parabolic shape when graphed.

Solving quadratic equations requires understanding the various methods available to find their solutions, such as factoring, completing the square, and using the quadratic formula. Each method offers a different approach to solving, but all ultimately lead to the same solution set, providing insights into the nature of the equation.

Methods for Solving Quadratic Equations

Factoring: When a quadratic equation can be factored, you can rewrite it as a product of two binomials. Setting each factor equal to zero gives the solutions.
Completing the Square: This method involves rearranging the equation to form a perfect square trinomial, allowing you to solve for the variable more easily.
Quadratic Formula: This is a universal method for solving any quadratic equation. The formula x = (-b ± √(b² – 4ac)) / 2a provides the solutions directly, regardless of whether the equation can be factored.

Graphing and Interpreting Quadratics

When you graph a quadratic equation, the result is a parabola. The vertex of this parabola represents the maximum or minimum value of the quadratic function, depending on whether the parabola opens upward or downward. Understanding how to identify the vertex, axis of symmetry, and intercepts on the graph is key to interpreting the behavior of the equation.

Vertex: The highest or lowest point on the parabola, depending on its direction.
Axis of Symmetry: A vertical line that divides the parabola into two mirror-image halves, passing through the vertex.
Intercepts: The points where the parabola crosses the x-axis (real solutions) and the y-axis.

By exploring quadratic equations, you gain a deeper understanding of how variables can relate in nonlinear ways, helping you solve complex problems in both mathematics and the real world.

Tips for Simplifying Radical Expressions

Simplifying radical expressions is a key skill in understanding how to handle roots and powers in mathematical operations. These expressions often appear as square roots, cube roots, or other nth roots, and simplifying them can make solving problems much easier. The process involves breaking down complex expressions into simpler components, allowing you to work with them more efficiently.

One effective strategy is to look for perfect squares or cubes within the radicand. By factoring out these perfect powers, you can simplify the expression significantly. Another important step is to combine like terms when possible, just as you would with regular algebraic expressions. Lastly, rationalizing the denominator is a common technique used to eliminate roots from the bottom of fractions, making the expression easier to handle.

Factor the Radicand: Look for perfect squares, cubes, or other roots within the radicand. For example, √36 simplifies to 6 because 36 is a perfect square.
Combine Like Terms: If there are multiple terms under the same root, simplify them together where possible. For instance, √8 + √2 can be simplified to 3√2.
Rationalize the Denominator: If the denominator contains a root, multiply both the numerator and denominator by a term that will eliminate the root from the denominator.

By following these tips, you’ll be able to handle radical expressions with greater ease, improving your ability to solve problems accurately and efficiently.

Exponents and Their Rules

Understanding exponents is essential for working with powers and exponential growth in mathematical expressions. Exponents represent repeated multiplication of a number by itself, which simplifies complex calculations and makes it easier to express large or small numbers efficiently. There are several rules and properties that help manage and simplify expressions involving exponents, allowing you to handle various operations with greater ease.

Here are the key rules that govern how exponents interact in different operations:

Rule	Explanation	Example
Product Rule:	When multiplying two numbers with the same base, add the exponents.	a^m × a^n = a^(m+n)
Quotient Rule:	When dividing two numbers with the same base, subtract the exponents.	a^m ÷ a^n = a^(m-n)
Power Rule:	When raising a number with an exponent to another power, multiply the exponents.	(a^m)^n = a^(m×n)
Zero Exponent:	Any non-zero number raised to the power of zero equals 1.	a^0 = 1 (a ≠ 0)
Negative Exponent:	Negative exponents represent the reciprocal of the base raised to the positive exponent.	a^(-n) = 1/a^n

By mastering these exponent rules, you can simplify and manipulate expressions involving powers with confidence, making complex problems more manageable. These principles are not only fundamental in mathematics but also in fields like physics, computer science, and engineering, where exponential growth and decay play a crucial role.

Understanding Algebraic Fractions

Working with fractions involving variables can seem challenging at first, but mastering the basic principles makes them more manageable. These expressions consist of polynomials in both the numerator and denominator, and simplifying or solving them requires understanding the relationship between the terms. Whether adding, subtracting, multiplying, or dividing, handling such fractions efficiently is crucial for solving more complex problems.

To simplify or perform operations with these types of expressions, it is essential to follow a systematic approach:

Identifying Common Denominators: For addition or subtraction, ensuring both expressions have the same denominator is key to combining them.
Factoring: Factoring both the numerator and denominator can simplify expressions significantly and help identify cancelable terms.
Multiplication and Division: When multiplying, multiply the numerators and denominators directly. For division, multiply by the reciprocal of the second fraction.
Canceling Common Factors: Simplifying fractions by canceling out common factors in the numerator and denominator reduces the complexity of the expression.

Once you understand the fundamentals of manipulating these expressions, you can apply the rules to solve a wide range of problems, including those involving equations, inequalities, and more advanced mathematical concepts. Mastery of these techniques provides a solid foundation for tackling more sophisticated topics in mathematics.

Preparing for Multiple Choice Questions

Multiple choice questions often test a student’s ability to apply concepts quickly and accurately. With the right approach, you can maximize your chances of selecting the correct answer. While the format may seem straightforward, it’s essential to employ strategies that allow you to analyze each question thoroughly and make educated decisions under time pressure.

Key Strategies for Success

Read Each Question Carefully: Take the time to fully understand what is being asked before reviewing the answer choices. Often, the phrasing of the question can provide hints about the correct answer.
Eliminate Clearly Incorrect Answers: Start by crossing out any choices that are obviously wrong. This improves your odds of selecting the correct one from the remaining options.
Look for Clues in the Question: Some questions contain subtle hints that can help narrow down the possibilities. Pay attention to keywords or numbers that might guide your reasoning.
Check for Answer Patterns: If you’re unsure between two choices, look for patterns that might indicate the correct answer. For example, if one choice seems to “fit” better with the others, it might be the right option.

Time Management Tips

Don’t Spend Too Much Time on One Question: If you find yourself stuck, move on to the next question and come back later. This helps ensure that you answer all questions within the given time limit.
Practice with Timed Quizzes: Simulating exam conditions with timed quizzes can help improve your speed and reduce anxiety during the actual assessment.

Approaching multiple choice questions with a methodical strategy and good time management can significantly enhance your performance. The more you practice, the more confident you’ll become in your ability to identify the correct answers quickly and accurately.

Time Management During the Exam

Effective time management is key to ensuring you can complete all questions within the allotted time. Whether it’s a series of problem-solving tasks or conceptual questions, knowing how to pace yourself can make a significant difference in your performance. The ability to prioritize and manage your time efficiently can reduce stress and allow you to focus on providing the best possible answers.

Set a Time Limit for Each Section: Before you begin, quickly scan through the entire assessment. Estimate how much time to allocate for each section based on the number of questions or the difficulty level. For instance, if one section has more complex problems, allocate a bit more time to that section and adjust accordingly for the others.

Use a Watch or Timer: Keep track of time throughout the assessment. If you don’t have a watch, set a timer on your phone or ask the proctor for time reminders. Be aware of the time passing, but avoid watching the clock too closely as it can lead to anxiety.

How to Handle Difficult Questions

Don’t Get Stuck: If you encounter a challenging question, move on. Spending too long on one problem can eat up valuable time that you could use on questions you’re more confident about. Come back to difficult questions after you’ve answered the rest.
Mark Unanswered Questions: If you’re unsure about a specific question, mark it and return to it later. Make sure you answer all questions, even if you need to guess. An unanswered question is a guaranteed missed opportunity.

Final Check

Leave Time for Review: Ideally, save the last 5-10 minutes of your time for reviewing your answers. Use this time to check for any mistakes, misread instructions, or incomplete answers. This can help catch errors that might otherwise be overlooked.
Stay Calm and Focused: Don’t panic as time starts running out. Remaining calm and composed will help you think more clearly and make the most of the time you have left.

By managing your time wisely, you’ll be able to approach the assessment with confidence, allowing you to focus on answering each question to the best of your ability.