
As the time approaches for your upcoming math evaluation, it’s crucial to consolidate the knowledge and skills you’ve acquired throughout the course. The key to performing well lies in a solid understanding of core concepts, as well as practicing problem-solving techniques. This guide will help you strengthen your grasp on essential topics, allowing you to approach the test with confidence.
Focus on mastering key principles and reviewing the most challenging areas you’ve encountered. By revisiting fundamental ideas and tackling a variety of practice problems, you can sharpen your ability to apply what you’ve learned effectively. Break down complex problems into manageable parts and refresh your memory on the methods that lead to solutions.
Developing a strong strategy for your preparation can significantly improve your results. Whether it’s understanding intricate relationships between equations or enhancing your problem-solving speed, this section is designed to guide you through the most important aspects of the subject. Be sure to allocate time for practice and review, and you will be well-equipped for the challenge ahead.
Mathematics Assessment Preparation Plan
Preparing effectively for your upcoming math assessment involves a structured approach to ensure thorough understanding and mastery of key concepts. By focusing on the most important topics and practicing regularly, you can enhance both your confidence and problem-solving abilities. A well-organized study schedule will allow you to prioritize areas that require more attention and review others that you are already comfortable with.
Follow this plan to guide your preparation:
- Organize Your Study Materials: Gather all relevant notes, textbooks, and assignments that cover the topics likely to appear in the assessment.
- Identify Core Concepts: Focus on the main areas that are crucial for the test, such as functions, trigonometry, and polynomial equations.
- Practice Problems Regularly: Solve a variety of practice problems to reinforce your understanding and improve problem-solving speed.
- Review Mistakes: When you make errors, take time to understand why they happened and how to avoid them in the future.
- Prioritize Difficult Topics: Allocate more study time to areas you find challenging or those that have been difficult to grasp in class.
Consistency and active practice are key to success. Make sure to set aside time each day for focused study sessions, and don’t hesitate to seek help when you encounter difficult concepts. Following this plan will help you stay on track and achieve your best performance on the assessment.
Key Topics to Focus On
To succeed in your upcoming math assessment, it’s important to concentrate on the essential topics that form the foundation of the subject. These core areas are likely to be the focus of many questions and will test your ability to apply concepts in various contexts. By mastering these key subjects, you’ll be well-prepared to tackle a wide range of problems confidently.
Focus on the following important topics:
- Functions and Their Properties: Understand the different types of functions, such as linear, quadratic, and exponential, and how to analyze their graphs and transformations.
- Trigonometric Identities: Be familiar with basic trigonometric functions and their identities, and practice simplifying expressions involving these functions.
- Polynomials and Rational Expressions: Review methods for factoring polynomials, solving polynomial equations, and simplifying rational expressions.
- Systems of Equations: Focus on solving linear and nonlinear systems, both algebraically and graphically, and understand the methods used to find solutions.
- Sequences and Series: Study arithmetic and geometric sequences, series, and the formulas used to calculate their sums.
By dedicating time to these topics and practicing regularly, you’ll strengthen your understanding and improve your ability to solve complex problems during the assessment.
Understanding Functions and Their Graphs
One of the most important aspects of mathematics is understanding how functions work and how their behavior is represented visually. Functions describe relationships between variables, and their graphs provide a powerful tool to analyze these relationships. Knowing how to interpret and draw graphs of various functions will help you solve problems more effectively and gain deeper insights into the concepts.
Types of Functions

Different types of functions, such as linear, quadratic, and exponential, each have distinct characteristics that influence their graphs. For example, a linear function produces a straight line, while a quadratic function creates a parabola. Exponential functions exhibit rapid growth or decay, depending on the direction of the graph. Understanding the form of the equation is key to predicting the shape and behavior of its graph.
Graphing Techniques
To accurately graph a function, it’s essential to understand its key features, including its intercepts, slopes, and asymptotes. Start by identifying the domain and range of the function, which define the values that the variables can take. Then, plot points that satisfy the function’s equation and connect them to reveal the graph’s overall shape. Pay attention to transformations such as shifts, stretches, and reflections, as these will modify the graph’s appearance.
By mastering these concepts, you will be able to visualize mathematical relationships and use graphs to solve a variety of problems with confidence.
Mastering Trigonometric Identities
Trigonometric identities are fundamental tools used to simplify expressions and solve equations involving angles and lengths in triangles. These identities allow you to transform complex expressions into simpler forms, making them easier to work with. Mastering these identities is crucial for solving a wide range of problems that arise in various fields of mathematics and science.
Key identities, such as the Pythagorean, reciprocal, and quotient identities, provide the foundation for more advanced concepts. By understanding how to apply these identities and recognize their patterns, you can simplify trigonometric equations efficiently. Practice with a variety of problems will help reinforce your ability to manipulate these identities and solve equations with ease.
Knowing when and how to apply the right identity will help you approach problems more strategically and reduce the complexity of the equations you’re working with. Developing proficiency in this area is essential for progressing in your mathematical studies.
Reviewing Polynomial Equations
Polynomial equations play a crucial role in algebra and provide the foundation for understanding more advanced mathematical concepts. These types of equations involve expressions with terms that contain variables raised to different powers. Being able to solve polynomial equations efficiently is essential for handling a variety of problems in mathematics.
Key Concepts to Understand
When working with polynomial equations, it’s important to recognize their key characteristics. Focus on the following concepts:
- Degree of a Polynomial: The highest power of the variable in the equation determines the degree. This will help you identify the number of solutions or roots.
- Factoring Polynomials: Factoring is a powerful method for solving polynomial equations. Look for common factors, and apply methods like grouping or the quadratic formula if necessary.
- Roots and Solutions: The roots of the polynomial equation are the values of the variable that make the equation true. These solutions can often be found by factoring or using numerical methods.
Solving Polynomial Equations
There are several strategies for solving polynomial equations, including factoring, using the Rational Root Theorem, and synthetic division. Begin by trying to factor the polynomial into simpler expressions, and if factoring proves difficult, try other methods such as synthetic or long division. Once factored, set each factor equal to zero to find the roots of the equation.
By mastering these techniques, you will be able to approach polynomial equations with greater confidence and accuracy, allowing you to solve a wide variety of problems more effectively.
Important Limits and Continuity Concepts
Understanding the concepts of limits and continuity is fundamental for analyzing the behavior of functions, particularly as they approach certain values or extend toward infinity. These concepts are critical in calculus and have applications in various mathematical areas. A solid grasp of limits and continuity provides the foundation for further study in more advanced topics such as derivatives and integrals.
Key Limit Concepts
Limits describe how a function behaves as its input approaches a certain value. Mastering this concept is essential for evaluating functions at specific points, particularly when direct substitution fails. Here are some key concepts to focus on:
- One-Sided Limits: These limits examine the behavior of a function as the input approaches a value from either the left or the right side.
- Infinite Limits: These limits deal with functions that grow without bound as the input approaches a certain value.
- Limits at Infinity: These limits analyze the behavior of a function as the input grows larger or smaller without bound.
Understanding Continuity
Continuity of a function ensures that there are no gaps, jumps, or breaks in the graph of the function. A function is continuous at a point if the following conditions are met:
- The function is defined at the point.
- The limit of the function exists at that point.
- The value of the function at the point equals the limit.
Understanding these concepts allows you to identify the behavior of functions under various conditions and is essential for solving more complex problems in calculus.
Working with Rational Expressions
Rational expressions are fractions that involve polynomials in the numerator and denominator. Simplifying, multiplying, dividing, and adding these expressions are essential skills that provide a foundation for solving more complex equations. By mastering rational expressions, you can solve problems involving ratios and proportions more effectively.
Simplifying Rational Expressions
Simplification involves reducing a rational expression to its simplest form. This can be done by factoring both the numerator and denominator and canceling out common factors. It is crucial to look for common factors and factor polynomials whenever possible to make the expression more manageable.
- Factor both the numerator and denominator.
- Cancel out common factors.
- Ensure that no variable makes the denominator equal to zero.
Operations with Rational Expressions
When working with rational expressions, the operations of addition, subtraction, multiplication, and division require specific steps:
- Multiplication and Division: Multiply or divide the numerators and denominators directly. Remember to simplify the result.
- Addition and Subtraction: Find a common denominator first, then combine the numerators. Simplify the final expression.
By practicing these techniques, you will become proficient in working with rational expressions and be able to tackle a wide range of algebraic problems.
Tips for Solving Exponential Problems
Exponential problems often appear in various areas of mathematics, requiring a solid understanding of the properties of exponents and logarithms. These types of problems involve equations where the variable is in the exponent position, which can make solving them more challenging. By applying the right techniques and strategies, you can tackle these problems with confidence.
Understand Exponent Rules
The key to simplifying and solving exponential problems is understanding the basic exponent rules. Familiarity with these rules allows you to manipulate the terms more effectively. Here are the most important exponent rules:
- Product Rule: When multiplying two exponential terms with the same base, add the exponents.
- Quotient Rule: When dividing exponential terms with the same base, subtract the exponents.
- Power Rule: When raising an exponential expression to another exponent, multiply the exponents.
- Zero Exponent Rule: Any number raised to the power of zero is equal to one.
Use Logarithms for Solving Exponential Equations
In many cases, logarithms are used to solve exponential equations. Logarithms allow you to rewrite exponential equations in a form that is easier to handle. Here’s how you can use logarithms effectively:
- Isolate the exponential term: Try to get the exponential term by itself on one side of the equation.
- Apply the logarithm: Take the logarithm of both sides using the same base as the exponential expression.
- Use the logarithmic properties: Use the logarithmic rules to simplify and solve for the variable.
By mastering these techniques and practicing a variety of problems, you will develop a strong foundation for solving exponential equations with ease.
Understanding Logarithmic Functions
Logarithmic functions are the inverse of exponential functions, and they play an essential role in various areas of mathematics, especially in solving equations where the unknown is in the exponent. These functions allow us to transform complex problems into more manageable forms by applying the principles of logarithms. Mastering logarithms opens up the ability to solve a wide range of mathematical problems, from simple equations to more advanced applications in science and engineering.
The basic idea behind logarithms is that they represent the exponent to which a base must be raised to produce a given number. For example, in the equation b^x = y, the logarithmic form is log_b(y) = x. This transformation helps in solving equations where the variable is in the exponent, simplifying the problem into a more straightforward form.
To work effectively with logarithmic functions, it is important to understand several key properties:
- Change of Base Formula: The formula log_b(x) = log_c(x) / log_c(b) allows you to compute logarithms with any base using a different base (typically base 10 or base e).
- Product Rule: log_b(x * y) = log_b(x) + log_b(y), which states that the logarithm of a product is the sum of the logarithms.
- Quotient Rule: log_b(x / y) = log_b(x) – log_b(y), which states that the logarithm of a quotient is the difference of the logarithms.
- Power Rule: log_b(x^n) = n * log_b(x), which allows the exponent to be brought in front of the logarithmic expression.
By understanding these key properties and practicing with different types of problems, you can gain a solid foundation in logarithmic functions, which will make tackling complex equations much easier.
Solving Systems of Equations
Solving systems of equations involves finding the values of variables that satisfy multiple equations simultaneously. These systems can arise in various contexts, from geometry to economics, and solving them helps in finding the intersection points of different relationships. There are several methods to solve these systems, and the choice of method depends on the specific structure of the equations involved.
In general, there are three primary methods for solving systems of equations:
- Graphical Method: By graphing the equations on a coordinate plane, the solution is found at the point where the graphs intersect.
- Substitution Method: One equation is solved for one variable, and the result is substituted into the other equation to find the solution.
- Elimination Method: By adding or subtracting equations, variables are eliminated, simplifying the system to a single equation.
Example of Solving a System Using Substitution
Consider the following system of equations:
| x + y = 6 |
| 2x – y = 3 |
Step 1: Solve the first equation for x:
Step 2: Substitute this value of x into the second equation:
Step 3: Solve for y:
12 – 2y – y = 3
-3y = -9
y = 3
Step 4: Substitute y = 3 into x = 6 – y:
x = 6 – 3
x = 3
The solution to the system is (x, y) = (3, 3).
Conclusion
By using appropriate methods, solving systems of equations becomes a structured and systematic process, enabling you to find the solutions efficiently. Each method has its advantages depending on the complexity and form of the system.
Conic Sections and Their Properties
Conic sections are curves that can be derived from the intersection of a plane and a double-napped cone. These curves appear in various real-world applications, such as in physics, engineering, and astronomy. The four primary types of conic sections include circles, ellipses, parabolas, and hyperbolas, each with its own distinct properties and equations. Understanding the characteristics of these curves is essential for solving many geometric and algebraic problems.
Each conic section has unique attributes that differentiate them from one another. Here’s an overview of these curves and their main properties:
| Conic Section | Standard Equation | Main Characteristics |
|---|---|---|
| Circle | (x – h)² + (y – k)² = r² | All points are equidistant from a central point (h, k). The radius is constant. |
| Ellipse | (x – h)²/a² + (y – k)²/b² = 1 | The sum of the distances from any point on the ellipse to two fixed points (foci) is constant. It has two axes: major and minor. |
| Parabola | y = a(x – h)² + k | It has a single focus and directrix, and the shape opens either upward, downward, left, or right depending on the equation. |
| Hyperbola | (x – h)²/a² – (y – k)²/b² = 1 | It consists of two separate curves called branches. The difference in distances from any point on the hyperbola to two foci is constant. |
Each of these conic sections is derived from varying the angle of the intersecting plane relative to the cone. Understanding how these equations relate to their geometrical properties helps in solving a wide variety of problems, especially in fields that require precise measurements and calculations.
Sequences and Series: A Refresher
Understanding the patterns and sums of numbers is essential for solving many problems in mathematics. Sequences and series are fundamental concepts that describe ordered sets of numbers and their corresponding sums. By recognizing these patterns, it becomes easier to predict the behavior of the terms and calculate their totals. Whether dealing with finite or infinite sets, mastering these concepts is key to progressing in many areas of algebra and calculus.
Types of Sequences
A sequence is simply an ordered list of numbers that follow a specific rule or pattern. The two most common types are:
- Arithmetic Sequences: In these sequences, the difference between consecutive terms remains constant. This difference is called the common difference.
- Geometric Sequences: Here, each term is found by multiplying the previous term by a fixed number known as the common ratio.
Summing the Terms
When we sum the terms of a sequence, we get a series. The formula to find the sum depends on the type of sequence:
- Arithmetic Series: The sum of the first n terms of an arithmetic sequence can be calculated using the formula: Sₙ = n/2 * (2a + (n – 1)d), where ‘a’ is the first term, ‘d’ is the common difference, and ‘n’ is the number of terms.
- Geometric Series: The sum of the first n terms of a geometric sequence is given by Sₙ = a * (1 – rⁿ) / (1 – r), where ‘a’ is the first term and ‘r’ is the common ratio (provided that r ≠ 1).
Mastering these sequences and series allows for the efficient calculation of totals in various mathematical contexts, from simple summation problems to more complex applications in calculus and finance.
Preparation for Word Problems
Word problems often require translating real-world situations into mathematical expressions and equations. The key to solving these problems effectively is understanding the context and recognizing the relationships between different variables. By breaking down complex scenarios into manageable steps, it becomes easier to identify the correct approach and apply the appropriate mathematical methods.
Steps for Solving Word Problems
To tackle word problems successfully, follow a systematic approach:
- Understand the Problem: Read the problem carefully and identify the important information. Pay attention to what is being asked and the given values.
- Define Variables: Assign variables to unknown quantities. This makes it easier to set up an equation based on the relationships described in the problem.
- Set Up an Equation: Use the relationships between the variables to form an equation. Often, this involves translating phrases like “total cost” or “rate of change” into mathematical expressions.
- Solve the Equation: Apply the necessary mathematical operations to solve for the unknown variable(s). This may involve algebraic manipulation or using other problem-solving techniques.
- Check Your Answer: Once you find a solution, check it against the context of the problem to ensure it makes sense.
Common Types of Word Problems
Word problems can appear in a variety of contexts, including:
| Type of Problem | Example |
|---|---|
| Linear Equations | Determining the cost of an item based on price per unit |
| Rate and Time | Calculating the time it takes to travel a certain distance at a specific speed |
| Mixture Problems | Finding the final concentration of a solution made from two different concentrations |
By practicing different types of word problems, you will become more proficient in identifying the best methods for solving them and handling various scenarios with confidence.
Practice with Complex Numbers
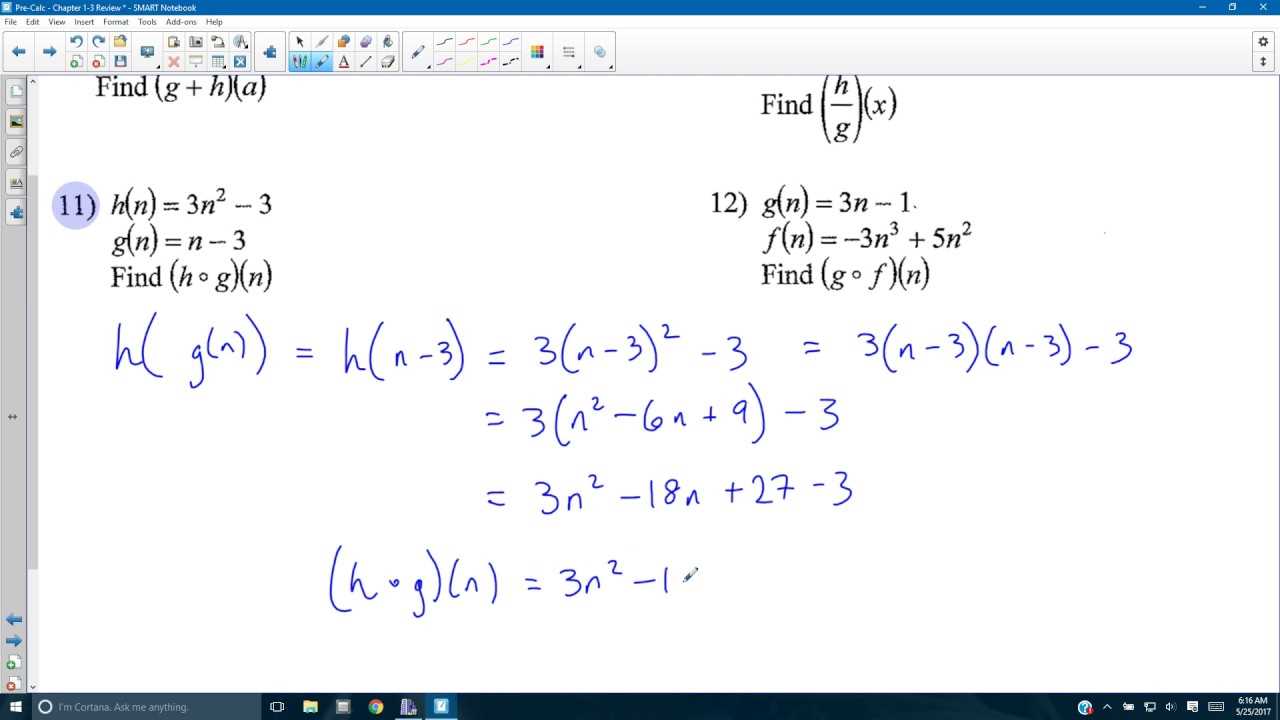
Complex numbers play a crucial role in many mathematical concepts, especially when working with equations that cannot be solved using only real numbers. These numbers consist of a real part and an imaginary part, which allows for the extension of number systems and provides solutions to problems involving square roots of negative numbers. Mastering the operations and properties of complex numbers is essential for advancing in mathematics and solving more challenging problems.
Basic Operations with Complex Numbers
Working with complex numbers involves several key operations, including addition, subtraction, multiplication, and division. These operations follow rules similar to those used with real numbers, with the added consideration of the imaginary unit, denoted as i, where i is the square root of -1.
- Addition: To add two complex numbers, simply add their real parts and their imaginary parts separately. For example, (a + bi) + (c + di) = (a + c) + (b + d)i.
- Subtraction: Subtract the real and imaginary parts separately. For instance, (a + bi) – (c + di) = (a – c) + (b – d)i.
- Multiplication: Multiply complex numbers using the distributive property, remembering that i² = -1. For example, (a + bi) × (c + di) = (ac – bd) + (ad + bc)i.
- Division: To divide complex numbers, multiply both the numerator and the denominator by the conjugate of the denominator. This eliminates the imaginary part from the denominator.
Applications and Further Practice
Complex numbers are not just theoretical; they have real-world applications in fields such as engineering, physics, and signal processing. To develop a deeper understanding, practice problems that involve complex number operations, as well as their use in solving quadratic equations, are highly beneficial.
By continuously practicing these operations and their applications, you will gain confidence and improve your ability to solve more advanced mathematical challenges involving complex numbers.
Calculating Derivatives and Integrals
Understanding the concepts of derivatives and integrals is fundamental to solving many problems in mathematics, especially in calculus. Derivatives help us understand how a function changes at any given point, while integrals are used to determine the accumulated quantity or area under a curve. Both of these operations are vital tools in various fields, including physics, economics, and engineering.
Finding Derivatives
To find the derivative of a function, we apply specific rules that describe how functions behave as their inputs change. These rules include the power rule, product rule, quotient rule, and chain rule, each useful for different types of functions. The derivative can be thought of as the slope of the tangent line to the function at any given point.
- Power Rule: For functions of the form f(x) = x^n, the derivative is f'(x) = nx^(n-1).
- Product Rule: For two functions multiplied together, (f * g)’ = f’ * g + f * g’.
- Quotient Rule: For dividing two functions, (f / g)’ = (f’ * g – f * g’) / g².
- Chain Rule: For composite functions, (f(g(x)))’ = f'(g(x)) * g'(x).
Calculating Integrals
Integrals are used to find the area under a curve or the accumulation of a quantity over an interval. The process of finding an integral is called integration. There are different techniques, such as direct integration, substitution, and integration by parts, depending on the form of the function.
- Power Rule for Integration: For functions of the form f(x) = x^n, the integral is ∫ x^n dx = (x^(n+1)) / (n+1).
- Substitution: This method involves substituting part of the function to simplify the integration process. It is particularly useful when dealing with compositions of functions.
- Integration by Parts: This technique is based on the product rule for derivatives and is used when the function is a product of two simpler functions.
Mastering both derivatives and integrals provides a powerful set of tools for analyzing and solving mathematical problems, especially when dealing with rates of change and accumulated quantities. Practice with a variety of functions will strengthen your understanding and improve your problem-solving abilities in these areas.
Test-Taking Strategies for Success
Achieving success during assessments requires more than just knowledge of the material. The way you approach the task, manage your time, and handle stress can greatly impact your performance. By using effective strategies, you can maximize your efficiency and accuracy when answering questions, ensuring the best possible results.
Time Management
One of the most important skills to master is managing your time wisely. Allocate sufficient time to each section based on its difficulty and the number of points it offers. Start with the questions you feel most confident about to build momentum, and leave more challenging problems for later. Avoid spending too much time on a single question–move on if you get stuck, and return to it once you’ve completed the easier ones.
Understanding the Instructions
Always read the instructions thoroughly before beginning. Misunderstanding the requirements of a question can lead to unnecessary mistakes. Pay close attention to keywords that guide you in how to approach each problem, such as “solve,” “explain,” “simplify,” or “graph.” Clarifying any doubts before you begin can save you valuable time.
Focus on Accuracy
While it’s important to work efficiently, accuracy should never be sacrificed. Take a moment to double-check your answers, especially for calculations and complex reasoning. Small errors can have a large impact, so ensure your work is clean and organized. If possible, review key steps of your process to confirm you haven’t missed anything important.
Managing Stress
Staying calm under pressure is essential for peak performance. Deep breathing exercises or quick relaxation techniques can help reduce anxiety and keep you focused. If you start to feel overwhelmed, pause for a moment, reset, and remind yourself that you’ve prepared for this challenge. Confidence in your abilities will improve your efficiency and clarity in thinking.
By practicing these strategies, you can approach assessments with a sense of control, ensuring that your preparation and efforts translate into successful outcomes. Focused, well-organized work habits will set you up for long-term success in any academic challenge.