Ab Calculus Exam Review Sheet Tips and Key Concepts

Preparing for a high-level mathematics assessment requires a clear understanding of core principles and effective strategies. In this guide, we will explore the fundamental areas you need to focus on to excel in the subject, emphasizing key concepts, techniques, and tools to boost your performance.

Thorough preparation is crucial, as it not only helps reinforce your understanding but also builds your confidence for tackling a wide range of problems. Whether you’re aiming to perfect your skills in differentiation, integration, or understanding complex functions, a focused approach can make all the difference.

We’ll walk you through the most important areas to master, provide tips on managing your time effectively, and highlight common challenges students face. With the right mindset and approach, you can successfully navigate any test with ease and precision.

AB Calculus Exam Review Sheet

Success in advanced mathematics assessments requires a solid grasp of various fundamental topics. Focusing on core principles, such as functions, derivatives, and integrals, will prepare you to tackle even the most complex problems. The key is mastering these concepts so that they become second nature, allowing you to approach any question with confidence and clarity.

To optimize your study process, it’s essential to revisit important formulas and methodologies that frequently appear in testing scenarios. A deep understanding of these techniques will not only help you solve problems faster but also avoid common mistakes. Consistent practice with different problem types enhances both speed and accuracy.

Additionally, understanding how to apply theoretical knowledge to practical situations is crucial. Being able to interpret graphs, solve word problems, and analyze real-world applications will strengthen your overall performance. With the right preparation, you’ll be ready to approach the test with the skills and mindset needed for success.

Key Concepts to Focus On

Mastering certain core ideas is essential for achieving success in this advanced mathematical field. Understanding the foundational principles behind each topic allows you to approach problems from multiple angles and develop a deeper insight into the material. Below are some of the most crucial concepts to focus on during your preparation:

Limits and Continuity: Grasp the behavior of functions as they approach specific points and the conditions for continuous functions.
Derivatives: Learn how to find the rate of change of a function and apply this knowledge to real-world scenarios such as motion and optimization.
Integrals: Understand the process of finding areas under curves and solving problems related to accumulation and total change.
The Fundamental Theorem: Make sure you comprehend how differentiation and integration are connected, and how to apply this relationship in problem-solving.
Related Rates: Develop the skill to solve problems that involve multiple variables changing with respect to time.
Optimization: Focus on how to find maximum and minimum values for various functions, especially in applied contexts.
Sequences and Series: Study the behavior of series, including convergence tests and power series expansions.

Concentrating on these core concepts will build the strong foundation necessary to handle complex questions and improve your problem-solving abilities in the subject. By regularly practicing these topics, you will develop both the speed and precision needed to succeed.

Essential Formulas You Must Memorize

Having a strong command over key formulas is crucial for solving problems efficiently and accurately. By memorizing the most important equations and relationships, you can save valuable time during assessments and ensure that you’re ready for a wide range of questions. Below are the essential formulas that should be at the forefront of your study sessions:

Key Derivative and Integral Formulas

Power Rule: d/dx [x^n] = n * x^(n-1)
Product Rule: d/dx [u(x) * v(x)] = u'(x) * v(x) + u(x) * v'(x)
Quotient Rule: d/dx [u(x) / v(x)] = (v(x) * u'(x) - u(x) * v'(x)) / [v(x)]^2
Chain Rule: d/dx [f(g(x))] = f'(g(x)) * g'(x)
Definite Integral: ∫[a, b] f(x) dx = F(b) - F(a)
Area Under a Curve: ∫[a, b] f(x) dx

Important Theorems and Relationships

The Fundamental Theorem of Calculus: ∫[a, b] f'(x) dx = f(b) - f(a)
Integration by Parts: ∫ u dv = uv - ∫ v du
Mean Value Theorem: f'(c) = [f(b) - f(a)] / (b - a)
Euler’s Method: y(n+1) = y(n) + h * f(x(n), y(n))
Horizontal and Vertical Asymptotes: Find limits as x approaches infinity for horizontal and the limit of the function at undefined points for vertical asymptotes.

Memorizing and understanding these formulas is essential for mastering the subject and applying them to a variety of problems. Regular practice with these equations will not only improve recall but also make the application of these formulas second nature when solving complex problems.

Strategies for Time Management

Efficient use of time is one of the most critical factors for success when tackling a rigorous mathematical test. By organizing your approach, you can maximize your productivity, reduce stress, and ensure you have sufficient time to address all questions thoroughly. Below are some practical strategies to help manage your time effectively during a high-pressure situation:

Strategy	Description
Prioritize Problems	Start with the questions you find easiest, then move on to more complex ones. This helps build momentum and boosts your confidence.
Allocate Time for Each Section	Divide the total time based on the number of questions and difficulty level. Stick to your time allocation and move on when it’s up.
Skip and Return	If a problem is taking too long, skip it and return to it later. Often, other questions can trigger insights for tougher ones.
Work Efficiently, Not Perfectly	Avoid spending excessive time on a single problem. Focus on getting a good enough solution rather than trying to perfect every detail.
Use Calculators Wisely	Leverage calculators for complex computations but avoid over-relying on them. Ensure that they don’t become a time drain.

By following these strategies, you can ensure that you’re not only completing all sections of the test but also doing so with accuracy and efficiency. Time management isn’t just about speed; it’s about finding the right balance between speed and thoughtful problem-solving.

Understanding Limits and Continuity

Grasping the concepts of behavior at specific points and the smoothness of functions is crucial for solving a wide range of mathematical problems. Limits describe how a function behaves as its input approaches a particular value, while continuity ensures that there are no sudden jumps or breaks in the function’s graph. Together, these concepts form the foundation for more advanced techniques and problem-solving approaches.

Limits are used to understand the end behavior of functions and to deal with cases where values might approach infinity or undefined points. Continuity, on the other hand, ensures that functions are well-behaved throughout their domain, which is vital when solving real-world problems where stability and predictability are key.

When approaching limits, focus on evaluating the behavior of functions as they approach a specific point or infinity. In the case of continuity, ensure that the function’s value at a given point matches its limit at that point and that there are no gaps in the function. A function is continuous if it is defined at a point, its limit exists at that point, and the function’s value equals the limit.

Mastering Derivatives and Their Applications

Understanding the rate of change of functions is central to solving a variety of mathematical problems. By mastering derivatives, you gain the ability to analyze how functions behave and how they change in response to input variations. This concept is not only foundational for solving mathematical problems but also has practical applications in fields like physics, economics, and engineering.

To effectively apply derivatives, it’s essential to familiarize yourself with the various rules and techniques for finding them. These include:

Power Rule: Quickly find derivatives of polynomial functions.
Product and Quotient Rules: Handle the differentiation of products or quotients of functions.
Chain Rule: Differentiate composite functions efficiently.
Implicit Differentiation: Solve equations where the function is not explicitly given.

Once you have a strong grasp of how to compute derivatives, it’s important to apply this knowledge to real-world situations. Some common applications include:

Optimization: Use derivatives to find maximum or minimum values of functions, such as minimizing costs or maximizing profit.
Motion Analysis: Derivatives are used to analyze the velocity and acceleration of moving objects.
Linear Approximation: Estimate values of functions near a specific point using the tangent line.

By practicing these techniques and their applications, you will develop a deeper understanding of how functions change and how to solve problems involving rates of change. Mastery of derivatives will enhance your problem-solving skills and provide you with the tools needed for more advanced mathematical concepts.

Integration Techniques to Know

Mastering integration is essential for solving problems related to areas, volumes, and accumulation. While the process of integration can often seem complex, there are several key methods and techniques that simplify the task. By familiarizing yourself with these approaches, you can tackle a wide variety of problems with confidence and precision.

Basic Integration Methods

Before diving into more advanced techniques, it’s important to be comfortable with basic integration rules. These include:

Power Rule: Integrating powers of x, such as ∫x^n dx = (x^(n+1))/(n+1), is a fundamental skill.
Basic Exponential and Logarithmic Integrals: Be sure to remember key formulas like ∫e^x dx = e^x and ∫1/x dx = ln|x|.
Constant Multiplication: Recognize that constants can be factored out of integrals: ∫a * f(x) dx = a ∫f(x) dx.

Advanced Integration Techniques

Once you’ve mastered the basics, you can apply more advanced methods to tackle challenging integrals:

Integration by Parts: This technique, derived from the product rule of differentiation, is useful for integrating products of functions. The formula is: ∫u dv = uv - ∫v du.
Substitution: A powerful method for simplifying integrals by substituting a part of the integrand with a new variable. This is particularly useful for compositions of functions.
Partial Fractions: Decompose rational functions into simpler fractions that are easier to integrate. This method is especially helpful for integrating rational expressions.
Trigonometric Integrals: Use identities to simplify integrals involving trigonometric functions, such as ∫sin^2(x) dx or ∫cos(x) sin(x) dx.

By practicing these methods, you will gain the skills necessary to solve a wide range of integration problems, from basic to advanced. A thorough understanding of these techniques will not only enhance your ability to compute integrals but also improve your overall problem-solving abilities in mathematics.

Important Theorems and Their Usage

In advanced mathematics, theorems play a crucial role in establishing foundational principles that help solve complex problems. Understanding key theorems and knowing how to apply them is essential for addressing a wide variety of mathematical scenarios. These theorems are not only fundamental to solving theoretical problems but also provide practical tools for real-world applications in science and engineering.

Key Theorems and Their Applications

Below are some essential theorems and how they can be used in different contexts:

Theorem	Usage
Fundamental Theorem of Calculus	Links the concept of differentiation with integration. It allows the evaluation of definite integrals and helps in finding antiderivatives efficiently.
Mean Value Theorem	Establishes that for any continuous function over a closed interval, there is at least one point where the instantaneous rate of change equals the average rate of change over the interval.
Intermediate Value Theorem	States that if a function is continuous on a closed interval and takes opposite signs at the endpoints, there exists a point in the interval where the function equals zero.
Rolle’s Theorem	Is a special case of the Mean Value Theorem. It asserts that if a function is continuous on a closed interval and differentiable on the open interval, and the function has equal values at the endpoints, then there is at least one point where its derivative is zero.

Applications in Problem Solving

Understanding how to apply these theorems is crucial for tackling various types of mathematical problems. For instance:

Optimization Problems: The Mean Value Theorem and the Fundamental Theorem of Calculus are invaluable in finding maximum or minimum values in real-world optimization problems.
Root-Finding Problems: The Intermediate Value Theorem is often used to prove the existence of roots within a given interval.
Curve Analysis: Rolle’s Theorem and the Mean Value Theorem help in understanding the behavior of curves, particularly in determining where a function’s slope is zero.

By mastering these theorems and their applications, you can solve a wide variety of problems more effectively and gain a deeper understanding of mathematical concepts.

Practice Problems for Differentiation

To strengthen your understanding of how functions change with respect to their variables, it is essential to practice applying differentiation techniques. By solving problems that involve finding the rate of change or slope of various types of functions, you can build your confidence and improve your problem-solving skills. The following problems cover a range of differentiation methods, including basic rules, product and quotient rules, and the chain rule.

Basic Differentiation Problems

Start with fundamental exercises to ensure that you are comfortable with basic differentiation rules:

Differentiate the function f(x) = 3x^4 - 5x^3 + 2x - 7.
Find the derivative of g(x) = 4e^x - 2x^2 + 5.
Differentiate the following: h(x) = sin(x) + cos(x).

Advanced Differentiation Problems

Once you’re comfortable with basic problems, move on to more complex scenarios that require the application of additional rules:

Use the product rule to differentiate f(x) = (2x^3 + 3x)(x^2 - 1).
Apply the quotient rule to differentiate g(x) = (5x^2 + 3) / (x - 2).
Differentiate the composite function h(x) = sin(3x^2 + 1) using the chain rule.

By working through these practice problems, you’ll not only strengthen your understanding of differentiation techniques but also enhance your ability to apply these methods to solve more advanced mathematical challenges.

How to Tackle Complex Integration Problems

When faced with intricate integration problems, it can be helpful to break them down into simpler parts and apply a systematic approach. By recognizing patterns, applying appropriate techniques, and remaining patient, you can efficiently solve even the most challenging integrals. This section outlines strategies to tackle complex problems, covering a range of methods that can simplify the process and help you achieve accurate results.

Steps to Approach Complex Integrals

Follow these key steps to effectively solve complex integration problems:

Identify the type of integral: Before starting, determine if the integral fits a basic form or if more advanced methods are needed, such as substitution, integration by parts, or partial fractions.
Look for patterns: Recognize common patterns such as trigonometric identities, rational expressions, or exponential functions that can simplify the integrand.
Choose the right technique: Depending on the structure of the integrand, apply the appropriate method. For example, use substitution for composite functions, integration by parts for products of functions, or partial fractions for rational functions.
Break the integral into simpler parts: For more complicated integrals, split the expression into smaller, more manageable components that can be integrated separately and then combined.

Common Techniques for Complex Problems

Several methods are especially useful when dealing with complex integrals:

Substitution: If the integral involves a composition of functions, use substitution to simplify the integral. For example, let u = g(x) and transform the integral into a more straightforward form.
Integration by Parts: This method is helpful when integrating products of functions. The formula ∫u dv = uv - ∫v du allows you to break the problem into smaller parts.
Partial Fractions: For rational functions, decompose the integrand into simpler fractions that are easier to integrate. This is especially effective for integrals involving polynomials in the numerator and denominator.
Trigonometric Substitution: If the integral involves square roots of quadratic expressions, trigonometric substitution can simplify the problem. For example, use x = tan(θ) to handle integrals involving √(a^2 + x^2).

By practicing these strategies and techniques, you can develop a systematic approach to solving complex integration problems with greater ease and accuracy. Over time, these methods will become second nature, allowing you to tackle even the most difficult integrals with confidence.

Common Pitfalls to Avoid During the Exam

When tackling a challenging assessment, it’s easy to make simple mistakes under pressure. Being aware of common errors and knowing how to avoid them can help ensure that you approach problems more effectively. This section highlights some frequent pitfalls students encounter and offers tips to steer clear of them, so you can perform at your best.

Misunderstanding the Problem

One of the most common mistakes is misinterpreting the problem or failing to fully understand what is being asked. Take time to read each question carefully and identify the key elements before you begin solving. Look out for words like “at,” “from,” or “to” that may change the way you approach the solution.

Tip: Underline or highlight important information, such as initial conditions, boundaries, or restrictions.
Tip: Break complex problems into smaller parts to avoid overlooking critical details.

Rushing Through Calculations

It’s easy to get caught up in time pressure, but rushing through calculations can lead to avoidable mistakes. Ensure that you double-check your work as you go along, especially when performing multiple steps or using formulas.

Tip: Write each step clearly and leave enough space to recheck your work.
Tip: Always verify your final answers by plugging them back into the original problem whenever possible.

Neglecting to Check for Simple Errors

Small errors, such as sign mistakes or missing parentheses, can snowball into bigger problems. These are easy to overlook, especially in lengthy problems, but they can drastically change your answer.

Tip: After finishing a problem, take a moment to carefully review your steps, particularly the calculations.
Tip: Pay extra attention to negative signs and exponents, which are common sources of error.

Overcomplicating the Problem

Another frequent mistake is overthinking the problem or applying complex methods when a simpler approach would suffice. While it’s important to demonstrate your understanding, it’s also essential to recognize when a straightforward method will yield the correct result.

Tip: Simplify the problem by identifying patterns or symmetries that could help you solve it more efficiently.
Tip: Don’t be afraid to use basic methods, such as factoring or basic trigonometric identities, to simplify complex expressions.

Forgetting Units or Constraints

Forgetting to include units or failing to respect given constraints can result in incomplete or incorrect answers. Make sure that all conditions, whether units or restrictions, are included in your final answer.

Tip: Double-check if the problem specifies units, and ensure you provide your answer in the correct format.
Tip: If the problem involves inequalities or limits, ensure that you respect those conditions in your solution.

By avoiding these common pitfalls and following these simple tips, you can enhance your performance and tackle problems with more confidence. Practice and careful attention to detail will help minimize mistakes, ensuring that you stay on track throughout the assessment.

Tips for Multivariable Calculus Questions

When tackling problems involving more than one variable, the complexity can increase significantly. These types of questions require a solid understanding of how to manage and manipulate multiple components at once. To efficiently solve these challenges, it’s crucial to use the right approach, break down each problem step by step, and apply the necessary concepts with confidence.

Visualize the Problem Geometrically

One of the first steps in handling multi-variable questions is to visualize the problem in a geometric context. Many of these problems represent physical quantities, such as surfaces or volumes, and understanding the spatial relationships can offer valuable insight into how to proceed.

Tip: Always sketch the problem or visualize the surfaces and curves involved to make sense of the problem’s context.
Tip: Recognize common geometric shapes like spheres, cylinders, or planes, as they simplify calculations and guide your approach.

Focus on Partial Derivatives

Partial derivatives are a fundamental tool when working with functions that depend on multiple variables. Knowing how to compute and apply partial derivatives will allow you to isolate the effect of each individual variable on the overall function.

Tip: Practice taking partial derivatives for functions with more than one variable, keeping the other variables constant.
Tip: Keep track of the order in which you differentiate to avoid errors, especially with complex expressions.

Use the Chain Rule Wisely

The chain rule is a critical technique for differentiating composite functions. When multiple variables are involved, understanding how to apply the chain rule in these contexts is vital for solving complicated problems.

Tip: Be comfortable with multi-variable chain rules to differentiate nested functions involving several variables.
Tip: Pay attention to how each variable influences the overall function when using the chain rule in multi-dimensional settings.

Master Multiple Integrals

In problems involving areas, volumes, or other quantities, integrating over multiple variables is a key concept. Mastering double and triple integrals, as well as knowing how to change between coordinate systems, will help you handle these problems with ease.

Tip: Practice setting up and evaluating integrals in Cartesian, polar, and spherical coordinates to tackle a variety of problems.
Tip: Understand how to change the order of integration to simplify calculations or make them more manageable.

Understand Vector Fields and Flux

Vector fields, which represent quantities like velocity or force in multi-dimensional spaces, are often integral to multi-variable problems. Being able to compute and interpret divergence, curl, and flux is crucial for solving these problems effectively.

Tip: Familiarize yourself with how to compute divergence and curl for vector fields, especially in different coordinate systems.
Tip: Understand how the Divergence Theorem and Stokes’ Theorem are used to relate integrals of vector fields to surface and volume integrals.

By keeping these strategies in mind and consistently practicing multi-variable problems, you’ll develop a deeper understanding of the concepts and gain confidence in solving these types of questions effectively.

Using Graphing Calculators Effectively

Graphing calculators are powerful tools that can greatly enhance your ability to solve complex problems. These devices allow you to visualize equations, analyze data, and perform calculations that would be time-consuming by hand. However, to get the most out of them, it’s important to use the calculator effectively, understanding both its features and limitations.

Familiarize Yourself with Key Features

Before relying on a graphing calculator during any problem-solving process, make sure you understand its essential functions. These include graphing equations, computing derivatives, performing integrals, and solving systems of equations. Familiarity with these capabilities will save time and help you apply the right tool at the right moment.

Tip: Learn how to input and manipulate equations in the calculator’s interface to ensure accurate results.
Tip: Practice graphing various functions to visualize their behavior across different ranges of values.

Use the Zoom and Window Features for Better Accuracy

One of the key advantages of graphing calculators is their ability to zoom in on specific regions of a graph. This can help you identify key features such as intercepts, turning points, and asymptotes with greater precision. Additionally, adjusting the window settings allows you to focus on the most relevant parts of a graph, ensuring you are working with the most accurate data.

Tip: Adjust the window settings before graphing to ensure the graph fits within a meaningful domain and range.
Tip: Zoom in on specific sections of a graph to examine areas of interest more closely, especially when dealing with complex curves.

Double-Check Solutions Using Multiple Methods

While graphing calculators can provide quick answers, it’s important to verify those answers by using multiple methods when possible. This ensures that you aren’t relying solely on the calculator’s output, but are also building a deeper understanding of the problem and its solution.

Tip: After finding a solution using the calculator, attempt to solve the problem manually or use an alternative approach for confirmation.
Tip: Use the calculator’s table feature to cross-check values or find solutions to specific problems.

By mastering these techniques and using your graphing calculator as a supportive tool rather than a crutch, you’ll enhance your problem-solving skills and improve your efficiency in tackling more difficult tasks.

Common Mistakes in AP Calculus Exams

When preparing for advanced mathematics tests, students often make several critical mistakes that can impact their performance. These errors typically arise from a lack of attention to detail, poor time management, or misunderstanding key concepts. Recognizing and addressing these pitfalls can significantly improve your chances of success.

Misinterpreting Questions

One of the most common mistakes is misinterpreting the questions, especially when they involve multiple steps or require specific techniques. It’s easy to rush through the wording and miss crucial information that affects the approach to solving the problem. Read each question carefully and underline key terms or phrases that indicate what needs to be done.

Tip: Break down the problem step by step to ensure you understand what is being asked before proceeding.
Tip: Pay attention to words like “find,” “verify,” “simplify,” and “evaluate” to help guide your solution process.

Incorrect Application of Rules and Formulas

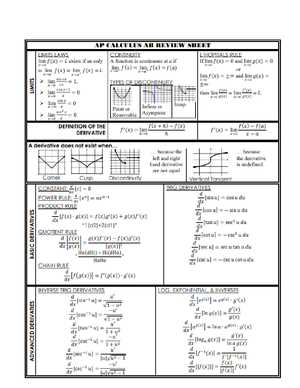
Another common issue is applying the wrong formula or rule to a problem. This often occurs when students are under pressure and mistakenly use a technique that doesn’t fit the situation. It’s essential to have a clear understanding of when and how to apply each mathematical rule.

Tip: Take a moment to recall the specific conditions under which each formula or rule is valid before applying it.
Tip: Review common techniques such as the chain rule, product rule, and integration by parts to ensure you’re using the correct one for the problem.

By staying focused and careful, you can avoid these mistakes and approach each problem with confidence. Thorough preparation, practice, and understanding of the material are key to overcoming these challenges and achieving success on your tests.

How to Approach Word Problems

Word problems often present challenges due to the need to translate real-world situations into mathematical expressions. The key to solving these problems is breaking them down step by step and understanding how the information given relates to the concepts you’ve learned. A structured approach can help you navigate through the complexity and find the solution effectively.

Start by Understanding the Problem

Before jumping into calculations, take the time to carefully read the problem. Identify what is being asked and the data you are provided with. Highlight or underline important numbers, units, and key terms that will guide your solution process. Understanding the context of the problem helps ensure that you approach it correctly.

Translate Words into Equations

Next, translate the given information into mathematical expressions. This is where many students struggle, as they might not know which formula or concept applies. Look for clues such as rates, distances, areas, or volumes to determine the appropriate approach. Writing out the equation that models the situation is crucial for solving the problem correctly.

Tip: Create variables to represent unknowns and use them to form equations.
Tip: Pay attention to relationships between quantities, such as “per hour,” “per unit,” or “total,” which can help you set up proportional relationships.

Break the Problem into Manageable Steps

Once you have your equations, solve them systematically. Avoid trying to tackle everything at once. Break down the problem into smaller, more manageable steps, solving each one before moving on to the next. If necessary, solve intermediate equations to isolate unknowns and gradually build up to the final answer.

Tip: Keep track of units to avoid errors and ensure that the final answer is in the correct form.
Tip: Double-check your work at each step to make sure the math is correct and that it aligns with the problem’s context.

Review Your Solution

Finally, once you’ve solved the problem, review your solution to make sure it makes sense within the context of the question. Does your answer fit the situation described in the problem? Are the units consistent? If something seems off, retrace your steps to identify any mistakes.

Approaching word problems with this structured method will help you tackle even the most complex scenarios confidently and correctly. Practice will also improve your ability to identify the most effective strategies for solving different types of problems.

Reviewing Past Exam Papers

One of the most effective ways to prepare for a test is by going through previous assessments. Analyzing past papers helps you understand the format, identify commonly tested topics, and spot areas where you may need more practice. It also provides insight into the types of problems you might encounter, allowing you to develop strategies for solving them efficiently.

By working through past assessments, you can gain familiarity with the question styles and learn how to allocate your time during the test. Additionally, reviewing past work allows you to pinpoint any recurring themes or concepts that you need to revisit. This process can significantly boost your confidence and improve your overall test-taking skills.

Identify Key Patterns and Topics

Start by examining the types of questions that frequently appear. Some topics might be tested more often, indicating that they are particularly important. Focus on these areas, ensuring you understand the key concepts and how to apply them in different scenarios. This targeted preparation helps you manage your study time more effectively and avoid wasting effort on less critical material.

Practice Under Test Conditions

Once you’ve reviewed the material and identified key topics, simulate real testing conditions by timing yourself while working through past papers. This exercise helps you improve your time management and reduces anxiety on the actual day. It also lets you evaluate how well you can recall information and apply it under pressure.

Analyze Mistakes and Learn From Them

After completing past assessments, carefully review any mistakes you made. Understanding why you got something wrong is crucial for improvement. Did you misinterpret the question? Did you overlook a crucial step? Reflecting on these errors helps prevent them from happening again. This process of self-assessment is key to refining your approach and strengthening your understanding of challenging concepts.

Incorporating the practice of reviewing past assessments into your preparation routine is a powerful way to enhance your readiness. By becoming familiar with the test format, reinforcing your knowledge of essential topics, and learning from past mistakes, you’ll be well-equipped to succeed in your upcoming challenges.

How to Stay Calm on Test Day

The key to performing well during an important assessment is maintaining a calm and focused mindset. Anxiety can cloud your thinking, making it harder to recall information and apply concepts effectively. Preparing both mentally and physically in the days leading up to the test can help you approach the situation with confidence and composure. Staying calm doesn’t mean being passive; it’s about developing a sense of control and confidence that allows you to think clearly when it matters most.

On the day of the test, take steps to manage any stress or nervousness. With the right strategies, you can create a sense of calm that enables you to perform at your best. Here are a few tips that can help you stay composed and focused during the process:

1. Practice Deep Breathing

When nerves start to creep in, pause and take a few slow, deep breaths. Deep breathing helps activate the body’s relaxation response, reducing the physical symptoms of stress such as a racing heart or shallow breathing. This simple technique can quickly calm your mind and restore your focus.

2. Get Enough Rest the Night Before

A good night’s sleep is crucial for clear thinking and optimal performance. Lack of sleep can impair memory, concentration, and decision-making skills. Try to rest the night before the assessment, and avoid staying up late cramming information. Your mind needs time to relax and recharge, so aim for 7-8 hours of sleep before the day.

3. Stay Organized and Prepared

Being well-prepared for the day ahead can significantly reduce stress. Make sure you have all necessary materials (such as pens, ID, calculator, etc.) ready the night before, so you don’t have to rush in the morning. Knowing that you’re fully prepared will give you a sense of control and help ease any pre-test anxiety.

4. Stay Positive and Focused

A positive mindset can go a long way in helping you stay calm. Avoid focusing on what could go wrong; instead, remind yourself of the hard work you’ve done and trust in your abilities. Reassure yourself that you are capable of handling whatever comes your way during the test. Stay present and focus on one question at a time.

5. Manage Your Time Effectively

Time management is an essential part of staying calm during a test. If you find yourself stuck on a question, don’t dwell on it for too long. Move on to the next question and come back to the challenging one later if time permits. By keeping a steady pace, you’ll avoid feeling rushed or overwhelmed as you progress through the assessment.

Remember, staying calm is not just about controlling stress–it’s about setting yourself up for success with the right mindset and strategies. By practicing relaxation techniques, staying organized, and focusing on the positive, you’ll be able to approach the test with clarity and confidence.