Mathematical challenges can often seem daunting, but with the right approach, they become manageable and even enjoyable. The path to mastering complex problems involves understanding the fundamental principles and applying them step by step. This section offers valuable insights into how to tackle various exercises, helping to improve your skills and build confidence.
Breaking down each problem into smaller parts allows for clearer understanding and more effective solutions. By focusing on key concepts and practicing regularly, you’ll develop a deeper grasp of the material. Overcoming common obstacles and avoiding typical mistakes will further enhance your ability to solve problems with precision.
Whether you’re looking to strengthen your foundations or refine advanced techniques, this guide will support your learning journey. With focused practice and the right resources, you can tackle any challenge that comes your way, turning theory into practice and building your mathematical proficiency.
Mathematical Problem Solutions
When tackling mathematical exercises, it’s essential to approach each problem with a clear strategy. Understanding the steps involved and checking your work ensures that you are on the right track. In this section, we explore how to find the correct methods for solving various challenges and verify the outcomes effectively.
Key steps to solving problems:
- Carefully read each question to understand its requirements.
- Identify the type of problem and the methods needed to solve it.
- Work through each step methodically, checking for accuracy as you go.
- Verify your final solution by reviewing the steps and considering alternative approaches.
Common challenges students face:
- Difficulty in applying concepts to new problems.
- Making errors in algebraic manipulation or function interpretation.
- Not fully understanding the logic behind formulas or theorems.
By practicing regularly and following these steps, you can improve your problem-solving abilities and gain a stronger understanding of complex mathematical topics. The more you practice, the more confident you’ll become in your ability to approach problems systematically and accurately.
Understanding Key Mathematical Concepts

Grasping fundamental mathematical ideas is crucial for solving complex problems effectively. Whether you’re working with functions, equations, or graphing techniques, understanding the core principles is the first step toward success. This section delves into essential topics and strategies that lay the foundation for more advanced study.
Essential concepts to focus on:
- Understanding the relationship between variables and how they interact within equations.
- Mastering different types of functions and their properties, such as linear, quadratic, and exponential functions.
- Recognizing the importance of algebraic manipulation in solving problems and simplifying expressions.
Strategies to reinforce these concepts:
- Practice breaking down complex expressions into simpler parts to build a better understanding.
- Work on visualizing functions and equations through graphs to see how variables change and interact.
- Test your understanding with real-world problems to strengthen your problem-solving skills.
By mastering these fundamental concepts and techniques, you’ll be better equipped to handle more advanced challenges and improve your overall mathematical abilities. Consistent practice and a clear understanding of the basics will set the stage for continued progress in your studies.
How to Solve Complex Equations
Solving intricate mathematical equations requires a clear understanding of the underlying principles and a methodical approach. By breaking down the problem step by step, you can systematically solve for the unknowns. This section covers effective techniques and strategies to approach even the most challenging equations.
Step-by-Step Problem Solving
The key to solving complicated equations lies in carefully simplifying and isolating terms. Start by organizing the equation, ensuring that all like terms are combined, and look for patterns that may simplify your work. Depending on the type of equation, use appropriate methods such as:
- Factoring the equation to break it down into smaller, more manageable parts.
- Applying substitution or elimination methods to solve systems of equations.
- Using logarithms or exponents to simplify expressions involving powers or roots.
Common Techniques for Solving Complex Problems

Algebraic Manipulation: Rearranging terms and applying algebraic rules are essential steps when solving equations. Always check for opportunities to factor or cancel terms early in the process.
Graphing for Visualization: For some equations, especially those involving functions, graphing can provide insight into the behavior of the equation and help identify solutions more intuitively.
By following these techniques and practicing consistently, you’ll build confidence in tackling even the most difficult equations. The key is to approach each problem systematically, check your work along the way, and refine your strategies with practice.
Step-by-Step Approach to Problems
Solving complex problems becomes much more manageable when broken down into smaller, logical steps. By following a systematic approach, you ensure that no part of the problem is overlooked and that every step is handled correctly. This method not only improves accuracy but also builds confidence as you progress through each phase of the solution.
Breaking the process into clear stages:
| Step | Action | Purpose |
|---|---|---|
| 1 | Read the problem carefully | Understand what is being asked and identify key information. |
| 2 | Simplify the equation | Combine like terms, eliminate unnecessary elements, and make the problem easier to handle. |
| 3 | Choose a solution method | Select an appropriate method (e.g., factoring, substitution, graphing) based on the problem type. |
| 4 | Perform the calculations | Work through the chosen method step by step, showing all necessary steps clearly. |
| 5 | Check the solution | Verify the result by substituting back into the original equation or considering logical consistency. |
By consistently following this process, you will be able to approach a wide range of problems with confidence and clarity. Whether you’re solving algebraic equations or more advanced mathematical challenges, a structured approach ensures that you understand each part of the solution and arrive at the correct answer.
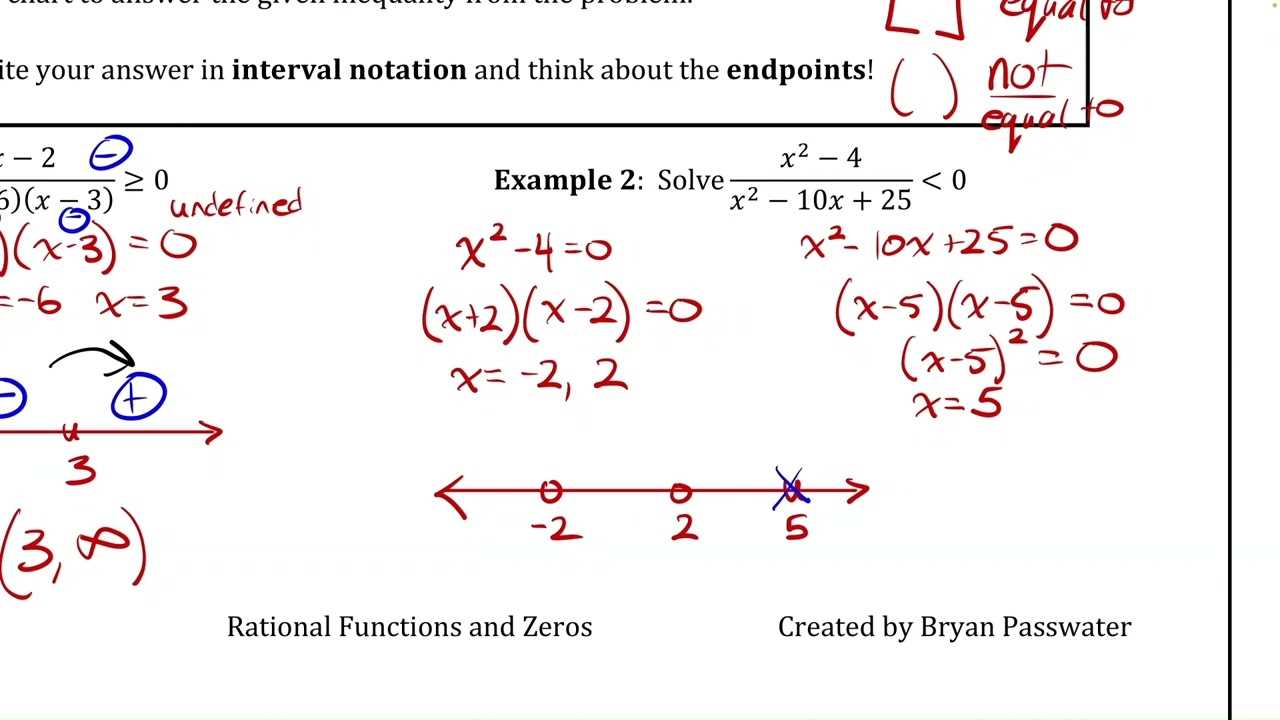
Common Mistakes in Mathematical Exercises
When working through mathematical challenges, it’s easy to make mistakes that can lead to incorrect results. These errors often stem from misunderstandings of core concepts or simple miscalculations. Identifying and avoiding these common pitfalls will improve your accuracy and help you solve problems more efficiently.
Frequent errors to watch out for:
- Misunderstanding the problem: Failing to carefully read the problem or misinterpreting the given information can result in solving for the wrong variable or applying the wrong method.
- Incorrectly simplifying expressions: Combining like terms or factoring expressions incorrectly can lead to a flawed solution. Always double-check your simplifications to avoid errors.
- Forgetting to distribute: Omitting necessary distribution of terms, especially when working with parentheses, can cause major missteps in your calculations.
- Sign errors: One of the most common mistakes is losing track of positive and negative signs, which can drastically change the outcome of the solution.
- Rushing through steps: Trying to solve problems too quickly without paying attention to each individual step often leads to simple arithmetic mistakes or overlooked details.
Tips to avoid mistakes:
- Always read the problem carefully and identify key information before starting the solution process.
- Work through each step methodically, double-checking for errors as you go.
- When simplifying expressions, recheck your calculations for consistency and correctness.
- Take your time to avoid rushing through the process and make sure every step is clear and accurate.
By being mindful of these common mistakes and applying strategies to avoid them, you can significantly improve your problem-solving skills and achieve better results in mathematical exercises.
Effective Strategies for Practice
Consistent practice is essential for mastering mathematical concepts and improving problem-solving skills. However, it’s not just about solving a large number of problems–it’s about practicing effectively and focusing on the right areas. Implementing targeted strategies during your study sessions will help solidify your understanding and boost your confidence.
Key strategies to enhance your practice:
- Focus on foundational concepts: Before diving into complex problems, make sure you have a solid understanding of the basic principles. Mastering the fundamentals makes it easier to approach more advanced material.
- Break down difficult problems: Instead of tackling complex exercises in one go, break them into smaller, manageable parts. This makes the process less overwhelming and helps you focus on each step.
- Practice regularly: Set aside dedicated time each day or week to work through problems. Consistency is key to building fluency and retaining knowledge.
- Review mistakes: After completing practice problems, review any mistakes you made. Understanding where you went wrong helps identify areas for improvement and prevents similar errors in the future.
- Work on diverse problem types: Don’t limit yourself to a single type of problem. The more varied your practice, the better prepared you’ll be for any challenges that come your way.
Additional tips:
- Seek explanations for difficult problems: When you encounter a challenging concept, find resources that provide clear explanations. Visual aids, such as graphs and diagrams, can also be very helpful.
- Simulate test conditions: To build exam readiness, try practicing under timed conditions to mimic the pressure of a real test.
- Stay patient and persistent: Improvement takes time. Don’t get discouraged by mistakes; instead, view them as opportunities to learn and grow.
By following these strategies and maintaining a disciplined approach to practice, you will see significant progress in your mathematical abilities. The more focused and intentional your practice, the greater the payoff in your understanding and problem-solving skills.
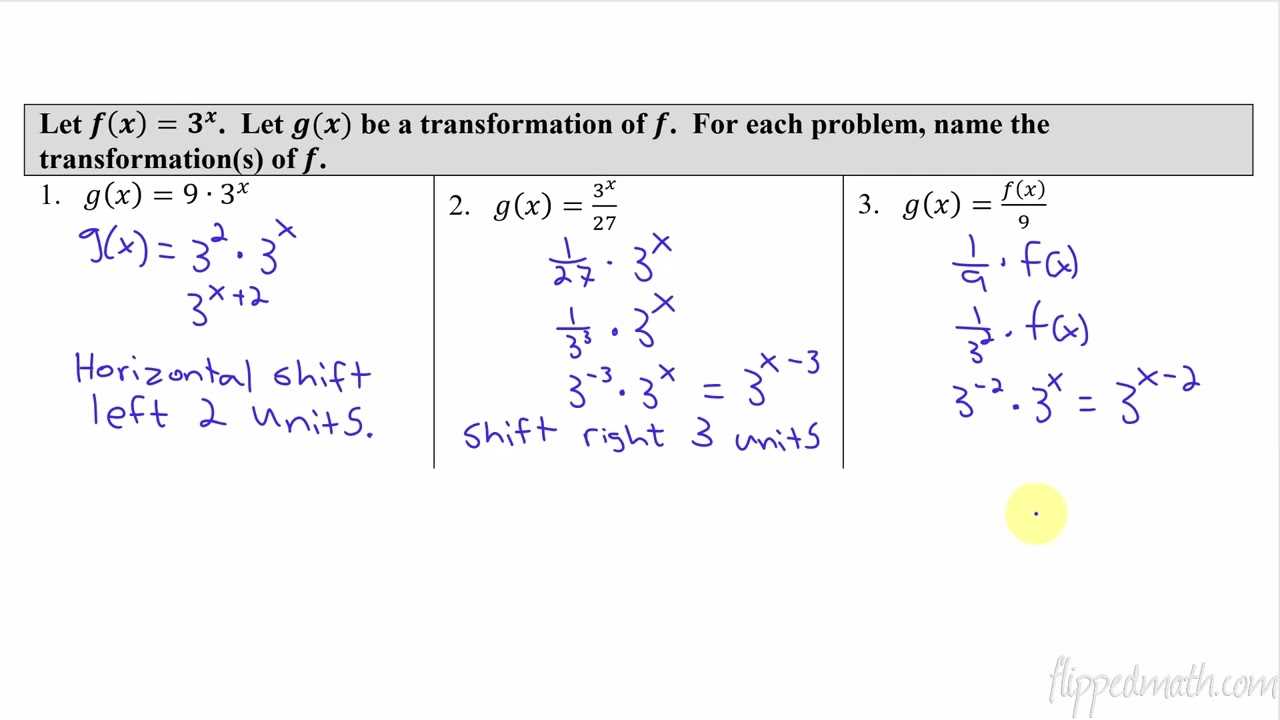
Using Graphing Tools in Solutions
Graphing tools are invaluable when solving mathematical problems, particularly those that involve relationships between variables. These tools allow you to visualize complex equations, making it easier to identify patterns, solutions, and behaviors that might not be immediately apparent through algebraic manipulation alone.
Benefits of Using Graphing Tools
Graphing provides several advantages when tackling mathematical exercises. By plotting equations and inequalities, you can observe the shape and intersections of functions, which helps in determining solutions and understanding their implications. Some key benefits include:
- Visualizing function behavior: Graphs allow you to see how a function behaves over a given range, making it easier to identify trends and key features such as maxima, minima, and intercepts.
- Identifying intersections: When solving systems of equations, graphs can help you pinpoint the exact point(s) where the equations intersect, which corresponds to the solution(s).
- Improving problem-solving speed: By providing a visual representation of the problem, graphing tools help you quickly assess the situation and narrow down possible solutions.
How to Use Graphing Tools Effectively
To make the most of graphing tools, it’s important to use them strategically. Start by accurately plotting the equation or system of equations, making sure that you have the correct scale and axis labels. Once plotted, look for:
- Intercepts: Where the graph crosses the x-axis and y-axis, which correspond to solutions or key points of the equation.
- Slopes and curvature: The steepness and shape of the graph can give you insight into the rate of change and behavior of the equation.
- Symmetry: Some functions exhibit symmetry, which can simplify your analysis and help identify solutions more quickly.
By incorporating graphing tools into your problem-solving approach, you can gain a deeper understanding of the relationships within the equations and improve the accuracy of your solutions. Whether you are working with linear functions, quadratic equations, or more complex relationships, graphing is a powerful method for enhancing your mathematical analysis.
Interpreting Graphs and Functions
Understanding how to read and interpret graphs is a critical skill in mathematics. Graphs provide a visual representation of relationships between variables, making it easier to identify trends, key points, and behaviors that might not be immediately obvious from equations alone. By analyzing graphs, you can uncover valuable insights about the function’s behavior and solve problems more effectively.
Key aspects to focus on when interpreting graphs:
- Identifying intercepts: The points where the graph crosses the axes provide important information. The x-intercept shows where the value of the function is zero, and the y-intercept indicates where the function crosses the vertical axis.
- Analyzing slope and curvature: The steepness and curvature of the graph can reveal how the function behaves as the input values change. A steep slope typically indicates rapid changes, while a flatter curve suggests slower growth or decline.
- Understanding asymptotes: Some graphs approach certain lines but never actually touch them. These are called asymptotes and can provide insights into the function’s limits or boundaries as it extends towards infinity.
- Recognizing symmetry: Many functions exhibit symmetry, such as being even or odd, which can simplify problem-solving and help predict the function’s behavior in different intervals.
By focusing on these elements, you can gain a clearer understanding of the function’s characteristics and how it behaves across different values. Interpreting graphs accurately enables you to solve complex problems more efficiently and helps you visualize the underlying patterns in mathematical relationships.
Trigonometry Tips for Success
Mastering trigonometry requires a solid understanding of key concepts and the ability to apply them to a variety of problems. By focusing on essential techniques and strategies, you can improve your problem-solving skills and build a deeper understanding of trigonometric relationships. These tips will help you tackle challenges more efficiently and confidently.
Key tips for excelling in trigonometry:
- Know the fundamental ratios: Understand the basic trigonometric ratios–sine, cosine, and tangent–as they form the foundation of most trigonometric calculations. These ratios relate the sides of a right triangle to its angles and are essential for solving problems.
- Use unit circles: The unit circle is a powerful tool for understanding trigonometric functions, especially when dealing with angles outside of right triangles. Familiarize yourself with the unit circle to gain a deeper insight into sine, cosine, and tangent at various angles.
- Memorize key identities: Trigonometric identities, such as the Pythagorean identity and angle sum and difference formulas, are essential for simplifying and solving more complex problems. Memorizing these identities can save time and effort during calculations.
- Practice regularly: Like any other mathematical concept, practice is crucial for mastering trigonometry. Solve a variety of problems to strengthen your understanding and improve your problem-solving speed and accuracy.
- Visualize problems: Whenever possible, draw diagrams or graphs to visualize the trigonometric relationships. This can help you better understand the problem and identify the best approach for finding a solution.
By incorporating these strategies into your study routine, you’ll enhance your ability to approach and solve trigonometric problems with confidence. With practice and persistence, trigonometry will become a more intuitive and manageable topic to master.
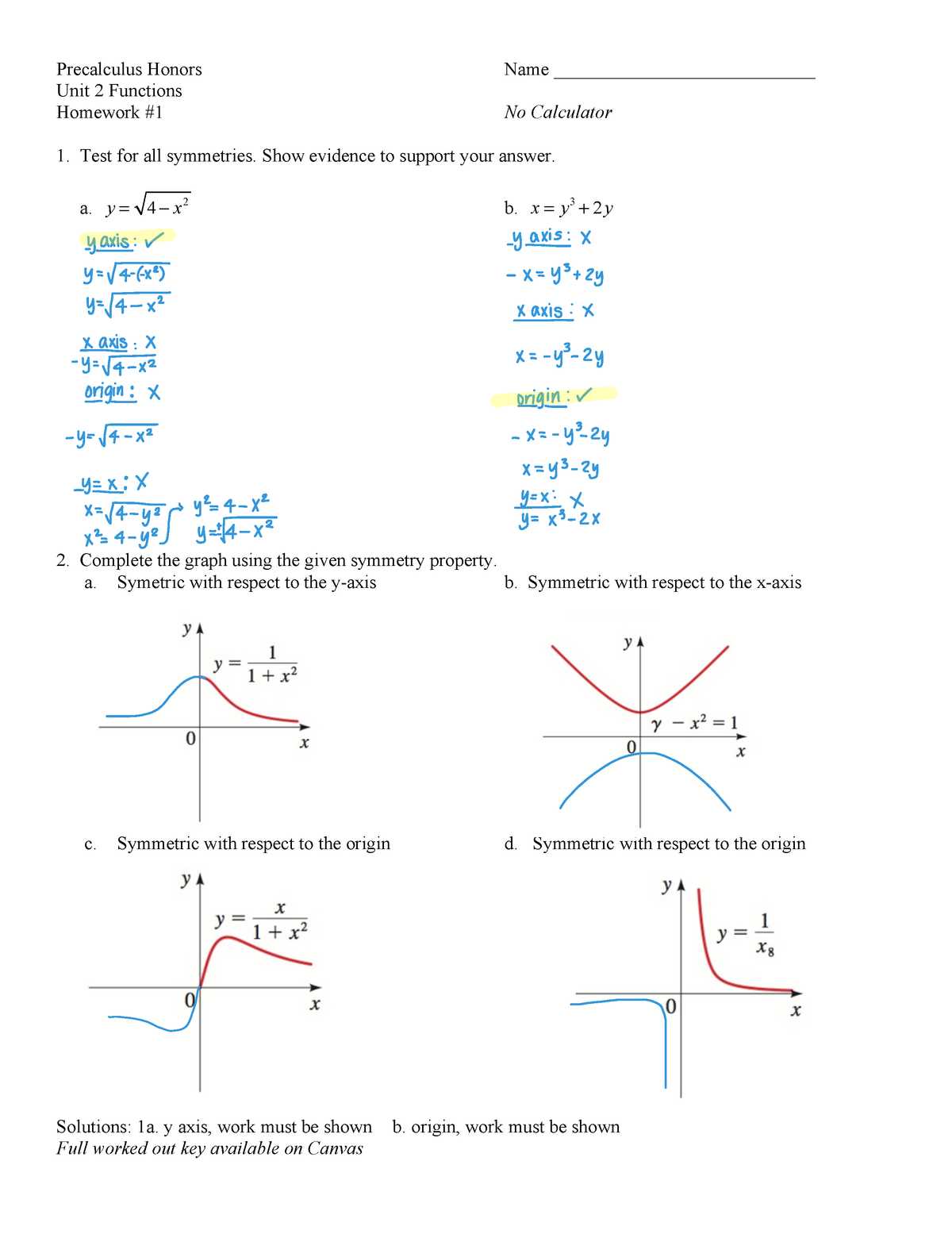
Applying Algebra to Precalculus Problems
Algebra plays a crucial role in solving a wide range of mathematical problems, particularly in advanced studies. By applying algebraic principles to more complex equations, you can break down difficult problems into manageable steps. This integration of algebra helps simplify expressions, solve for unknowns, and ultimately allows for a more effective approach to solving problems in higher-level mathematics.
Key Algebraic Concepts to Use
Factoring: Factoring is one of the most important tools when dealing with quadratic expressions or polynomials. Being able to factor expressions correctly can reveal hidden solutions and make the process of solving equations more straightforward.
- Solving quadratic equations: Using the quadratic formula or factoring techniques can help solve quadratic equations efficiently. These methods allow you to find real or complex solutions depending on the given values.
- Working with rational expressions: Simplifying fractions that involve polynomials is essential for solving equations that include rational terms. Understanding how to cancel out common factors helps simplify the process and avoid unnecessary complexity.
Using Algebra to Solve Complex Problems
In many cases, advanced mathematical problems require combining algebraic methods with other concepts. For instance, when working with functions, you may need to manipulate algebraic expressions to isolate variables or to combine terms in a way that reveals key relationships. In trigonometry, algebra helps simplify trigonometric identities or solve for unknown angles.
Strategies for applying algebra:
- Rearranging equations: Rewriting equations in different forms can help identify patterns or make it easier to solve for unknowns. This might involve moving terms from one side of the equation to the other or factoring to simplify the expression.
- Combining like terms: When working with polynomials or rational expressions, it’s essential to combine like terms. This reduces the number of terms you have to work with, making the equation easier to handle.
By mastering the use of algebra in these contexts, you can build a stronger foundation for solving a wide variety of problems and gain confidence in tackling more advanced mathematical concepts.
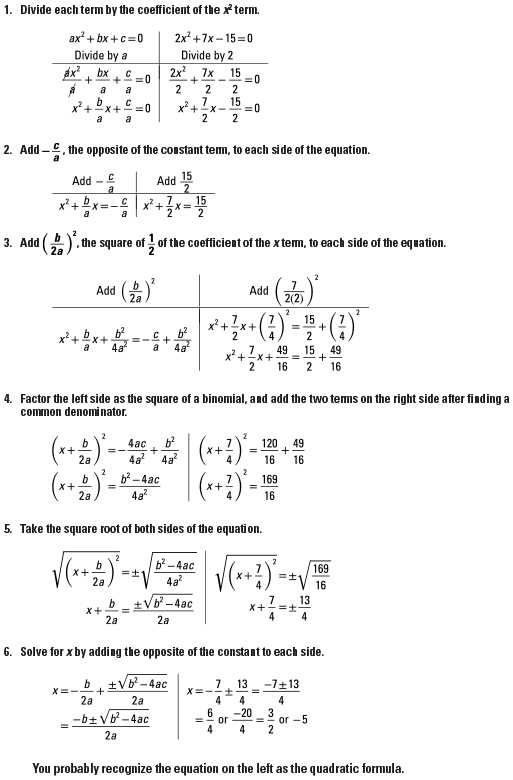
Reviewing Key Theorems and Formulas
A solid understanding of essential theorems and formulas is crucial for solving complex mathematical problems. These principles serve as the foundation for many calculations and can significantly streamline the problem-solving process. By familiarizing yourself with key theorems and formulas, you can approach tasks with greater efficiency and accuracy, while also gaining a deeper understanding of mathematical relationships.
Important Theorems to Remember
Mathematical theorems provide important truths that can simplify equations and clarify complex relationships. Mastering these theorems allows you to apply them directly to problems, saving time and avoiding unnecessary steps.
| Theorem | Description |
|---|---|
| Pythagorean Theorem | Used to relate the sides of a right triangle: ( a^2 + b^2 = c^2 ), where ( c ) is the hypotenuse. |
| Quadratic Formula | A formula for solving quadratic equations: ( x = frac{-b pm sqrt{b^2 – 4ac}}{2a} ). |
| Distributive Property | States that ( a(b + c) = ab + ac ), useful for simplifying expressions. |
Essential Formulas for Problem Solving
Formulas are shortcuts that allow for the quick calculation of values based on given variables. They often emerge from underlying theorems and provide efficient methods for solving specific types of problems.
| Formula | Usage |
|---|---|
| Sine, Cosine, and Tangent Ratios | Used in trigonometry to relate the angles of a right triangle to its sides. |
| Area of a Triangle | The formula ( A = frac{1}{2} times base times height ) is used to calculate the area of a triangle. |
| Circle Equation | The equation ( (x – h)^2 + (y – k)^2 = r^2 ) describes a circle with center ( (h, k) ) and radius ( r ). |
By reviewing and applying these key theorems and formulas, you can simplify complex problems, speed up calculations, and improve your overall problem-solving skills. Regular practice with these concepts will ensure you are well-prepared to tackle a wide range of mathematical challenges.
Understanding Polynomial and Rational Functions
Functions play a key role in mathematical analysis, with polynomial and rational functions being two fundamental types that appear frequently in algebraic problems. These functions help describe relationships between variables and are used in a variety of contexts, from simple equations to more complex models. A solid grasp of how these functions behave is essential for solving related problems and applying mathematical concepts effectively.
Polynomial functions are expressions involving powers of variables with non-negative integer exponents. They can take many forms and are often used to model real-world situations where quantities change in a predictable pattern. Rational functions, on the other hand, involve the ratio of two polynomials and can be more complex, often exhibiting asymptotic behavior or undefined points.
| Function Type | Form | Key Characteristics |
|---|---|---|
| Polynomial Function | ( f(x) = a_n x^n + a_{n-1} x^{n-1} + … + a_1 x + a_0 ) | Continuous, no denominators, the highest power determines the degree |
| Rational Function | ( f(x) = frac{P(x)}{Q(x)} ), where ( P(x) ) and ( Q(x) ) are polynomials | Can have vertical asymptotes, may be undefined for specific values of ( x ) |
Both function types have distinct properties that are essential when solving equations or analyzing graphs. Understanding their general behavior–such as how polynomial functions tend to smooth out and how rational functions can approach limits or become undefined–helps in their application to a wide range of problems.
Tips for Managing Time During Tests
Effective time management is essential during exams, especially when dealing with complex problems that require careful attention and multiple steps to solve. With limited time, it’s crucial to approach each section methodically, prioritizing tasks and allocating the appropriate amount of time for each one. By organizing your approach, you can reduce stress and improve your chances of completing the test with confidence.
One important strategy is to quickly skim through the entire test before diving into any questions. This gives you an overview of the content, allowing you to identify the questions that you can answer quickly and those that may take more time. After that, it’s wise to tackle the easier questions first, building momentum and ensuring that you secure those points before focusing on more challenging ones.
Another helpful tip is to set time limits for each section or question. For instance, allocate a fixed amount of time for each problem based on its complexity. If you’re spending too long on one question, move on to the next and come back later if time permits. This helps you avoid getting stuck and ensures that you give yourself a fair chance at completing the entire test.
Additionally, practice taking timed tests before the actual exam. This will help you become familiar with managing your time under pressure and improve your ability to pace yourself. Regular practice can also help you identify areas where you need to improve your speed or efficiency, so you can work on those areas in advance.
Improving Problem-Solving Speed
Increasing your speed when solving mathematical problems requires a combination of practice, strategy, and familiarity with key concepts. By refining your approach, you can become more efficient and confident in tackling problems, even under time constraints. Building speed is not about rushing through problems but about improving your ability to think critically and apply techniques quickly and accurately.
Practice Mental Math Techniques
One effective way to improve speed is to practice mental math. This involves performing calculations without relying on a calculator or paper. By doing this, you can reduce the time spent on basic arithmetic, allowing you to focus on more complex problem-solving. Regular practice with numbers, fractions, and percentages will also help increase your overall fluency.
Develop a Systematic Approach
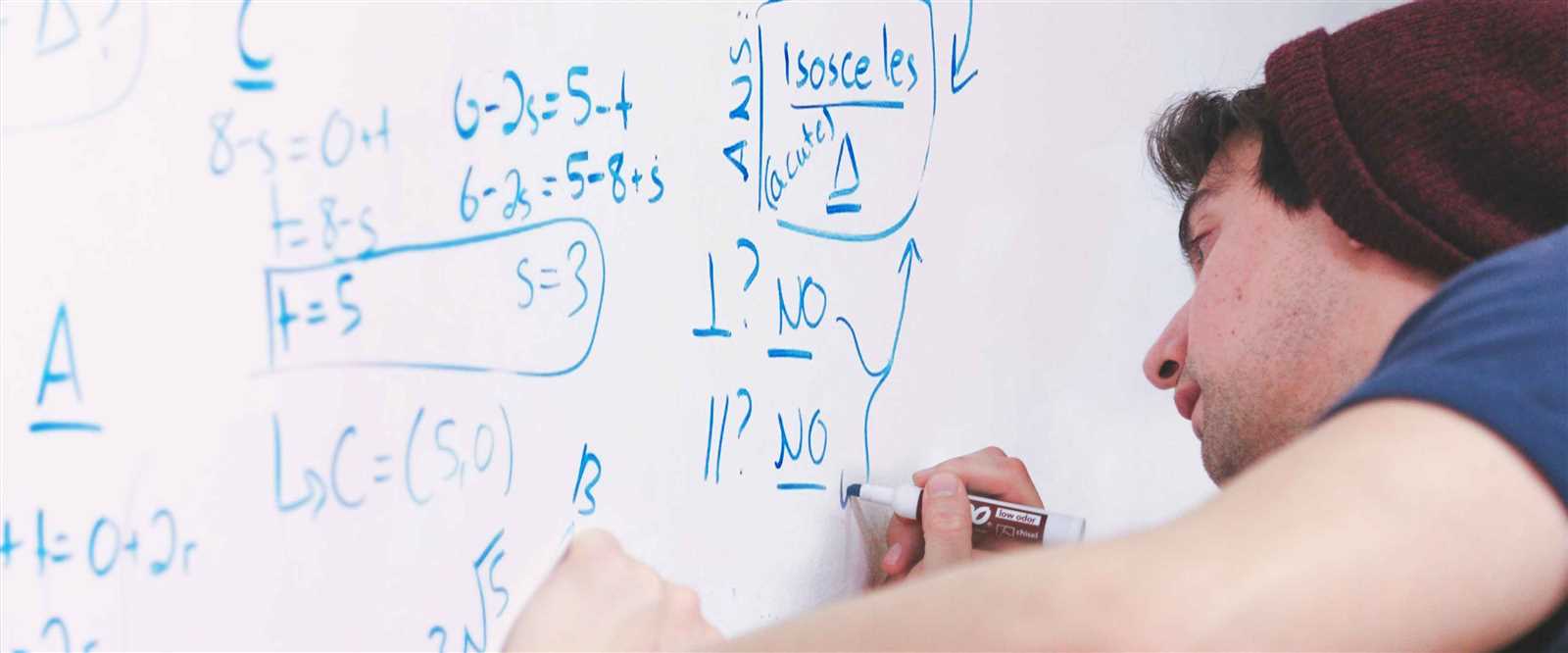
Another crucial factor in speeding up problem-solving is to develop a systematic approach. Start by breaking down each problem into smaller, manageable parts. Focus on identifying patterns, common techniques, or shortcuts that can simplify the process. Over time, this methodical approach will help you approach problems more quickly and with greater confidence.
Additionally, familiarizing yourself with common formulas, rules, and procedures will save valuable time when solving problems. The more you practice, the more you’ll recognize similar problem types, allowing you to apply known strategies more quickly.
Real-World Applications of Precalculus
Mathematical concepts learned in advanced algebra and functions have a wide range of applications in everyday life and various industries. From technology to engineering, these techniques help solve complex problems that impact our daily routines. Understanding how these concepts work in real-world scenarios can provide valuable insights into the practical use of math and its importance in shaping the modern world.
Applications in Engineering and Design

In engineering and architecture, understanding functions, curves, and rates of change is essential for designing structures and systems. Here are some key ways mathematics is used:
- Structural analysis: Engineers use functions to model how materials and structures will respond to forces such as tension, compression, and shear.
- Optimization: Functions help engineers design systems that are efficient, such as minimizing energy consumption or maximizing output in production systems.
- Simulation: Engineers simulate physical systems using mathematical models to predict outcomes, such as in fluid dynamics or thermal analysis.
Applications in Technology and Computing

Technology and computing fields rely heavily on advanced mathematical concepts to build software, create algorithms, and manage data. Some examples include:
- Computer graphics: Functions and transformations are used to create realistic images, animations, and simulations in video games and virtual environments.
- Cryptography: The development of secure communication systems relies on algorithms that use functions to encode and decode information.
- Data analysis: Statistical models and regression analysis, rooted in functions, help process and interpret large sets of data for decision-making in industries like finance and healthcare.
These applications highlight how mathematical skills are crucial in solving real-world problems, proving that the concepts we learn in the classroom extend far beyond textbooks and play a pivotal role in our technological advancement and innovation.
Resources for Extra Help
For those seeking additional support in mastering mathematical concepts, a wide variety of resources are available. These tools provide extra guidance, explanations, and practice to reinforce learning and boost confidence in tackling complex problems. Whether through online platforms, tutors, or educational materials, these resources can make a significant difference in achieving success.
Online Platforms and Websites
The internet offers numerous websites and platforms that cater to learners in need of extra assistance. Here are some valuable options:
- Khan Academy: A free platform with video lessons, practice exercises, and instructional material on various math topics.
- Wolfram Alpha: An advanced computational tool that helps solve equations and provides step-by-step solutions, perfect for visualizing complex problems.
- PatrickJMT: A YouTube channel dedicated to math tutorials, offering clear, concise explanations on a wide range of topics.
- Desmos: A powerful graphing calculator tool that assists in visualizing mathematical functions and their behaviors.
Personal Tutoring and Study Groups
Personalized learning can significantly enhance understanding, and there are several ways to engage with tutors or study groups:
- Private Tutors: Hiring a tutor for one-on-one sessions allows for customized learning, targeting areas where improvement is needed.
- Study Groups: Collaborating with peers in a study group can provide mutual support and different perspectives on solving problems.
- Online Tutoring Services: Websites like Chegg and Tutor.com offer expert tutoring services, available from the comfort of home, whenever needed.
By leveraging these resources, learners can gain a deeper understanding of mathematical concepts and improve their skills through various learning formats tailored to their individual needs.