
Understanding the foundational principles of mathematics is crucial for excelling in any assessment. Whether it’s tackling basic problems or more complex exercises, having a clear grasp of the core concepts is essential for success. This section focuses on strategies for mastering the initial stage of your mathematical journey, providing you with the necessary tools to navigate challenges effectively.
By reviewing essential ideas and techniques, you can enhance your problem-solving abilities and build confidence. The following guidelines will help you break down problems, apply logical reasoning, and identify patterns that will aid in achieving optimal results. With consistent practice and focus, your proficiency in mathematical tasks will improve, making future learning more manageable and rewarding.
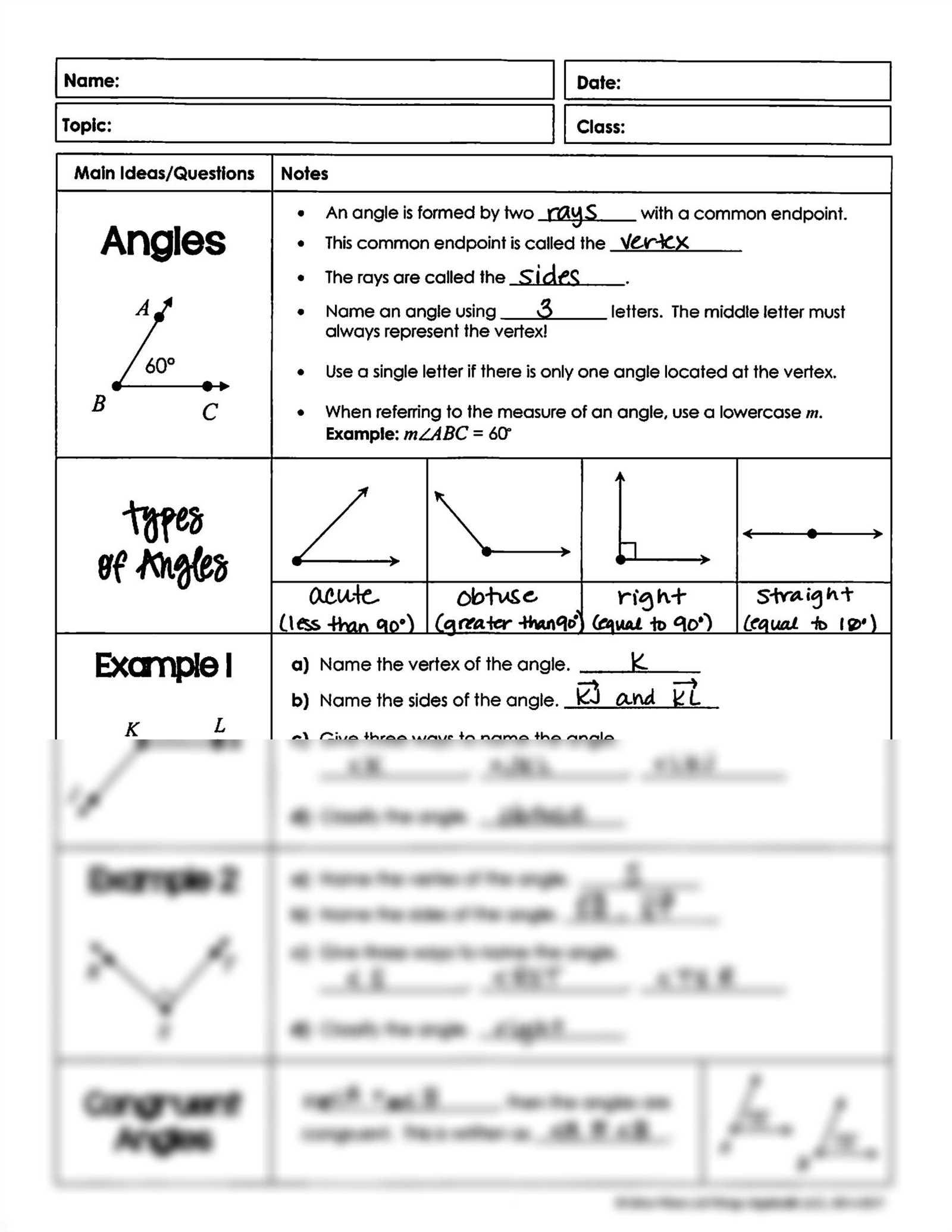
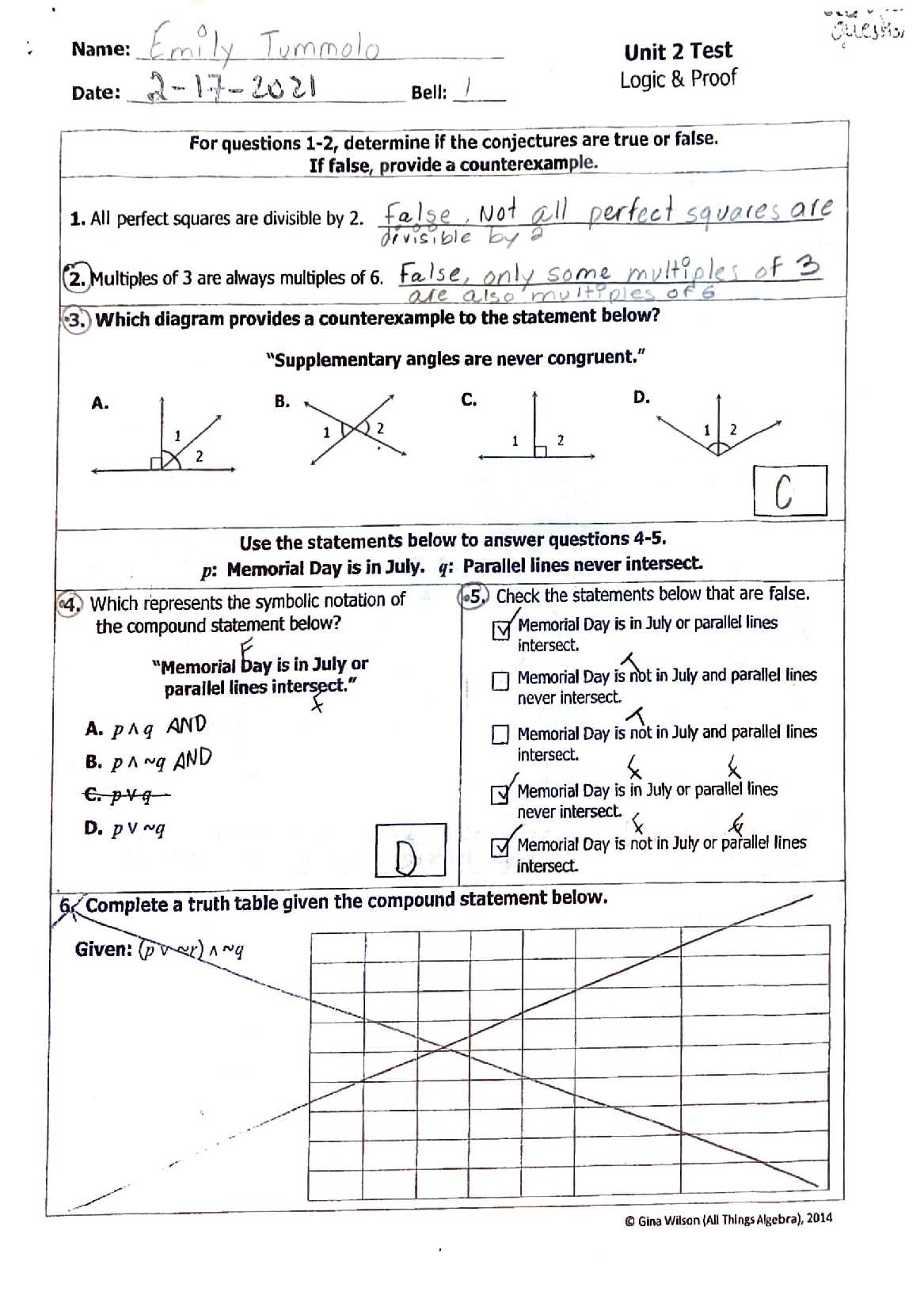
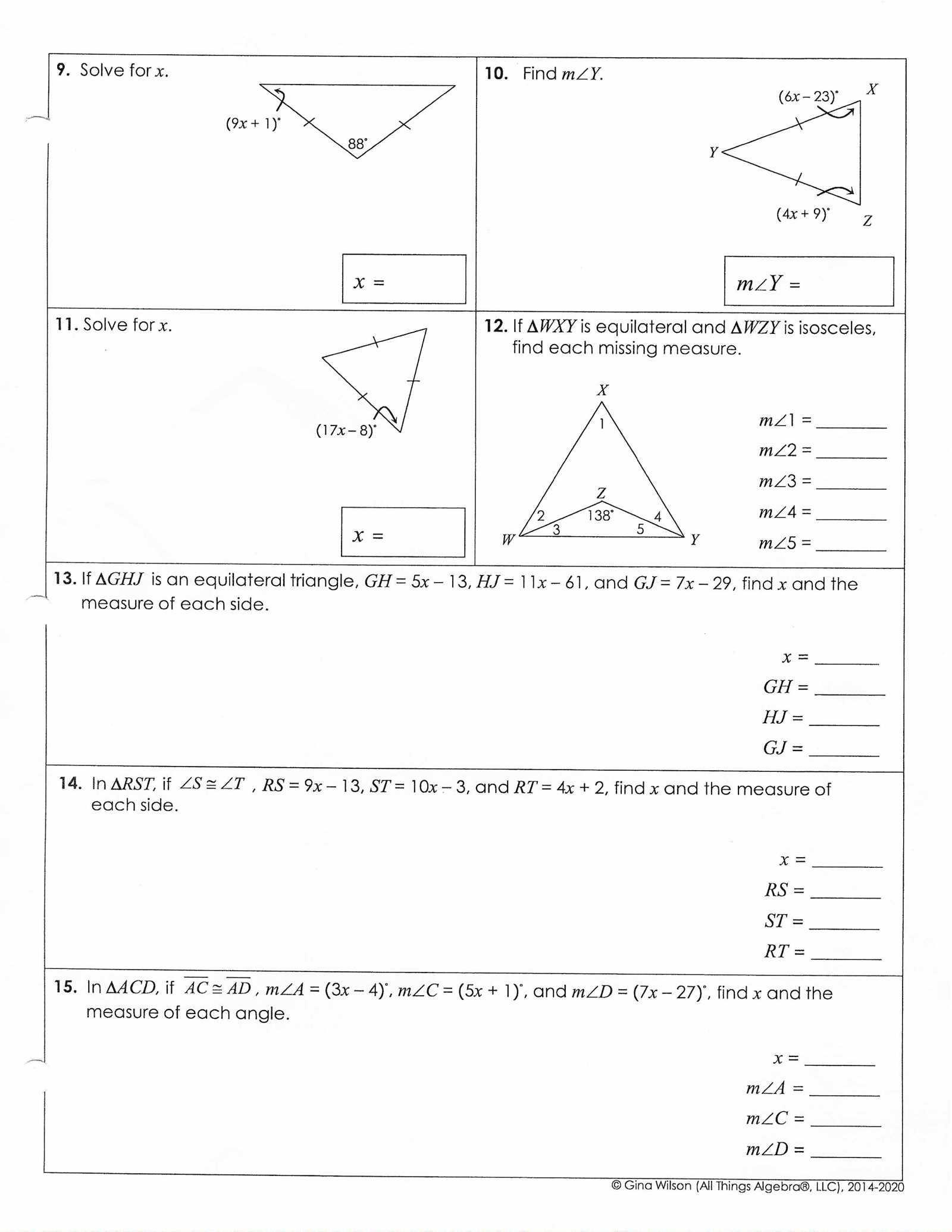
Geometry Unit 1 Test Overview

This section introduces the key elements of the first assessment in your mathematical journey. It is designed to evaluate your understanding of essential principles and your ability to apply them to various problems. The focus will be on fundamental concepts that form the building blocks for more advanced topics in the field.
The questions in this section are structured to test both your knowledge and problem-solving skills. Expect to encounter tasks that require you to identify shapes, calculate dimensions, and understand relationships between different components. Mastering these areas will lay a solid foundation for tackling more complex material in future assessments.
Key Concepts in Geometry Unit 1
Understanding the fundamental principles in this initial phase is crucial for mastering the subject. This section focuses on core topics that form the foundation of the material. A strong grasp of these concepts will enable you to approach a variety of challenges with confidence and clarity.
Basic Shapes and Their Properties
In this section, the focus is on familiar geometric shapes such as triangles, quadrilaterals, and circles. It’s important to understand their defining characteristics, such as angles, sides, and symmetries. Recognizing how these elements interact is essential for solving a variety of problems.
Measurement and Calculations
Accurate measurement plays a central role in solving mathematical problems. From calculating area and perimeter to understanding scale and proportion, mastering these techniques will ensure you are prepared for more advanced topics in future assessments. Knowing how to apply formulas and interpret results is key to achieving success.
Understanding Question Types
In any mathematical evaluation, it’s crucial to recognize the different styles of questions you’ll encounter. Understanding the format of these questions helps you strategize your approach and solve them more efficiently. This section will explore common question types and how to tackle them effectively.
Multiple Choice Questions
Multiple choice questions typically present a problem along with several potential solutions. Your task is to select the correct one. These questions are designed to assess your ability to quickly identify the right method or answer from a set of options.
- Read each option carefully.
- Eliminate obviously incorrect choices.
- Double-check calculations before finalizing your answer.
Problem-Solving Questions
Problem-solving questions require a more in-depth understanding of the material. You may need to demonstrate your ability to apply theories and formulas to new situations. These questions often test your critical thinking and logical reasoning skills.
- Identify the given information.
- Determine which formula or concept is relevant.
- Work through the problem step-by-step to find the solution.
How to Approach Mathematical Problems
When faced with a mathematical challenge, having a systematic approach can greatly improve your chances of finding the correct solution. Instead of jumping straight into calculations, it’s helpful to first analyze the problem and break it down into manageable steps. This approach will help you stay organized and reduce the likelihood of errors.
Step-by-Step Strategy
To solve any problem effectively, it’s essential to follow a logical sequence of steps. Start by identifying the known values, then determine what you’re asked to find. Once you have a clear understanding of the problem, use relevant formulas or concepts to guide your work.
- Identify the given data and what needs to be determined.
- Choose the appropriate method or formula for the situation.
- Break the problem into smaller parts and solve incrementally.
- Check your work for any inconsistencies or mistakes.
Visualization and Diagrams
For many problems, drawing a diagram can help you visualize the situation more clearly. Whether it’s a shape, a series of measurements, or a relationship between variables, diagrams can make complex problems easier to understand and solve.
- Sketch the problem if possible, marking all given information.
- Label key elements, such as angles, sides, or points of intersection.
- Use the diagram as a reference to guide your calculations.
Step-by-Step Guide to Solving Questions
Approaching each question systematically is essential for achieving accurate results. By following a structured method, you can break down the problem and focus on solving it step by step. This guide outlines the process that will help you tackle problems efficiently and minimize errors.
Understanding the Problem
The first step is always to fully understand what the question is asking. This involves identifying the given information and determining what needs to be found. Pay close attention to the wording, as it will provide clues about the method or formula you should use.
- Read the problem carefully and underline key details.
- Note down any given values or relationships between elements.
- Identify the unknowns and what you are required to solve for.
Applying the Appropriate Method

Once you understand the problem, choose the most suitable approach for solving it. This might involve using specific formulas, logical reasoning, or making use of visual aids like diagrams. By applying the correct method, you ensure a structured approach to finding the solution.
- Choose the formula or method that best fits the given information.
- Substitute the known values into the formula or equation.
- Perform the necessary calculations step by step.
- Double-check your work for accuracy and consistency.
Common Mistakes in Unit 1 Tests
While preparing for any assessment, it’s easy to make simple errors that can cost valuable points. Recognizing these common mistakes early on can help you avoid them and improve your overall performance. In this section, we’ll highlight the typical pitfalls and offer tips on how to stay on track.
One frequent mistake is misinterpreting the question or overlooking crucial details. It’s important to read every part of the problem carefully to ensure you fully understand what’s being asked. Skipping over key pieces of information can lead to incorrect solutions, even if your calculations are otherwise correct.
- Not double-checking the problem’s wording.
- Missing out on essential data or instructions.
- Confusing similar terms or concepts.
Another common error is rushing through calculations without properly organizing your work. Failing to set up the problem in a clear and structured way can result in mistakes. Taking time to work through each step methodically ensures accuracy and reduces the likelihood of errors.
- Skipping intermediate steps when solving a problem.
- Forgetting to check the units or dimensions involved.
- Not reviewing your final answer for reasonableness.
Important Theorems for Unit 1

In any mathematical discipline, understanding key principles is essential for solving problems effectively. Theorems provide the foundational rules that guide calculations and reasoning. In this section, we will explore the core theorems that form the basis for the first stage of study, helping you to approach problems with clarity and precision.
Basic Angle Relationships
One of the most important concepts in early learning is the relationship between angles. Theorems such as the angle sum property of triangles and the vertical angle theorem are fundamental for solving various problems. These principles help identify unknown angles and make it easier to work with shapes and figures.
- The sum of the angles in a triangle is always 180°.
- Vertical angles are congruent, meaning they have the same measure.
- Complementary and supplementary angles add up to 90° and 180°, respectively.
Properties of Parallel Lines
Parallel lines and the angles formed by them are critical when analyzing different geometric structures. The theorems related to alternate interior angles, corresponding angles, and consecutive interior angles are essential tools for solving problems involving parallel lines.
- Alternate interior angles are equal when two parallel lines are cut by a transversal.
- Corresponding angles are congruent when a transversal crosses parallel lines.
- Consecutive interior angles are supplementary, meaning they sum up to 180°.
Review of Geometric Proofs and Logic
In any mathematical framework, reasoning and logical structure are key components in proving statements and solving problems. The process of proving geometric relationships involves using previously established facts and applying logical steps to reach a conclusion. This section will review the fundamental methods and strategies for constructing geometric proofs and applying logical reasoning in problem-solving scenarios.
Logical Foundations of Proofs
To create valid proofs, it’s essential to follow a logical progression. Each step must be justified by known postulates, theorems, or definitions. Understanding the basic principles of logic, such as direct and indirect proofs, is crucial for building strong arguments. Here are some of the most common methods used:
| Method | Description |
|---|---|
| Direct Proof | Start with known facts and use logical steps to prove a statement. |
| Indirect Proof | Assume the opposite of what you need to prove, then show that it leads to a contradiction. |
| Proof by Contradiction | Assume the statement is false and show that this assumption leads to an impossible situation. |
Commonly Used Theorems in Proofs
Several key theorems are frequently used in geometric proofs. Understanding these principles allows you to apply them efficiently and accurately in various scenarios. Some of the most essential theorems include properties of angles, lines, and shapes that are crucial for constructing logical arguments in proofs.
| Theorem | Application |
|---|---|
| Angle Sum Theorem | The sum of the angles in a triangle is always 180°. |
| Congruence Theorems | Criteria to prove two triangles are congruent, such as SSS, SAS, ASA, and AAS. |
| Parallel Postulate | If a line is parallel to another, certain angle relationships hold, such as alternate interior angles being congruent. |
Essential Formulas for Unit 1
When studying any field of mathematics, formulas play a critical role in simplifying calculations and solving problems effectively. By mastering key equations, you can approach problems with confidence and precision. This section will cover the essential formulas that are crucial for the initial stage of your learning, helping you navigate through common problems efficiently.
Area of a Triangle
To calculate the area of a triangle, use the formula:
Area = 1/2 × base × height.
This formula is fundamental for finding the area of triangles with known base and height measurements.
Pythagorean Theorem
For right-angled triangles, the Pythagorean theorem is indispensable. It states that:
a² + b² = c²,
where a and b are the lengths of the two legs, and c is the length of the hypotenuse. This formula helps solve for unknown sides of right triangles.
Perimeter of a Rectangle
The perimeter of a rectangle is calculated by:
Perimeter = 2 × (length + width).
This formula is useful when you need to find the total distance around the outer edges of a rectangle.
Circumference of a Circle
The circumference of a circle can be determined with the formula:
Circumference = 2 × π × radius.
This equation is essential for problems involving circular shapes or motion.
By memorizing these basic formulas and understanding how to apply them, you’ll be well-prepared to solve a variety of problems in the early stages of your study. Remember to practice regularly to become more familiar with these calculations and use them with ease in different scenarios.
Tips for Effective Time Management

Managing your time effectively is key to performing well in any academic endeavor. Proper planning and organization can help you stay on track and reduce stress, ensuring you have enough time to focus on each task. In this section, we’ll explore useful strategies for making the most of your time while studying and preparing for assessments.
One of the most important aspects of time management is creating a structured schedule. Having a clear plan of action helps you allocate time for each subject and task, preventing last-minute cramming. By breaking your study sessions into manageable chunks, you can maintain focus and retain information more effectively.
| Tip | Description |
|---|---|
| Prioritize Tasks | Focus on the most important tasks first, and tackle easier ones once you’ve made progress on harder topics. |
| Use a Timer | Implement time blocks for studying (e.g., 25-minute sessions) followed by short breaks to stay fresh and productive. |
| Set Realistic Goals | Break down larger tasks into smaller, more achievable goals to track your progress without feeling overwhelmed. |
| Eliminate Distractions | Minimize interruptions by turning off notifications and creating a quiet, dedicated study environment. |
By following these tips, you can maximize your productivity and manage your time more effectively, ultimately improving your academic performance. Stay consistent with your schedule and adjust it as needed to ensure you’re making the best use of your available time.
Practice Questions and Sample Answers
To reinforce your understanding and improve problem-solving skills, practicing with sample questions is essential. Working through problems allows you to apply concepts learned and develop strategies for tackling various types of challenges. In this section, we provide practice problems along with solutions to guide your preparation.
Each practice question is designed to test different aspects of your knowledge, ensuring that you’re able to approach similar problems with confidence. Below are a few sample problems with step-by-step solutions to help you identify key concepts and methods for solving them effectively.
Practice Question 1:
Find the area of a triangle with a base of 10 units and a height of 6 units.
Solution:
To find the area of the triangle, use the formula:
Area = 1/2 × base × height.
Substitute the given values:
Area = 1/2 × 10 × 6 = 30 square units.
Practice Question 2:
Calculate the perimeter of a rectangle with a length of 8 units and a width of 5 units.
Solution:
To find the perimeter of a rectangle, use the formula:
Perimeter = 2 × (length + width).
Substitute the given values:
Perimeter = 2 × (8 + 5) = 26 units.
Practice Question 3:
Given two lines that are parallel, find the measure of alternate interior angles if one of the angles measures 40°.
Solution:
When two lines are parallel, alternate interior angles are congruent. Therefore, the other angle also measures 40°.
By practicing with these examples, you’ll build confidence in applying formulas and methods to solve similar problems. Keep practicing to improve your speed and accuracy in answering various questions.
Strategies for Test Success in Geometry
Achieving success in any academic evaluation requires a blend of preparation, practice, and strategy. To excel in assessments that focus on spatial reasoning and mathematical principles, it is crucial to approach the material with a clear plan and structured study routine. In this section, we’ll explore strategies that can help you perform at your best and avoid common pitfalls.
One key approach is to ensure that you understand the foundational concepts thoroughly before moving on to more complex problems. Take the time to review essential principles and practice applying them in different scenarios. Doing so will help you recognize patterns and shortcuts during the actual assessment, saving valuable time.
Another critical strategy is time management. Make sure you allocate enough time for each section of the exam. Don’t spend too long on any single problem–if you’re stuck, move on and return to it later with a fresh perspective. Remember that completing all questions, even with some uncertainty, is often more beneficial than leaving blanks.
Additionally, always read the instructions and questions carefully. Misinterpreting a question can lead to avoidable mistakes. Pay attention to units, specific wording, and any given conditions that may affect how you approach a problem.
Lastly, practicing with sample problems and reviewing past exercises is vital. The more you work through practice questions, the more prepared you’ll be. If you make a mistake, take the time to understand why it happened and how you can avoid it in the future.
By following these strategies, you can boost your confidence and improve your performance during assessments, setting yourself up for success.
How to Use Diagrams in Tests
Diagrams are essential tools that help you visualize problems and better understand their structure. They provide a clear representation of the problem, allowing you to identify key elements and relationships between different parts. Effectively interpreting and utilizing diagrams during assessments can significantly enhance your problem-solving abilities and increase your accuracy.
When you encounter a diagram, take the time to carefully examine it. Look for labeled points, lines, angles, and shapes, as these details are crucial to solving the problem. Often, the diagram will provide hints or constraints that are important for finding the solution. By paying close attention to these aspects, you can avoid common mistakes and ensure that you’re using the correct information.
In some cases, you may need to draw your own diagram based on the problem description. In these situations, it is important to represent the information as accurately as possible. Use rulers, protractors, or other tools to ensure that your diagram is precise. A well-drawn diagram can make it much easier to identify relationships and apply the appropriate formulas or methods.
Here are some tips for using diagrams effectively in assessments:
- Label all elements: Always label key points, angles, and sides as soon as you draw a diagram. This will help you avoid confusion later.
- Mark known values: If the problem gives you specific measurements, make sure to write them on the diagram to make them easy to reference.
- Look for symmetry: In many problems, symmetry can help simplify the solution process. Identifying symmetry can reveal shortcuts and relationships that aren’t immediately obvious.
- Draw auxiliary lines: If necessary, draw extra lines to help visualize relationships between different parts of the diagram. For example, adding perpendicular lines or diagonals can make it easier to apply theorems.
By mastering the art of using diagrams, you’ll be able to approach problems with a clearer understanding, which can lead to more efficient and accurate solutions.
Terminology You Need to Know
Understanding key terms is essential when solving problems related to shapes, space, and their properties. Whether you are working with angles, lines, or figures, knowing the correct vocabulary helps you interpret questions, understand concepts, and apply formulas more effectively. Mastering the terminology can lead to clearer problem-solving and a deeper grasp of the subject.
Here are some important terms that are crucial for success in assessments related to spatial reasoning and mathematical relationships:
Basic Shapes and Figures
These are the foundational objects you will work with. It is essential to know their characteristics and properties:
- Circle: A round shape where every point is equidistant from the center.
- Triangle: A three-sided polygon, with different types such as equilateral, isosceles, and scalene.
- Square: A four-sided polygon where all sides are equal and every angle is 90 degrees.
- Rectangle: A four-sided polygon with opposite sides equal and all angles being 90 degrees.
Angles and Relationships
Understanding the relationships between different angles and their properties is key to solving problems:
- Acute Angle: An angle smaller than 90 degrees.
- Right Angle: An angle equal to 90 degrees.
- Obtuse Angle: An angle larger than 90 degrees but smaller than 180 degrees.
- Complementary Angles: Two angles that add up to 90 degrees.
- Supplementary Angles: Two angles that add up to 180 degrees.
By familiarizing yourself with these terms and concepts, you will be better prepared to approach problems with confidence and clarity. Understanding the language of shapes and measurements is not only important for answering questions but also for gaining a deeper insight into spatial relationships.
Common Topics in Early Assessments
In the early stages of exploring spatial reasoning and mathematical relationships, several concepts are typically covered. Understanding these topics is crucial as they lay the foundation for more complex ideas and calculations. These themes often involve working with shapes, angles, properties, and the basic principles of measurement.
Here are some of the most frequently tested concepts in the initial stages of the subject:
Basic Shapes and Their Properties
Identifying and understanding the properties of various shapes is essential for solving problems related to spatial arrangements and measurements:
- Triangles: Recognizing different types such as equilateral, isosceles, and scalene, and understanding their properties.
- Quadrilaterals: Knowing the characteristics of squares, rectangles, parallelograms, and trapezoids.
- Circles: Understanding the radius, diameter, circumference, and area calculations.
Angles and Relationships
Angles play a vital role in understanding spatial configurations. Familiarizing yourself with their relationships is critical:
- Acute, Right, and Obtuse Angles: Identifying and working with different angle types and their sum properties.
- Complementary and Supplementary Angles: Recognizing when two angles combine to form 90° or 180° respectively.
- Angle Bisectors: Understanding how dividing an angle into two equal parts works.
Mastering these fundamental concepts provides a strong foundation for approaching more advanced topics and helps develop problem-solving strategies in mathematical reasoning.
Preparing for Future Mathematical Assessments
Success in future assessments requires a strategic approach that builds on your current understanding while focusing on areas that need improvement. By adopting effective study habits and preparing with purpose, you can enhance your problem-solving skills and mathematical reasoning. Preparation is not just about memorizing formulas but also about practicing application and developing critical thinking abilities.
Consistent Practice and Review
Regular practice is key to mastering the concepts that will be tested. It is important to review previously learned material while continuing to practice new problems:
- Practice with varied problems: Tackle a range of questions from easy to difficult to build confidence and versatility.
- Review past material: Go over older concepts to reinforce your knowledge and ensure you retain foundational skills.
- Use practice resources: Leverage books, online quizzes, and practice exams to mimic real testing conditions.
Focus on Key Areas
In addition to general practice, focus on areas where you are less confident. This could include understanding specific properties, mastering theorems, or solving complex problems. By targeting these areas, you can ensure a more thorough grasp of all necessary topics:
- Mastering difficult concepts: Focus on areas that challenge you, such as complex relationships or advanced calculations.
- Ask for help: When unsure, seek clarification from teachers, tutors, or study groups to fill knowledge gaps.
With consistent effort, a targeted approach to review, and regular practice, you can be well-prepared for any upcoming assessments. By the time your exams approach, you will have the skills and confidence necessary to perform well under pressure.