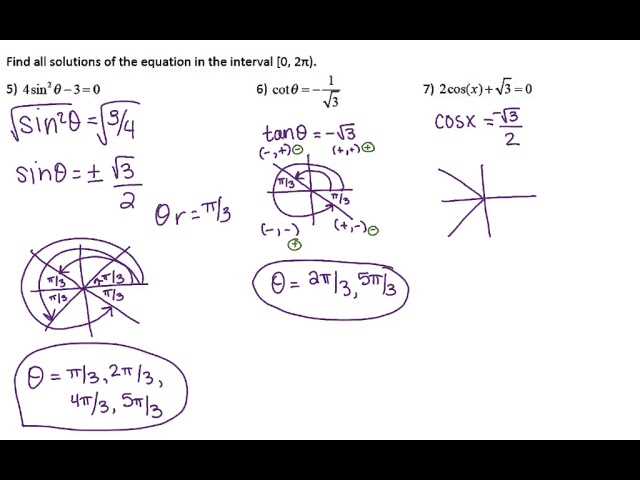
In this section, we will explore the essential mathematical principles needed to excel in more advanced topics. You will gain a deeper understanding of core ideas that form the foundation for solving problems efficiently. By focusing on specific methods and strategies, you can improve your problem-solving skills and build confidence in applying these techniques.
Throughout this guide, we break down the most important areas that you need to grasp. From identifying patterns to mastering algebraic manipulations, each concept plays a critical role in achieving success. Whether you’re practicing for an upcoming evaluation or simply strengthening your skills, this resource is designed to help you achieve your goals.
Precalculus Chapter 3 Test Answers
In this section, we will review key solutions and step-by-step processes to help you understand how to approach a variety of problems. This guide is designed to enhance your problem-solving skills, allowing you to grasp the methods necessary to arrive at the correct conclusions. Each example will demonstrate different techniques to effectively tackle complex questions, ensuring a deeper understanding of the material.
Whether dealing with polynomial expressions, function analysis, or graphing challenges, mastering these strategies is essential for gaining confidence in handling similar problems. The following table presents a variety of practice questions and their corresponding solutions, helping you to further comprehend the steps involved in solving these types of problems.
| Problem | Solution |
|---|---|
| Factor x² + 5x + 6 | (x + 2)(x + 3) |
| Find the vertex of y = x² – 4x + 3 | Vertex at (2, -1) |
| Solve for x: 2x² – 4x = 0 | x = 0 or x = 2 |
| Graph y = 2x – 4 | Linear equation with slope 2 and y-intercept -4 |
| Simplify: (3x² + 5x) – (2x² – 4x) | x² + 9x |
Understanding Key Concepts in Chapter 3
Grasping the foundational ideas of this section is crucial for mastering more advanced topics. The core principles explored here form the building blocks for tackling increasingly complex problems. These concepts are essential for improving your skills and gaining a deeper understanding of mathematical relationships, from manipulating expressions to analyzing graphs and functions.
As you work through different types of problems, it is important to recognize the key techniques that will help simplify solutions. Understanding how to identify patterns and apply appropriate methods is the key to success. The table below highlights some of the main concepts and their corresponding strategies to help reinforce your knowledge.
| Concept | Strategy |
|---|---|
| Factoring Quadratics | Look for common factors and apply the FOIL method |
| Solving Systems of Equations | Use substitution or elimination to find solutions |
| Graphing Linear Functions | Identify slope and y-intercept to plot the graph |
| Identifying Functions | Use vertical line test to confirm if it is a function |
| Simplifying Rational Expressions | Factor both numerator and denominator to cancel common terms |
Important Formulas for Precalculus
To successfully navigate through complex problems, it is essential to become familiar with key formulas that simplify and guide the solution process. These formulas are vital for understanding how different mathematical concepts relate to each other and how to efficiently manipulate expressions. Mastering these relationships will provide the foundation needed to tackle more advanced tasks with confidence.
Essential Algebraic Formulas
- Quadratic Formula: x = (-b ± √(b² – 4ac)) / 2a – Used to solve quadratic equations.
- Difference of Squares: a² – b² = (a + b)(a – b) – Useful for factoring expressions.
- Sum of Cubes: a³ + b³ = (a + b)(a² – ab + b²) – A formula for factoring cubic expressions.
Key Graphing Formulas
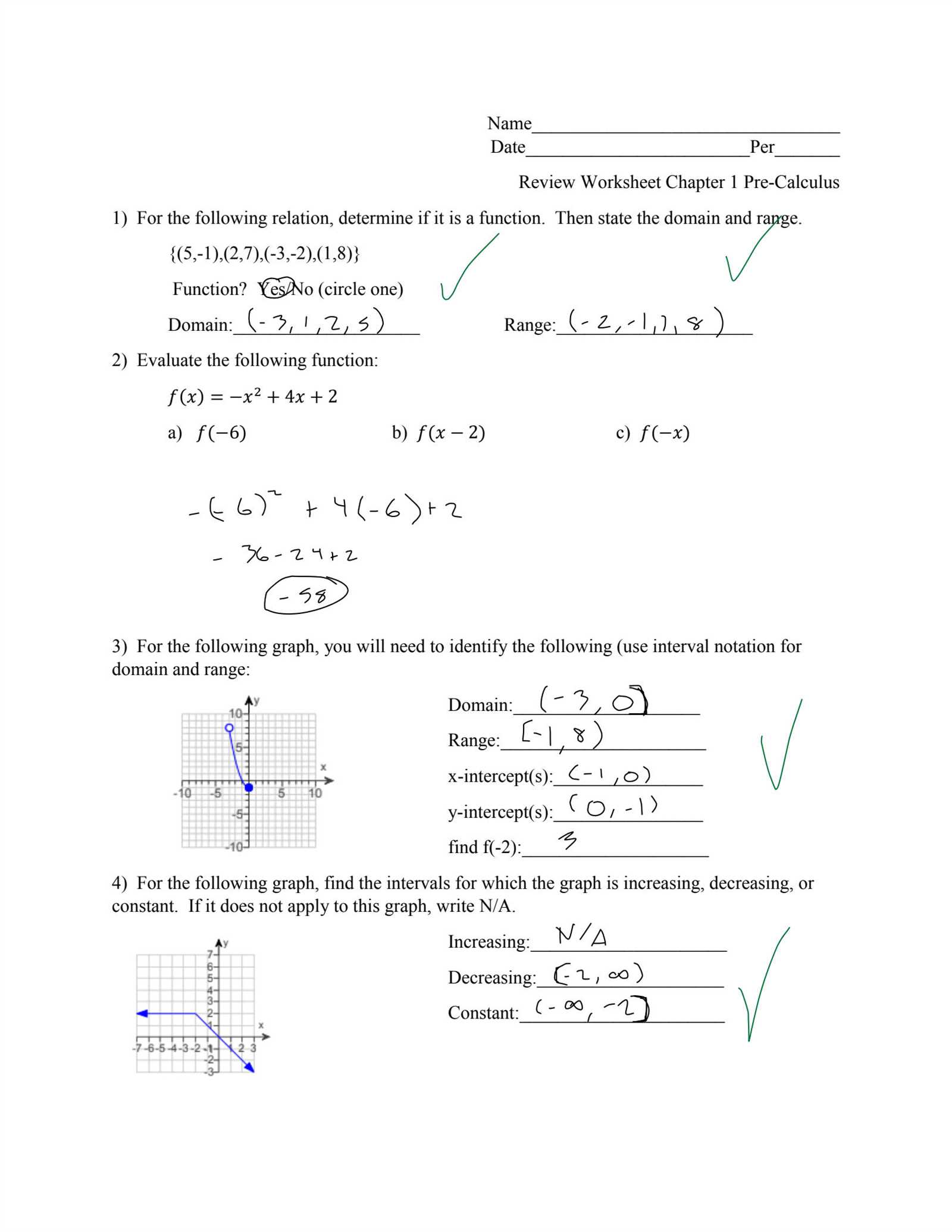
- Slope-Intercept Form: y = mx + b – Used to express linear equations.
- Point-Slope Form: y – y₁ = m(x – x₁) – Useful for finding the equation of a line given a point and slope.
- Standard Form of a Line: Ax + By = C – Another way to represent a linear equation.
These formulas serve as a powerful toolkit to solve various types of problems. Familiarizing yourself with them will streamline your approach and enable you to work more efficiently through each exercise.
How to Approach Chapter 3 Problems
Successfully solving mathematical problems requires a structured approach, especially when dealing with complex expressions and functions. To make the process more manageable, breaking down each problem into smaller, more manageable steps is key. By understanding the underlying principles and applying systematic methods, you can approach each question with confidence and clarity.
Step-by-Step Problem Solving
Start by carefully reading the problem to identify what is being asked. Pay attention to key information, such as the type of equation or the specific concept involved. Once you have a clear understanding, follow these steps:
- Identify the Type of Problem: Determine whether the problem involves solving equations, graphing functions, factoring expressions, or something else.
- Apply Relevant Formulas: Use the appropriate formulas or methods that best suit the type of problem at hand.
- Simplify Step by Step: Break down the problem into smaller parts and simplify each part before moving on to the next.
- Double Check Your Work: Once you reach a solution, review your calculations to ensure accuracy.
Common Problem Types
Throughout this section, you will encounter a variety of problems. Some of the most common include:
- Solving Quadratic Equations: Often requires factoring or using the quadratic formula.
- Graphing Functions: Requires an understanding of slope, intercepts, and transformations.
- Working with Rational Expressions: Involves simplifying or finding values that make expressions undefined.
By adopting a consistent approach and familiarizing yourself with the different types of problems, you’ll improve your ability to solve challenges efficiently and accurately.
Common Mistakes to Avoid in Tests
When tackling mathematical problems, it’s easy to overlook small details that can lead to errors. These mistakes often arise from misinterpreting the problem, rushing through steps, or neglecting important concepts. Being aware of these common pitfalls can help you avoid them and improve your accuracy when solving problems.
One of the most frequent errors is not properly reading the instructions or missing key information. Another common mistake is skipping steps or attempting to take shortcuts without fully understanding the process. Additionally, failing to double-check your work can lead to simple miscalculations or overlooked details. Paying attention to every step, however small, ensures that you stay on track and produce the correct solution.
Another critical area is the improper use of formulas or forgetting to apply the correct method for a given problem. Whether dealing with algebraic equations, graphing functions, or simplifying expressions, it’s important to apply the appropriate technique each time. Lastly, time management can also be a factor; rushing through problems without properly pacing yourself can result in avoidable mistakes.
Tips for Effective Time Management
Managing time efficiently is essential when tackling complex problems under time constraints. Without proper planning, it’s easy to waste valuable minutes on difficult sections, leaving less time for others. By developing a strategic approach, you can allocate enough time to each problem and maximize your performance during evaluations.
One of the most effective strategies is to allocate specific time slots for each question. By keeping track of time, you ensure that no problem is rushed or neglected. Another important tip is to focus on easier questions first, allowing you to build confidence and secure quick points before tackling more challenging problems. The following table provides a basic guide to help manage time effectively during assessments.
| Strategy | Description |
|---|---|
| Prioritize Simpler Problems | Complete easier problems first to secure quick points and save time for harder ones. |
| Time Allocation Per Question | Set a specific time limit for each problem to avoid spending too much time on one task. |
| Practice Under Time Constraints | Regularly practice problems within set time limits to improve speed and efficiency. |
| Review at the End | Reserve a few minutes at the end of the session to check your work for mistakes. |
By implementing these techniques, you can enhance your ability to manage time effectively, ensuring that each problem receives the attention it deserves without unnecessary stress.
Strategies for Solving Polynomial Equations
Solving polynomial equations requires a systematic approach that involves recognizing the form of the equation and applying the most efficient methods for finding the solutions. Polynomials can vary in complexity, from simple quadratic equations to higher-degree expressions. By mastering specific strategies, you can approach these problems with confidence and accuracy.
Factoring Techniques
One of the most effective ways to solve polynomial equations is by factoring the expression. Factoring breaks the polynomial down into simpler binomials or terms, which can then be set equal to zero to find the roots. Common methods include:
- Factoring by Grouping: This method works well when the polynomial has four terms. Group terms in pairs, factor each group, and then factor out the common binomial.
- Difference of Squares: If the polynomial is in the form of a² – b², it can be factored as (a + b)(a – b).
- Trinomial Factoring: For quadratics and higher-degree polynomials, look for two numbers that multiply to give the product of the first and last terms and add to the middle term.
Using the Rational Root Theorem
For higher-degree polynomials that do not factor easily, the Rational Root Theorem can help identify possible rational roots. The theorem suggests that any rational solution is a factor of the constant term divided by a factor of the leading coefficient. Testing these potential roots through substitution can help determine which, if any, are valid solutions.
Once you find a root, you can use synthetic division or long division to reduce the polynomial to a lower degree, making it easier to solve for remaining roots. This process allows you to break down complex problems step by step.
Graphing Techniques for Chapter 3
Graphing mathematical functions is a powerful tool for visualizing the relationships between variables. In many problems, understanding how a function behaves on a graph can simplify the process of finding solutions. Whether you’re dealing with linear, quadratic, or more complex expressions, certain graphing techniques can help you interpret the function’s key features and solve problems more effectively.
Before you begin plotting any graph, it’s important to identify the type of function you’re working with. Once that’s determined, applying the appropriate methods to sketch the graph accurately will make it easier to analyze its key characteristics, such as intercepts, slope, and asymptotes.
Key Graphing Strategies
- Identifying Intercepts: The x-intercepts occur where the graph crosses the x-axis, while the y-intercept is where the graph intersects the y-axis. These points are often critical in understanding the behavior of the function.
- Plotting Critical Points: For more complex functions, plotting key points, such as maxima, minima, and turning points, is crucial to understanding the function’s behavior over its domain.
- Using Symmetry: Many functions exhibit symmetry, either about the y-axis (even functions) or the origin (odd functions). Recognizing this can help in reducing the number of points you need to plot.
- Scaling the Axes: Properly scaling the x and y axes is important to accurately represent the function. Be mindful of the intervals and the values they represent to ensure a proportional and accurate graph.
Advanced Graphing Techniques
- Transformation of Functions: Understanding how shifts, stretches, compressions, and reflections affect the graph of a function is essential. These transformations allow you to quickly sketch graphs based on known parent functions.
- Asymptotes and Discontinuities: For rational functions or more complex equations, identifying vertical and horizontal asymptotes can help in understanding the long-term behavior of the function.
- Using Technology: Graphing calculators and software tools can provide a more accurate representation of functions, especially when working with complicated expressions or higher-degree polynomials.
By mastering these graphing techniques, you can gain deeper insights into the behavior of mathematical functions, making problem-solving more intuitive and less time-consuming.
Factoring Methods and Practice
Factoring is a fundamental technique used to break down complex expressions into simpler components. Whether you’re working with polynomials or algebraic expressions, mastering factoring methods can make problem-solving much more manageable. By understanding different factoring strategies, you can efficiently find the solutions to various equations, from basic quadratics to higher-degree expressions.
Different types of polynomials require specific approaches for factoring. It’s important to recognize when to apply methods like factoring by grouping, using the difference of squares, or factoring trinomials. By practicing these techniques, you can gain confidence in your ability to factor any given expression accurately and quickly.
Common Factoring Techniques
- Factoring by Grouping: This method works well for polynomials with four terms. Group terms in pairs, factor out the greatest common factor (GCF) from each group, and factor the remaining binomial.
- Difference of Squares: This is a special case where expressions like a² – b² can be factored as (a + b)(a – b). Recognizing this form can simplify problems significantly.
- Factoring Trinomials: For quadratics, look for two numbers that multiply to give the product of the first and last terms and add up to the middle term. This helps split the trinomial into two binomials.
- Perfect Square Trinomial: If a trinomial is in the form of a² ± 2ab + b², it can be factored as (a ± b)².
Practice Problems
- Factor the polynomial: x² + 5x + 6
- Factor by grouping: 2x² + 8x + 3x + 12
- Factor the difference of squares: x² – 9
- Factor the trinomial: x² – 7x + 12
By applying these factoring methods regularly, you can improve your skills and tackle increasingly complex expressions with ease. Make sure to practice consistently and recognize the different types of polynomials you may encounter, as each requires its own approach.
Functions and Their Applications Explained
Functions are a core concept in mathematics that describe the relationship between variables. By mapping an input to an output, they allow us to model real-world phenomena, solve problems, and make predictions. Understanding the behavior of different types of functions is crucial, as it enables us to apply them to a variety of scenarios, from physics and engineering to economics and biology.
Functions can be represented in various forms, such as equations, graphs, or tables. By analyzing these representations, you can identify important characteristics of the function, such as its domain, range, and key points of interest. Whether you’re dealing with linear, quadratic, or more complex functions, recognizing their patterns and applications is essential for solving problems effectively.
Types of Functions and Their Uses
Different types of functions have specific applications depending on their characteristics. Below are some of the most commonly used functions and how they are applied:
| Function Type | Application |
|---|---|
| Linear Functions | Used to model constant rates of change, such as speed, cost, or temperature variations. |
| Quadratic Functions | Applied in projectile motion, area calculations, and problems involving acceleration. |
| Exponential Functions | Commonly used to model growth or decay, such as population growth, radioactive decay, or interest rates. |
| Logarithmic Functions | Used in measuring sound intensity, earthquake magnitude, and data compression algorithms. |
Understanding Real-World Applications
By understanding how different functions behave, you can apply them to solve practical problems. For instance, a quadratic function can help calculate the optimal height for launching a rocket, while an exponential function can model the growth of a population over time. These applications make the study of functions not only valuable in academic settings but also crucial for real-life decision-making and analysis.
In conclusion, mastering functions and their applications allows you to interpret and solve a wide range of problems across various fields. Whether you’re calculating the trajectory of a ball, determining the most efficient path in logistics, or analyzing market trends, understanding how to use and manipulate functions is a key skill in mathematics and beyond.
Key Theorems You Should Remember
Mathematics relies heavily on a set of foundational principles that guide problem-solving and analysis. These key theorems provide a structured approach to understanding relationships between variables, simplifying complex expressions, and solving equations. Familiarity with these theorems is essential for tackling a wide range of mathematical problems with confidence.
Each theorem serves as a tool to handle specific types of problems, from simplifying expressions to solving equations and analyzing graphs. Whether you’re working with polynomials, rational functions, or inequalities, these essential theorems will be your guide in breaking down problems and arriving at accurate solutions.
The Fundamental Theorem of Algebra
The Fundamental Theorem of Algebra states that every non-constant polynomial equation has at least one solution in the complex number system. This theorem assures that polynomial equations, no matter how complex, always have a root, which is a key concept for solving higher-degree polynomials.
The Remainder Theorem
The Remainder Theorem provides a straightforward method for evaluating polynomials. According to this theorem, when a polynomial is divided by a binomial of the form (x – c), the remainder of the division is equal to the value of the polynomial at x = c. This is useful for quickly finding values and simplifying polynomial division.
The Factor Theorem
The Factor Theorem is closely related to the Remainder Theorem. It states that if a polynomial f(x) is divided by (x – c) and the remainder is zero, then (x – c) is a factor of f(x). This theorem allows you to factorize polynomials by finding their roots, facilitating easier simplification and solving.
The Zero Product Property
The Zero Product Property is a crucial theorem for solving equations. It asserts that if the product of two factors equals zero, at least one of the factors must be zero. This property is essential when solving equations involving products of binomials or higher-degree polynomials.
The Binomial Theorem
The Binomial Theorem provides a formula for expanding expressions that are raised to a power, such as (a + b)^n. This theorem allows for the systematic expansion of binomial expressions, making it easier to work with powers of sums in various mathematical contexts.
Remembering and applying these key theorems will not only enhance your problem-solving abilities but also deepen your understanding of mathematical concepts. By mastering these principles, you will be better prepared to tackle a wide range of mathematical challenges, both in academics and in practical applications.
Breaking Down Complex Word Problems
Word problems often present a challenge due to their detailed descriptions and the need to translate real-world scenarios into mathematical equations. The key to solving these problems lies in breaking them down into smaller, manageable parts. By identifying the relevant information, setting up an equation, and applying appropriate mathematical methods, you can systematically work towards a solution.
The first step in solving word problems is to carefully read the question and highlight the important data provided. This helps to avoid confusion and ensures that you’re focusing on the necessary details. Next, it’s crucial to translate the words into a mathematical form, such as an equation or function, based on the context of the problem. Once the problem is structured mathematically, you can apply algebraic techniques or other methods to find the solution.
In many cases, word problems also involve multiple steps. For instance, you may need to solve for an unknown variable in one part of the problem, and then use that result in another part. Keeping track of each step and checking your work along the way is essential for ensuring accuracy and avoiding mistakes.
By practicing these strategies, you will develop the skills needed to tackle even the most complex word problems with confidence. The more you practice, the more efficient and effective you will become at interpreting and solving these challenges.
Reviewing Sample Questions and Solutions
One of the most effective ways to prepare for mathematical challenges is by reviewing a variety of example problems and their solutions. This practice allows you to familiarize yourself with common question formats, identify patterns, and understand how to apply mathematical principles to solve real-world problems. Analyzing both correct and incorrect solutions helps you to refine your approach and avoid common mistakes.
Each sample question provides an opportunity to break down the problem-solving process step by step. Begin by carefully reading through the problem, identifying key information, and translating it into a mathematical framework. Once the equation or system is established, applying the appropriate techniques will guide you to the solution. Reviewing the reasoning behind each step is crucial to grasping the overall method used to reach the final result.
By practicing with diverse sample problems, you not only reinforce your understanding of core concepts but also build your confidence. Regularly revisiting solutions and revising your approach will strengthen your problem-solving abilities and prepare you for future challenges.
Understanding Transformations and Shifting
In mathematics, understanding how functions and graphs change when manipulated is crucial. These changes, often referred to as transformations, involve shifting, stretching, or reflecting the graph of a function. By mastering these techniques, you can predict how any given function will behave under various operations, making it easier to solve complex problems.
Transformations are generally classified into several types, each affecting the graph in a different way. These include:
- Vertical Shifts: Moving the graph up or down by a constant value.
- Horizontal Shifts: Moving the graph left or right along the x-axis.
- Reflections: Flipping the graph across the x-axis or y-axis.
- Stretching and Shrinking: Expanding or compressing the graph vertically or horizontally.
For instance, adding a constant to the function will shift the graph vertically, while altering the coefficient of the variable inside the function will affect its horizontal positioning. These operations allow for the transformation of functions from their original state into new forms that are easier to analyze or solve.
By practicing these transformations and learning how each one influences the graph, you can simplify and solve a wide variety of mathematical problems. The ability to visualize these changes helps in understanding more complex functions and their behaviors in different scenarios.
Advanced Topics in Chapter 3 Exam
As you progress to more complex mathematical challenges, the problems become less straightforward, requiring a deeper understanding of concepts and techniques. In this section, we explore the advanced topics that are often encountered in exams, focusing on the more intricate aspects that require critical thinking and problem-solving skills. Mastering these topics will not only enhance your ability to solve difficult problems but will also help you approach various functions and equations with greater confidence.
Some of the more advanced concepts that often appear in this section include:
- Nonlinear Equations: Working with equations that do not form straight lines, requiring specialized methods like factoring, completing the square, or applying the quadratic formula.
- Complex Functions: Understanding functions that involve multiple variables or more complex expressions, and how to manipulate them to find solutions.
- Polynomial Long Division: A method used to divide polynomials, which is essential for simplifying expressions and solving higher-degree equations.
- Inverse Functions: The concept of finding the inverse of a given function, which can reverse the operations performed by the original function.
- Logarithmic and Exponential Functions: These types of functions require a good understanding of their properties and how they interact with different transformations.
Each of these advanced topics builds upon foundational concepts, yet they demand a more nuanced approach. Familiarity with these areas will allow you to tackle more difficult questions with ease and precision. The ability to handle such complex material will ensure success in exams and provide a solid foundation for future studies in mathematics.
Final Review: What to Focus On
As you approach the final stage of your preparation, it’s crucial to consolidate your understanding and identify the key areas that will most likely appear on assessments. This section highlights the fundamental concepts that require special attention, ensuring that you are fully equipped to tackle the more challenging problems. By focusing on these core topics, you will be able to streamline your review and approach the exam with confidence.
Key Concepts to Master
Focus your efforts on the following areas that frequently pose challenges but are essential for achieving a strong performance:
- Function Manipulation: Ensure that you are comfortable working with various types of functions, including linear, quadratic, and polynomial. Understand how to perform operations such as addition, subtraction, multiplication, and division of functions.
- Graph Interpretation: Being able to analyze and interpret graphs is a critical skill. Pay particular attention to transformations, shifts, and reflections, as well as identifying key features like intercepts and asymptotes.
- Solving Equations: Whether linear or more complex, solving equations will be a significant part of the material. Brush up on solving systems of equations, factoring, and using appropriate methods for each type of equation.
- Exponential and Logarithmic Functions: These functions often challenge students due to their unique properties. Practice how to manipulate and solve problems involving both exponential growth and decay, as well as logarithmic equations.
Test-Taking Strategies
In addition to reviewing the core material, be sure to implement effective test-taking strategies. Time management is crucial, so practice answering questions under timed conditions to increase your speed and accuracy. Read each question carefully and break down complex problems into smaller, manageable steps. Prioritize the questions you feel most confident about, and leave more challenging ones for later.
By focusing on these key concepts and honing your test-taking skills, you’ll be ready to perform at your best. Be thorough in your review, but also trust your preparation and maintain a calm, focused mindset during the exam.