AP Statistics Unit 4 Test Answer Key

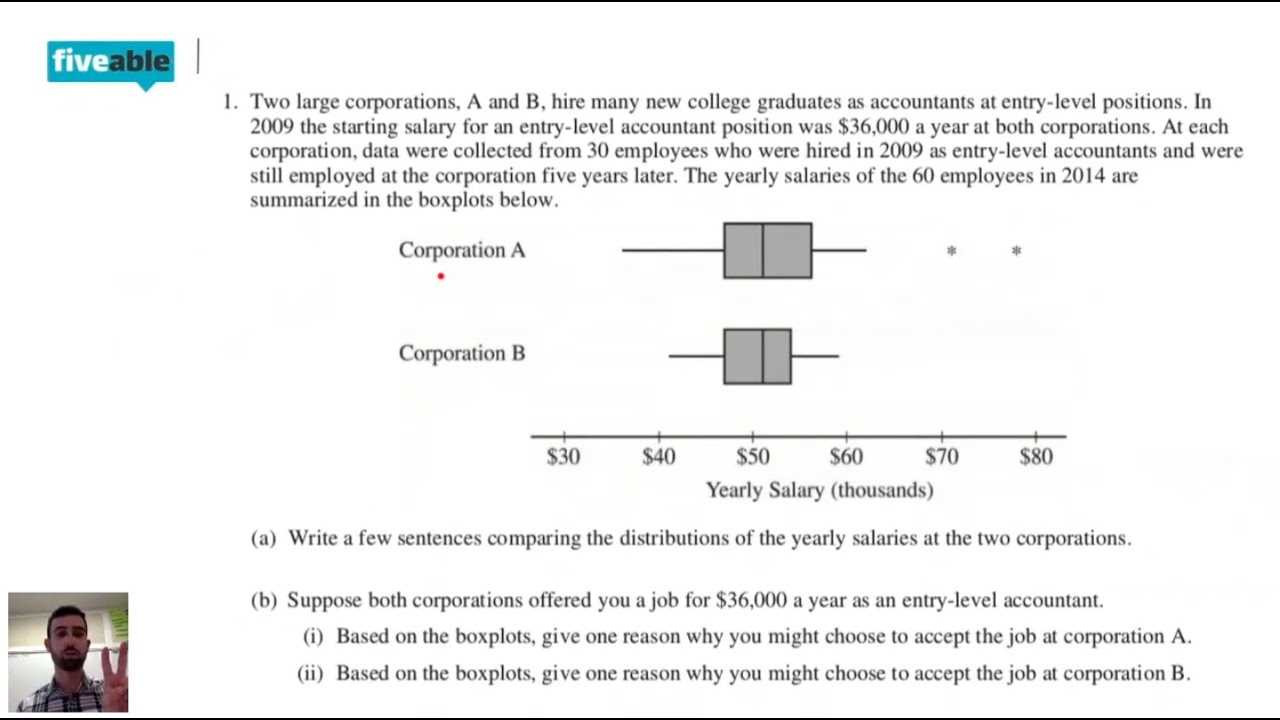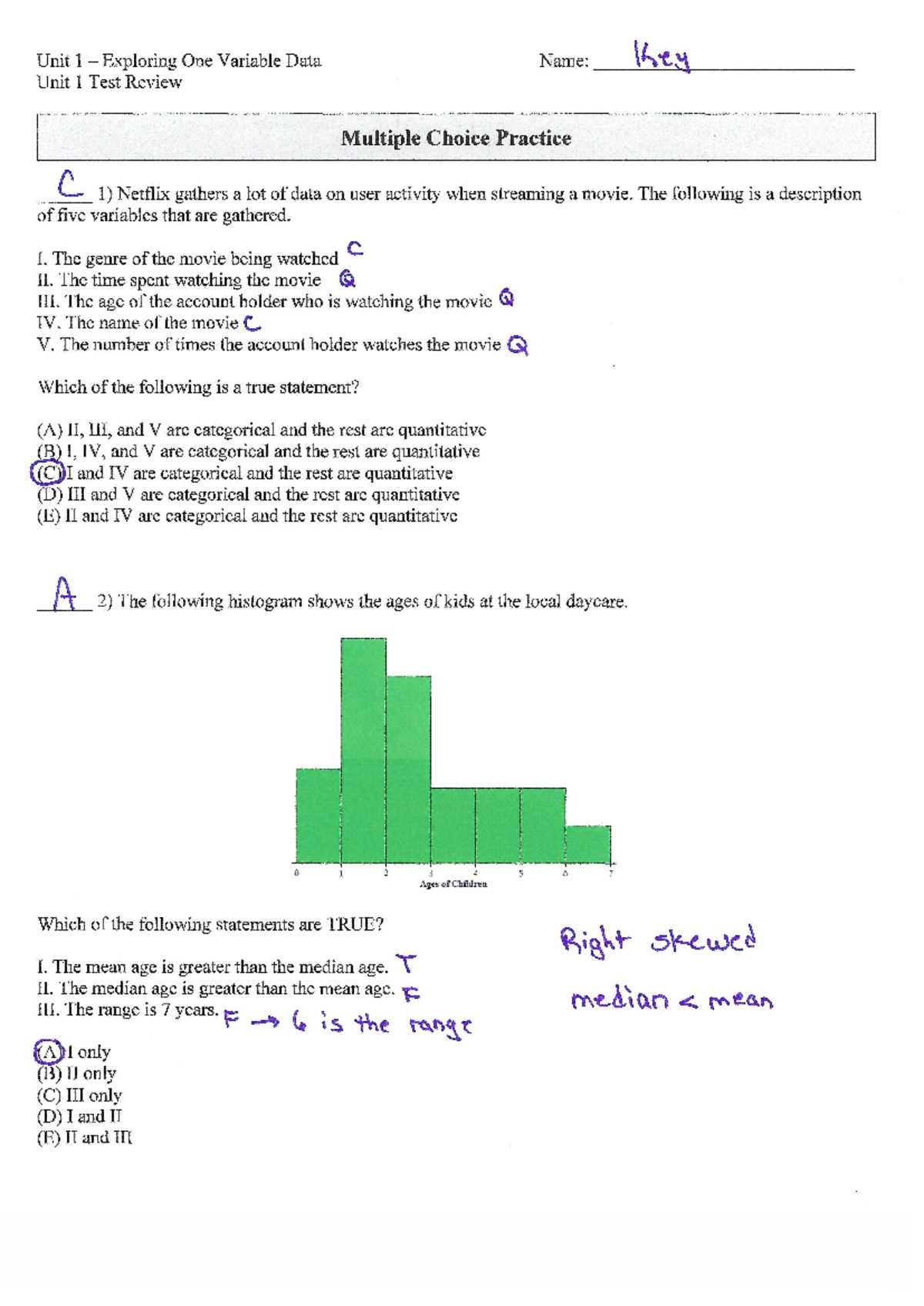
As you progress through your studies in advanced mathematics, mastering the concepts of probability, inference, and data analysis becomes crucial. This section will guide you through the essential skills and techniques needed to approach challenging problems with confidence. Understanding the core principles and applying them correctly is key to performing well in any assessment.

The focus here is on building a solid foundation in methods that help interpret data, draw conclusions, and solve real-world problems. Whether you’re reviewing concepts like margin of error or understanding complex distributions, a clear grasp of these areas will significantly improve your performance. By following structured steps and practicing problem-solving, you can navigate the more intricate aspects of the subject with ease.

AP Advanced Topics Assessment Guide

In this section, you’ll find essential resources to help you review and understand the solutions to the more complex problems in your recent evaluation. These insights will guide you through the process of solving intricate questions related to probability, data interpretation, and analysis. Mastering these concepts is crucial for excelling in higher-level assessments and ensures you are well-prepared for future challenges.

Each solution is accompanied by a detailed explanation that breaks down the steps required to arrive at the correct conclusion. By analyzing these examples, you can better grasp how to approach similar problems on your own. Whether you’re focused on constructing confidence intervals or evaluating hypothesis tests, understanding the underlying methods and logic is key to success.

Important Techniques: Pay close attention to how the concepts are applied across different scenarios. With careful practice and a strong understanding of these methods, you will be able to tackle even the most complex problems with confidence and accuracy.

Overview of Unit 4 Topics

In this section, we explore the core concepts that will help you master the more advanced aspects of mathematical analysis. You will dive deep into the techniques used for understanding data patterns, drawing conclusions, and assessing uncertainty in various contexts. This section is designed to provide you with a solid foundation for handling both theoretical problems and practical applications.

Key Concepts Covered

• Probability theory: Understanding the likelihood of events and the fundamentals of probability distributions.
• Confidence intervals: Estimating population parameters and understanding the margin of error.
• Hypothesis testing: Learning how to evaluate claims based on sample data and statistical significance.
• Distributions: Analyzing different data sets, including normal and binomial distributions.

Skills You Will Develop

• Problem-solving strategies: Mastering the techniques to approach complex scenarios and ensure accurate calculations.
• Data interpretation: Learning how to interpret and present results clearly to draw meaningful conclusions.
• Practical applications: Using these concepts in real-world situations to make informed decisions based on data.

Understanding Probability Concepts

Probability plays a fundamental role in predicting outcomes and understanding uncertainty. Whether you’re analyzing the chances of an event occurring or assessing the likelihood of various outcomes, a strong grasp of probability concepts is essential. By learning how to calculate and interpret probabilities, you’ll be able to make informed decisions based on the available data.

At its core, probability involves assessing the likelihood of specific events, which can range from simple scenarios like flipping a coin to more complex situations involving multiple variables. Understanding how to calculate these probabilities and use them in different contexts will provide valuable insights, whether you are working with theoretical models or real-world applications.

Key Concepts:

• Event probability: The likelihood that a particular event will occur based on the possible outcomes.
• Conditional probability: The probability of one event occurring given that another has already happened.
• Independent and dependent events: Understanding how different events influence one another and their impact on probability calculations.
• Probability distributions: A function that describes the likelihood of each possible outcome in an experiment.

Interpreting Confidence Intervals

Confidence intervals provide a range of values within which we expect a population parameter to fall, based on sample data. Understanding how to interpret these intervals is crucial for assessing the precision of estimates and making informed decisions. They offer a way to quantify uncertainty and are commonly used in decision-making processes where exact values are unknown but estimates are required.

The width of the interval indicates the level of confidence we have in our estimate–wider intervals suggest more uncertainty, while narrower ones imply more precision. A 95% confidence interval, for example, means that if the sampling process were repeated many times, approximately 95% of the intervals would contain the true population parameter.

To interpret these intervals correctly, it’s important to consider the context, sample size, and confidence level. A properly constructed interval provides valuable information about the range of likely values and helps in evaluating the reliability of conclusions drawn from data.

Calculating Margin of Error

The margin of error is a critical value that helps quantify the uncertainty in an estimate. It provides a range within which the true population parameter is likely to fall. This concept is especially important when interpreting sample data, as it allows you to understand the precision of the results. A smaller margin of error indicates more confidence in the estimate, while a larger margin suggests greater uncertainty.

Steps to Calculate Margin of Error

• Identify the sample size: Larger sample sizes typically lead to more accurate estimates, thus reducing the margin of error.
• Find the critical value: This value depends on the desired confidence level, typically found using a standard normal distribution.
• Calculate the standard deviation: Use the sample data to estimate the variability, which will affect the margin of error.
• Apply the formula: Multiply the standard error by the critical value to obtain the margin of error.

Importance of the Margin of Error

The margin of error is essential in determining how much confidence you can place in your sample estimate. It helps to communicate the level of uncertainty in your results and is a key factor in decision-making processes. Understanding how to calculate and interpret this value can enhance the reliability of conclusions drawn from sample data.

Key Formulae for Unit 4 Tests

In any mathematical analysis, having a set of reliable formulas is essential for solving complex problems efficiently. In this section, we’ll cover the critical formulas you’ll need to navigate through key topics. Understanding how and when to apply these equations is fundamental to achieving accurate results, especially when dealing with probability, data estimation, and hypothesis evaluation.

Important Formulas to Know

• Confidence Interval Formula:

  CI = sample mean ± (critical value) × (standard error)

  Used to estimate the range of possible values for a population parameter based on sample data.

• Margin of Error Formula:

  Margin of Error = (critical value) × (standard error)

  A measure of the uncertainty associated with sample estimates.

• Standard Error Formula:

  SE = standard deviation / √n

  Helps determine the precision of a sample estimate by measuring variability.

• Z-Score Formula:

  Z = (sample mean – population mean) / standard error

  Used to assess how far a sample mean is from the population mean in terms of standard errors.

• Hypothesis Testing Formula:

  Z = (sample mean – hypothesized mean) / (standard deviation / √n)

  Helps in evaluating whether a sample data point supports or refutes a hypothesis.

How to Use These Formulas

• Apply the correct formula: Choose the appropriate formula based on the problem type, whether you are estimating values or testing hypotheses.
• Check units and values: Ensure that all values, like the sample size and standard deviation, are in the correct units and properly substituted into the formula.
• Interpret the results: After applying the formulas, interpret the outcomes in the context of the problem, considering the implications of the confidence intervals, error margins, and hypothesis test results.

Common Mistakes on the Test

Even when you have a strong understanding of the material, it’s easy to make mistakes during an assessment. These errors can range from simple miscalculations to misunderstanding key concepts. Recognizing common pitfalls can help you avoid them and improve your accuracy when answering questions. In this section, we’ll highlight frequent mistakes students make and offer tips on how to steer clear of them.

Frequent Errors to Watch For

Common Mistake	Why It Happens	How to Avoid It
Misinterpreting the question	Failing to carefully read the problem leads to wrong assumptions.	Read each question thoroughly and underline key terms.
Incorrect use of formulas	Forgetting to apply the right formula or using incorrect values.	Always review the formulae before applying them and check units.
Overlooking the sample size	Ignoring sample size when calculating error margins or confidence intervals.	Ensure the sample size is properly factored into all calculations.
Rounding too early	Rounding intermediate values instead of only the final result.	Perform all calculations without rounding until the final step.
Confusing critical values	Using the wrong z-score or t-value based on the confidence level.	Double-check the critical value from the correct distribution table.

How to Minimize Mistakes

To minimize errors, it’s important to practice regularly and develop a systematic approach to each problem. Taking time to understand the problem, applying the appropriate formula correctly, and double-checking calculations can significantly reduce mistakes. Additionally, practicing under timed conditions will help you manage your time more efficiently during the assessment.

Review of Sample Questions

Working through sample problems is a powerful way to reinforce understanding and sharpen skills for any evaluation. By tackling various examples, you can familiarize yourself with the problem types and ensure you are prepared to handle the concepts effectively. In this section, we will go over a selection of practice questions, offering solutions and tips for addressing similar challenges during your assessment.

Example Question 1: Estimating Parameters

A sample of 200 people shows an average age of 30 years, with a standard deviation of 8 years. You are asked to calculate a 95% confidence interval for the true mean age of the population.

• Step 1: Identify the critical value for a 95% confidence level, which is approximately 1.96 for a normal distribution.
• Step 2: Compute the standard error: SE = 8 / √200 ≈ 0.566.
• Step 3: Apply the formula:
  CI = 30 ± (1.96 × 0.566) = (28.87, 31.13).
• Step 4: Interpret the result: With 95% confidence, the true mean age of the population is between 28.87 and 31.13 years.

Example Question 2: Hypothesis Testing for Means

A company claims that their new product lasts an average of 500 hours. A sample of 50 products is tested, with a mean lifetime of 490 hours and a standard deviation of 25 hours. Conduct a hypothesis test at the 0.05 significance level to evaluate if the product’s lifetime differs from the claimed average.

• Step 1: Set up hypotheses:
  Null hypothesis: μ = 500, Alternative hypothesis: μ ≠ 500.
• Step 2: Calculate the test statistic:
  t = (490 – 500) / (25 / √50) ≈ -2.83.
• Step 3: Find the critical t-value for a two-tailed test with 49 degrees of freedom (t-critical ≈ ±2.009).
• Step 4: Compare the test statistic to the critical value. Since -2.83 is outside the range of -2.009 and 2.009, we reject the null hypothesis and conclude that the product’s lifetime is significantly different from the claimed average.

These sample questions demonstrate how to approach different problem types. Practicing with these examples can enhance your ability to analyze data, apply formulas, and make informed decisions during the actual assessment.

Steps to Solve Probability Problems

Solving probability problems involves a series of logical steps that allow you to calculate the likelihood of different outcomes. By following a systematic approach, you can break down complex problems and apply the appropriate formulas and methods to arrive at accurate solutions. This section will outline the key steps you should follow when working with probability scenarios.

Step 1: Understand the Problem

Before beginning any calculations, carefully read the problem to understand the scenario. Identify the key information, such as the total number of possible outcomes, the number of favorable outcomes, and the event you are interested in. Clarify whether the problem involves independent or dependent events, as this will influence the method you use to calculate probabilities.

Step 2: Identify the Type of Probability

Determine which type of probability you need to calculate. There are different methods based on the problem type, such as:

• Simple Probability: When you are calculating the likelihood of a single event happening. This can be found by dividing the number of favorable outcomes by the total number of outcomes.
• Compound Probability: When two or more events are involved, and you need to find the likelihood of them happening together or separately. In this case, you will either multiply or add probabilities based on whether the events are independent or mutually exclusive.
• Conditional Probability: When the probability of one event depends on the outcome of another event. This requires using the conditional probability formula, typically represented as P(A|B).

Step 3: Apply the Relevant Formula

Once you’ve identified the type of probability, apply the correct formula. For simple probability, use:

P(A) = (Number of favorable outcomes) / (Total number of outcomes)

For compound events, use multiplication or addition rules depending on whether the events are independent or dependent. For example:

• P(A and B) = P(A) × P(B) for independent events
• P(A or B) = P(A) + P(B) – P(A and B) for mutually exclusive events

Step 4: Solve and Interpret the Result

After applying the formula, calculate the final probability. Make sure your result is in the correct range, as probabilities should always be between 0 and 1. Finally, interpret the result in the context of the problem, explaining what the probability means in terms of the event occurring.

By following these steps, you can approach probability problems with clarity and confidence, ensuring that you arrive at the correct solution every time.

Interpreting Hypothesis Testing Results

Understanding the outcomes of hypothesis evaluations is a crucial step in making informed decisions based on data. Once you have conducted a hypothesis evaluation, interpreting the results involves analyzing various statistical indicators, such as the test statistic, p-value, and confidence intervals, to draw meaningful conclusions. The interpretation helps to determine whether the data supports or contradicts a given claim or assumption.

Key Elements in Interpretation

When reviewing the results, focus on the following critical components to help guide your interpretation:

• Test Statistic: This value quantifies the difference between your sample data and the null hypothesis. A larger value typically indicates stronger evidence against the null hypothesis.
• P-value: The p-value represents the probability of observing data as extreme as, or more extreme than, the current sample, assuming the null hypothesis is true. A smaller p-value suggests stronger evidence to reject the null hypothesis.
• Significance Level (α): The pre-established threshold for deciding whether to reject the null hypothesis. Common values for α are 0.05, 0.01, and 0.10.
• Confidence Interval: A range of values that is likely to contain the true parameter. If the confidence interval excludes the null hypothesis value, it provides evidence against the null hypothesis.

Decision Making Based on Results

Once you have reviewed the test statistic, p-value, and other relevant indicators, you can draw conclusions about your hypothesis:

• Rejecting the Null Hypothesis: If the p-value is less than the significance level (e.g., p
• Failing to Reject the Null Hypothesis: If the p-value is greater than the significance level (e.g., p > 0.05), you fail to reject the null hypothesis. This means there is insufficient evidence to support the alternative hypothesis.
• Making Informed Decisions: Interpreting the results correctly enables you to make informed decisions based on data. It’s important to consider the context and other factors that may influence the outcome, such as sample size and assumptions made during the evaluation.

By understanding and accurately interpreting hypothesis testing results, you can ensure that your conclusions are both valid and reliable, leading to more confident decisions and actions based on data.

Analyzing Data Using Normal Distributions

When examining large datasets, a common approach is to look for patterns that emerge in the distribution of values. Many datasets naturally follow a bell-shaped curve, which reveals key insights about the data’s central value and the variation around it. This type of distribution allows for efficient analysis and prediction, as it provides a framework for determining the likelihood of various outcomes. By understanding how data points are spread around the mean, one can make informed decisions based on probability and trends within the data.

Characteristics of Normal Distributions

Normal distributions have several defining features that make them useful for analysis:

• Symmetry: A normal distribution is symmetric around its center, meaning the data points on the left and right are mirror images of each other.
• Central Tendency: The mean represents the central value of the data, and it is located at the peak of the curve.
• Spread: The spread of the data is determined by the standard deviation, which shows how much individual data points deviate from the mean.
• Empirical Rule: In a normal distribution, approximately 68% of the data falls within one standard deviation of the mean, 95% within two, and 99.7% within three.

Steps to Analyze Data

To analyze a dataset using a normal distribution, follow these essential steps:

1. Step 1: Calculate the Mean and Standard Deviation: Begin by determining the mean (average) and standard deviation, as they provide a sense of the data’s central tendency and variation.
2. Step 2: Standardize the Data: Convert individual data points into Z-scores, which standardize them relative to the mean and standard deviation. This is done using the formula: Z = (X – μ) / σ, where X is a data point, μ is the mean, and σ is the standard deviation.
3. Step 3: Refer to the Z-Table: After calculating the Z-score, use a Z-table to find the cumulative probability. This will give the likelihood that a data point is less than or equal to a specific value.

Example Calculation

Let’s consider a set of scores that follows a normal distribution with a mean of 75 and a standard deviation of 8. To find the probability of a score greater than 85, follow these steps:

Comparing Different Statistical Methods

When analyzing data, it’s important to understand the various approaches available for interpreting results. Different techniques offer unique advantages depending on the nature of the data and the type of insights you’re seeking. These methods may vary in terms of complexity, assumptions, and the kind of conclusions they allow. By comparing different approaches, you can make more informed decisions about which method best suits your analysis and objectives.

Descriptive vs. Inferential Approaches

Two primary categories of data analysis are descriptive and inferential methods. While both help summarize and interpret data, they serve distinct purposes:

• Descriptive methods are used to summarize and describe the main features of a dataset. They help provide a clear picture of the data’s central tendency, spread, and patterns. Examples include calculating averages, medians, and standard deviations.
• Inferential methods go beyond mere description by making predictions or inferences about a population based on a sample. These techniques often rely on probability theory and hypothesis testing. Common examples include regression analysis and confidence intervals.

Which Method to Use?

The choice between descriptive and inferential methods depends largely on your goals:

• If you’re looking to simply summarize a dataset, descriptive methods will provide a clear and concise overview.
• If your aim is to make predictions or draw conclusions about a larger population from a sample, inferential techniques are more suitable.

Both approaches can be valuable in different contexts. However, understanding the strengths and limitations of each method is crucial to ensure accurate and meaningful interpretations of your data.

Understanding Type I and II Errors

In any form of hypothesis testing, the goal is to make a decision based on available data. However, due to the inherent uncertainty in making these decisions, errors can occur. Understanding the two primary types of errors–Type I and Type II–is essential for correctly interpreting results and minimizing the risk of drawing incorrect conclusions.

Type I Error: False Positive

A Type I error occurs when a hypothesis test incorrectly rejects a true null hypothesis. In other words, it is a false positive. This means that the test suggests there is an effect or relationship when, in reality, none exists. The probability of committing a Type I error is known as the significance level, often denoted by alpha (α).

• Example: Concluding that a new drug is effective when it actually has no effect.
• Consequence: A Type I error may lead to unnecessary actions or decisions based on incorrect findings.

Type II Error: False Negative

A Type II error happens when a hypothesis test fails to reject a false null hypothesis. This is known as a false negative. In this case, the test misses an effect or relationship that actually exists. The probability of a Type II error is represented by beta (β), and it is often related to the power of the test.

• Example: Concluding that a new drug is ineffective when it actually works.
• Consequence: A Type II error can result in missed opportunities, as important findings or innovations may be overlooked.

Both types of errors have significant implications, and understanding their potential risks can help in designing more robust tests and making more reliable conclusions from data.

Exam Tips for AP Statistics

Preparing for any assessment requires a strategic approach to ensure success. For those facing a challenging exam that tests the understanding of data analysis and probability concepts, having the right techniques and mindset can make a significant difference. Below are some essential strategies that can help boost performance and manage the exam effectively.

1. Master the Core Concepts

Understanding the foundational principles of data collection, analysis, and interpretation is critical. Familiarize yourself with key topics such as distributions, confidence intervals, and hypothesis testing. Having a solid grasp of these concepts will allow you to approach various problems with confidence and clarity.

2. Practice with Real-World Problems

Apply what you’ve learned by working through real-world examples. By solving problems that require data interpretation and statistical methods, you build problem-solving skills and become more comfortable with the types of questions that may appear on the exam.

3. Review Past Assessments

Reviewing previous assessments or practice exams can provide insight into the format and structure of the exam. This will help you understand the types of questions asked, and identify common patterns. It also gives you a chance to pinpoint areas where you may need additional study or practice.

4. Time Management

Effective time management is essential during the exam. Break down your study sessions into focused intervals and prioritize topics based on their importance. During the exam, ensure that you allocate enough time for each section without rushing through any part.

5. Use the Calculator Wisely

The calculator is an essential tool for efficiently solving complex problems. Be sure to know how to use it for tasks such as finding z-scores, calculating probabilities, and performing regression analysis. Familiarity with the calculator’s features will save valuable time during the exam.

6. Stay Calm and Confident

Finally, maintaining a calm and focused mindset can significantly enhance performance. Remember, the exam is designed to assess your understanding of key concepts, not to overwhelm you. Take deep breaths, read each question carefully, and approach every problem methodically.

By following these tips, you will be well-equipped to approach the assessment with confidence and maximize your chances for success.

Using Technology for Calculations

In modern assessments, leveraging technological tools can significantly enhance the efficiency and accuracy of performing complex calculations. Whether it’s handling large datasets or performing intricate mathematical operations, the right technology allows you to streamline the process and focus on interpreting results. Below are several ways technology can aid in solving problems and improve your overall performance.

1. Graphing Calculators

Graphing calculators are essential tools for visualizing data distributions and functions. These devices enable users to plot graphs, perform regressions, and find areas under curves. By using graphing calculators, you can quickly calculate probabilities, visualize trends, and make informed decisions about the data you’re working with.

2. Statistical Software

Statistical software programs like R, Python (with libraries like NumPy and Pandas), and SPSS offer more advanced capabilities for analyzing large sets of data. These tools can automate calculations, perform hypothesis tests, and generate various statistical plots. Mastering these platforms can save significant time, especially when dealing with complex calculations that are too tedious to perform manually.

3. Online Calculators and Apps

Online calculators and mobile apps provide a quick and accessible way to perform common calculations. These apps can handle everything from basic arithmetic to more advanced operations like finding z-scores or confidence intervals. Many apps also include step-by-step solutions, making them valuable resources for understanding the process behind each calculation.

4. Spreadsheets

Spreadsheets such as Microsoft Excel or Google Sheets can be used to perform a variety of tasks, from simple calculations to more complex data manipulations. By entering data into a spreadsheet, you can use built-in functions like SUM, AVERAGE, and STDEV to compute essential metrics quickly. Spreadsheets also allow you to easily create visual representations of data through charts and graphs, helping you better understand trends and relationships within the dataset.

5. Interactive Learning Tools

Interactive learning tools and simulators can provide a hands-on approach to understanding complex mathematical concepts. These platforms often include real-time simulations that allow you to manipulate variables and observe how changes affect outcomes. Such tools are especially useful for developing a deeper understanding of probability and distributions.

By mastering these technological resources, you can not only improve your ability to perform calculations but also enhance your overall understanding of how mathematical concepts are applied in real-world scenarios. Whether you’re working with small data sets or large datasets, technology is an invaluable asset for efficient problem-solving.

How to Prepare for Unit 4 Exam

To perform well in this upcoming examination, it’s essential to approach your preparation with a clear strategy. Focusing on mastering the core principles, practicing problem-solving techniques, and managing your time effectively are all crucial components of successful preparation. By organizing your study sessions and reviewing key concepts thoroughly, you will feel confident and capable during the assessment.

Review Key Concepts and Formulas

Begin by revisiting the fundamental principles that are central to the material covered. Make sure you understand the key concepts and formulas, as these will form the foundation for solving problems during the exam. Consider creating summary sheets or flashcards to help you quickly recall essential information. This process will solidify your understanding and ensure you’re well-equipped for any related questions.

Practice with Sample Problems

Consistent practice is one of the most effective ways to prepare. Work through a variety of sample problems to familiarize yourself with the types of questions you may encounter. Focus on both straightforward and complex problems to ensure you’re prepared for any difficulty level. By practicing regularly, you’ll improve your problem-solving speed and accuracy, which is crucial when time is limited during the exam.

Incorporate mock exams into your preparation plan to simulate the actual exam environment. This will help you refine your time management skills and boost your confidence. It’s important to review both correct and incorrect answers after practicing to understand the reasoning behind each solution. With this targeted approach, you’ll be prepared to perform at your best on exam day.

Final Thoughts on Unit 4 Review

As you approach the final stages of your preparation, it’s important to reflect on the progress you’ve made and ensure you’re well-prepared for the upcoming evaluation. Focusing on your strengths while identifying areas that require additional review will help optimize your readiness. A thoughtful approach, including consistent practice and understanding the underlying principles, will serve as the foundation for success. Stay organized, and continue reinforcing key concepts to ensure confidence when the time comes to demonstrate your knowledge.

Consolidate Your Understanding

Make sure you’ve reviewed all the major concepts thoroughly and can apply them effectively to solve different types of problems. It’s crucial to go beyond memorization and truly understand how the concepts interconnect. This will allow you to handle complex questions with ease. If you have any lingering doubts, take time to address them and clarify your understanding through additional resources or discussions with peers or instructors.

Stay Calm and Confident

On the day of the evaluation, approach the session with a calm and focused mindset. Trust in the effort you’ve put into preparing, and approach each question methodically. Remember that thorough preparation reduces anxiety and enhances performance. Stay confident in your ability to tackle any challenge that arises, and maintain a positive outlook throughout the process.

By consolidating your knowledge and staying composed, you will be ready to tackle the challenges ahead and demonstrate the full scope of your understanding when it matters most.

Score