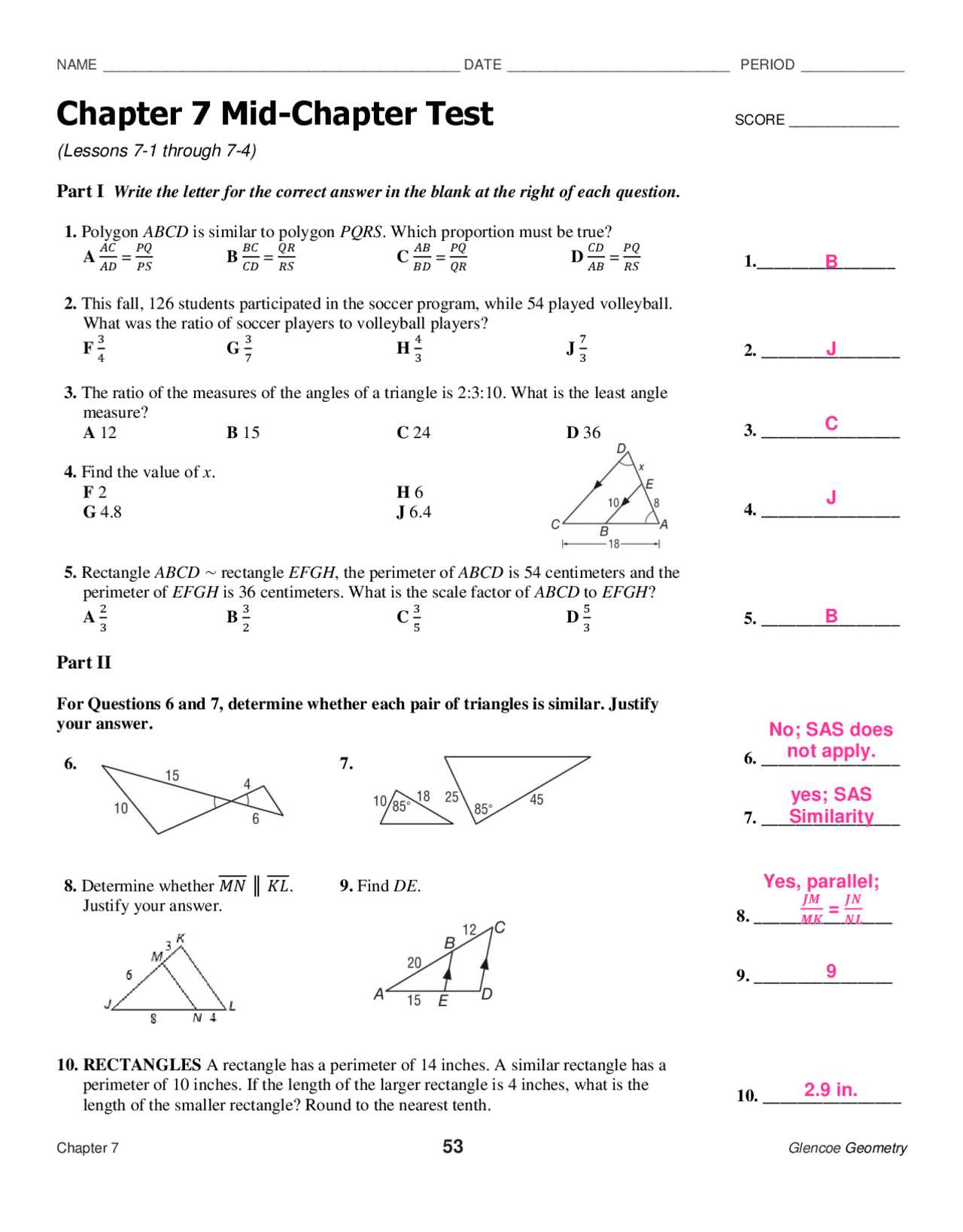
In this section, we focus on the essential principles and techniques that form the foundation of a particular area of study. The goal is to provide clear and structured explanations, helping learners build a strong understanding of the core concepts.
Whether you’re working through complex calculations or solving practical problems, the methods outlined here offer a step-by-step approach. By following these steps, students can gain confidence in tackling challenges and improve their problem-solving skills.
Practical exercises are included to help reinforce each concept, ensuring that learners can apply what they’ve learned effectively. Regular practice will solidify understanding and make it easier to handle more advanced topics in the future.
Chapter 7 Geometry Answers Overview
This section provides a comprehensive look at key problem-solving techniques and methods used in this unit. The focus is on ensuring a deeper understanding of the fundamental concepts, enabling students to confidently work through various exercises and challenges. By breaking down each problem, learners can grasp the necessary skills for tackling similar questions in future lessons.
Core Concepts Explained
The problems in this section are designed to test a wide range of skills, from basic calculations to more advanced logical reasoning. Understanding these core principles is crucial for mastering the subject, as they build upon one another and enhance the ability to solve complex issues. Each problem is approached with a clear, structured explanation that reinforces these ideas.
Problem Solving Strategies
In this section, you’ll find proven strategies to help guide you through each question. These methods are structured to simplify difficult concepts and make them more accessible, allowing students to progress more easily. Consistent practice and application of these techniques will lead to significant improvement in both speed and accuracy.
| Problem Type | Key Skills | Suggested Approach |
|---|---|---|
| Basic Calculations | Arithmetic operations, simplification | Follow order of operations, double-check results |
| Logical Reasoning | Pattern recognition, critical thinking | Break the problem into smaller parts, analyze patterns |
| Word Problems | Reading comprehension, algebraic manipulation | Identify key information, set up equations |
Understanding Key Geometry Concepts
This section aims to explore the fundamental ideas that form the backbone of spatial reasoning and problem-solving. These core principles are essential for developing a strong foundation, allowing learners to approach more complex topics with confidence. By mastering these concepts, students will be better equipped to tackle a variety of challenges effectively.
At the heart of this study are shapes, measurements, and relationships between angles and sides. Understanding how these elements interact enables a clearer approach to solving various types of problems. Grasping the significance of each concept is crucial for both theoretical understanding and practical application in real-world situations.
By reviewing the key terminology and visualizing how the elements fit together, learners can develop a more intuitive sense of how to approach problems. Regular practice and reflection on these concepts will ensure continuous improvement and mastery of the subject matter.
How to Approach Geometry Problems
Solving complex problems in this field requires a clear and methodical approach. The key to success lies in breaking down each problem into manageable parts and applying the right techniques to solve them. Developing a systematic approach not only improves accuracy but also enhances the ability to tackle increasingly difficult challenges.
Step-by-Step Breakdown
The first step in solving any problem is to carefully read and understand the question. Identify the key information and the unknowns, then visualize the situation through diagrams. Drawing a clear picture of the problem often provides valuable insights and makes the process of finding a solution easier.
Choosing the Right Method
Once the problem is understood, it’s important to choose the appropriate method for solving it. This might involve using formulas, applying logical reasoning, or breaking the problem into smaller, more manageable sub-problems. Practice will help in recognizing the most efficient strategies for different types of questions.
Step-by-Step Solutions for Geometry Questions
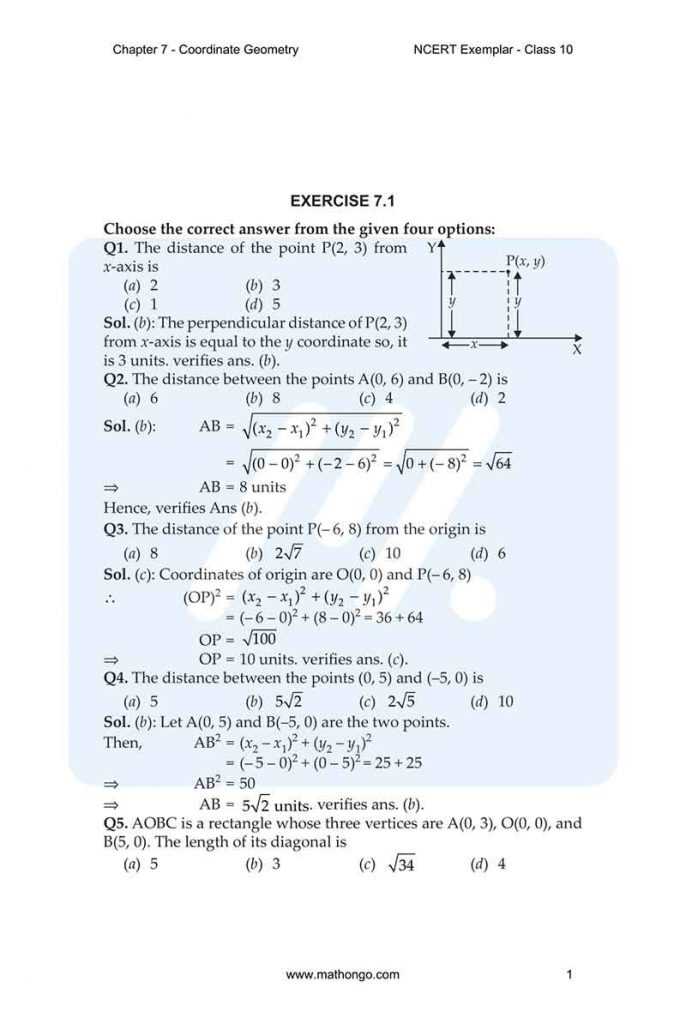
In this section, we will break down the problem-solving process into clear, manageable steps to help guide you through various questions. By following these systematic methods, students can understand how to approach each problem effectively and solve it with confidence.
Each solution follows a logical progression, ensuring that no steps are missed and every concept is explained in detail. Whether it’s a simple calculation or a more complex reasoning task, these steps can be applied to solve a variety of challenges.
Solution Process
- Read the Problem Carefully: Identify all the given information and highlight the unknowns.
- Draw a Diagram: If applicable, sketch the situation to visualize the relationships between elements.
- Choose the Appropriate Formula or Method: Select the most relevant approach based on the problem type.
- Work Through the Steps: Apply the chosen method, step by step, making sure to check each calculation along the way.
- Review and Verify: Double-check your work to ensure accuracy and consistency in the solution.
Example Problem Breakdown
Here is an example of how this step-by-step process can be applied:
- Problem: Calculate the area of a triangle with a base of 10 units and a height of 5 units.
- Solution:
- Step 1: Identify the given values (base = 10, height = 5).
- Step 2: Use the formula for the area of a triangle: Area = 1/2 * base * height.
- Step 3: Substitute the values: Area = 1/2 * 10 * 5 = 25 square units.
- Step 4: Verify the calculation.
Common Challenges in Geometry Chapter 7
As students work through various problems, there are several common difficulties that arise. These challenges often stem from misunderstanding key concepts, misapplying formulas, or encountering complex problem structures. Recognizing these issues early and knowing how to address them can help prevent frustration and improve overall performance.
By identifying the typical obstacles faced, learners can better prepare themselves to overcome them with confidence. Below are some of the most frequent challenges and strategies to address them.
Frequent Obstacles and Solutions
- Confusing Terminology: Many students struggle with the specific language used in problems, such as terms related to angles, sides, or properties of shapes. Solution: Regularly review definitions and use diagrams to better visualize the concepts.
- Difficulty with Proofs: Proving relationships between elements can be tricky, especially when it involves multiple steps or abstract reasoning. Solution: Break proofs into smaller, logical steps and understand the reasoning behind each step.
- Misapplication of Formulas: Incorrectly applying formulas or confusing similar formulas is a common issue. Solution: Memorize and understand the derivation of key formulas, and always double-check that you are using the correct one for each problem.
- Handling Complex Word Problems: Word problems often require multiple steps and can be overwhelming. Solution: Extract the important information, convert it into mathematical expressions, and solve step-by-step.
- Visualization of Shapes and Diagrams: Some problems require strong spatial reasoning, which can be challenging without a clear visual representation. Solution: Draw diagrams whenever possible, and if a diagram is provided, ensure it is labeled correctly and to scale.
Essential Formulas for Geometry Problems
Understanding and applying the correct formulas is crucial when solving problems involving shapes, sizes, and measurements. These mathematical expressions provide the foundation for finding solutions to various types of questions. Mastery of key formulas not only simplifies the process but also ensures accuracy when calculating areas, volumes, or other measurements.
Below are some of the most commonly used formulas for solving problems in this field. They cover a wide range of concepts, from basic shapes to more complex spatial relationships.
Basic Shape Formulas
- Area of a Triangle: Area = 1/2 * base * height
- Area of a Rectangle: Area = length * width
- Area of a Circle: Area = π * radius²
- Perimeter of a Rectangle: Perimeter = 2 * (length + width)
- Perimeter of a Square: Perimeter = 4 * side
Advanced Shape Formulas
- Volume of a Cube: Volume = side³
- Volume of a Cylinder: Volume = π * radius² * height
- Surface Area of a Sphere: Surface Area = 4 * π * radius²
- Pythagorean Theorem: a² + b² = c² (for right-angled triangles)
- Area of a Trapezoid: Area = 1/2 * (base₁ + base₂) * height
Tips for Mastering Geometry Equations

Mastering mathematical equations is essential for solving problems effectively and efficiently. Whether you are calculating areas, volumes, or finding unknown variables, having a strong grasp of the underlying principles will greatly enhance your ability to work through challenges. These strategies can help you better understand and apply the key formulas used in solving various types of problems.
1. Understand the Concepts Behind the Formulas
It’s not enough to just memorize formulas; understanding why and how each equation works is key. Familiarize yourself with the derivations of important formulas to gain a deeper insight into their application. This will help you recognize patterns and apply the right equation more naturally in different contexts.
2. Break Problems Into Smaller Parts
Large, complex problems can often seem overwhelming. Divide the problem into smaller, manageable steps to make it easier to handle. Start by identifying the known values and then determine what needs to be found. This approach will make equations more straightforward and prevent you from making mistakes along the way.
Additionally, practice regularly with a variety of problems. The more you work with equations, the quicker you’ll become at identifying the right method to solve each one.
Geometry Proofs Explained Simply
Proving geometric relationships is a vital part of understanding and applying theorems. At its core, a proof is a logical sequence of steps that show why a particular statement or relationship is true. While the process might seem complex at first, breaking it down into smaller, logical steps can make it much more manageable.
The key to successfully solving proofs lies in understanding the properties of shapes and the relationships between their parts. By using established rules and logical reasoning, you can demonstrate how and why certain relationships hold true. With practice, this process becomes more intuitive and allows for deeper understanding of the subject.
Steps in a Proof
To make the process of proof clearer, it’s important to follow a methodical approach:
- Step 1: Identify the given information and what needs to be proven.
- Step 2: Draw a diagram if applicable, and label all known elements clearly.
- Step 3: Use definitions, postulates, and theorems to establish a logical argument.
- Step 4: Proceed step-by-step, linking each statement to the previous one.
- Step 5: Conclude by showing that the desired result is true based on the logical progression.
Common Proof Techniques

Several methods can be used to approach proofs, depending on the problem at hand. Two of the most common techniques are:
- Direct Proof: This method involves proving the statement by directly using known facts and logical steps to reach the conclusion.
- Indirect Proof: In this method, you assume the opposite of what you are trying to prove and show that it leads to a contradiction, thereby proving the original statement must be true.
Solving Word Problems in Geometry
Word problems often present a challenge as they require translating real-life scenarios into mathematical expressions. These problems typically involve various figures and measurements, asking you to find missing information based on the relationships between the elements in the scenario. The key to solving these problems lies in carefully extracting relevant data and applying the appropriate formulas to reach a solution.
When approaching word problems, it’s essential to break down the information systematically. Drawing a diagram and identifying known values and what needs to be solved are crucial first steps. Once you have a clear understanding of the problem, you can apply your knowledge of mathematical principles to find the solution.
Steps for Solving Word Problems
- Read the problem carefully: Understand what is given and what needs to be determined.
- Draw a diagram: Visualize the scenario to better understand the relationships between the elements.
- Identify key information: Extract the known values, such as lengths, angles, or areas.
- Choose the appropriate formula: Apply the correct mathematical equation for the situation.
- Solve step-by-step: Work through the problem logically, checking each step as you go.
Example Word Problem
Consider the following word problem:
A rectangle has a length of 10 cm and a width of 5 cm. What is the perimeter of the rectangle?
To solve this, we use the formula for the perimeter of a rectangle: Perimeter = 2 × (length + width).
| Length (cm) | Width (cm) | Perimeter (cm) |
|---|---|---|
| 10 | 5 | 30 |
The perimeter of the rectangle is 30 cm, calculated by substituting the values into the formula: 2 × (10 + 5) = 30.
Using Diagrams to Solve Geometry
Diagrams are powerful tools that help visualize complex relationships in mathematical problems. They provide a clear, structured representation of the elements involved, making it easier to identify key information and understand how different parts of a figure relate to one another. By translating abstract concepts into visual forms, diagrams enable you to approach problems more intuitively and accurately.
When working with geometric problems, a well-drawn diagram can guide you through the solution process. It simplifies the understanding of shapes, angles, and distances, allowing you to apply the correct formulas and make logical deductions. Diagrams are particularly useful in cases where you need to calculate areas, volumes, or other measurements involving multiple elements.
Steps to Create Effective Diagrams
- Label Key Elements: Ensure that all known values, such as lengths, angles, and coordinates, are clearly marked.
- Use Accurate Measurements: Represent all dimensions to scale whenever possible to maintain accuracy.
- Highlight Relationships: Show the connections between different parts of the figure to understand how they influence each other.
- Update as You Solve: As you progress through the problem, adjust the diagram to reflect new information or intermediate results.
Example of a Diagram in Action
Consider a problem where you are asked to find the area of a triangle with a base of 8 cm and a height of 6 cm. Drawing the triangle and labeling the base and height helps you clearly see the dimensions involved. From here, you can apply the area formula for a triangle: Area = 1/2 × base × height.
With the diagram, the problem becomes straightforward, and the solution is simply:
Area = 1/2 × 8 × 6 = 24 cm²
By visually representing the problem, the solution process becomes much clearer and easier to execute.
Practice Problems for Chapter 7
Working through practice problems is one of the most effective ways to solidify your understanding of mathematical concepts. By applying the theories and techniques learned, you can test your knowledge, improve problem-solving skills, and build confidence in your abilities. These exercises are designed to help you practice different types of questions, each focusing on key skills and methods relevant to the topic at hand.
As you tackle these problems, make sure to take your time, draw diagrams when necessary, and refer back to formulas and theorems as needed. The more problems you work through, the better prepared you will be to handle more complex questions in the future. Practice is essential to mastering any subject, and applying it to mathematical reasoning will ensure you fully understand the material.
Example Practice Problems
Try the following practice problems to reinforce your learning:
- Problem 1: Find the area of a circle with a radius of 7 cm.
- Problem 2: Calculate the perimeter of a triangle with sides measuring 5 cm, 6 cm, and 7 cm.
- Problem 3: Determine the volume of a rectangular prism with dimensions 4 cm by 5 cm by 10 cm.
Solutions and Tips
As you work through these problems, remember the following tips:
- For area of a circle: Use the formula Area = π × radius².
- For perimeter of a triangle: Add the lengths of all three sides together.
- For volume of a rectangular prism: Use the formula Volume = length × width × height.
Once you have solved these problems, check your answers and revisit any steps you found challenging. This will help reinforce your understanding and improve your problem-solving techniques for future challenges.
How to Check Your Geometry Answers
Ensuring the accuracy of your solutions is a critical step in any mathematical task. After working through a problem, it’s essential to verify your results to confirm that you’ve applied the correct methods and formulas. Double-checking your calculations not only helps identify any potential mistakes but also solidifies your understanding of the concepts involved. By developing a systematic approach to reviewing your work, you can ensure that your answers are both reliable and precise.
There are several strategies to check your work effectively. One common method is to rework the problem from the beginning, paying attention to each step to spot any inconsistencies. Another technique involves using different approaches to solve the same problem and comparing the results. Additionally, when dealing with geometric problems, reviewing the diagram and checking measurements can often reveal discrepancies that might have been overlooked during the initial solving process.
Steps to Verify Your Solutions
- Revisit the problem: Carefully read through the problem statement again to ensure that you understand the question correctly.
- Double-check calculations: Review your mathematical operations, including adding, subtracting, multiplying, or dividing, to make sure each step is accurate.
- Cross-check with different methods: If possible, try solving the problem using a different formula or approach to confirm your result.
- Review your diagram: Ensure that any drawings or figures are accurate and correspond to the dimensions and conditions given in the problem.
Example: Checking a Triangle’s Area
Let’s say you’re asked to find the area of a triangle with a base of 6 cm and a height of 8 cm. The formula for the area of a triangle is:
| Formula | Calculation |
|---|---|
| Area = 1/2 × base × height | Area = 1/2 × 6 × 8 = 24 cm² |
Once you’ve completed the calculation, it’s a good idea to recheck the values you’ve used. Ensure that the base and height are correctly labeled in your diagram, and verify that you’ve applied the correct formula. By following these steps, you can be confident that your solution is accurate.
Important Theorems in Geometry Chapter 7
Understanding key theorems is essential for solving various problems in mathematics. These fundamental principles provide the foundation for making logical deductions and solving complex problems. Theorems serve as tools that help in proving relationships between different elements and structures, making them indispensable for anyone looking to deepen their understanding of mathematical reasoning. In this section, we will explore some of the most important theorems and how they apply to solving common problems.
By mastering these theorems, you will be able to approach problems with confidence, applying proven methods to reach correct solutions. Whether you’re working with angles, shapes, or measurements, understanding the underlying principles can simplify the process and help you avoid common mistakes. Theorems can often be used in combination, providing a powerful toolkit for tackling a wide range of mathematical challenges.
Geometry Terminology You Should Know
Having a strong grasp of key terms is crucial when tackling any mathematical problems related to shapes and their properties. Understanding the language of mathematics allows you to interpret questions correctly and apply the right concepts to solve problems effectively. Whether you’re analyzing angles, lengths, or specific geometric figures, the correct terminology helps you communicate your reasoning and understand the principles behind each solution.
In this section, we’ll cover some of the essential terms that will help you navigate complex problems with ease. Familiarizing yourself with these terms is the first step in building a solid foundation for understanding more advanced topics in the future.
Key Terms in Mathematical Reasoning
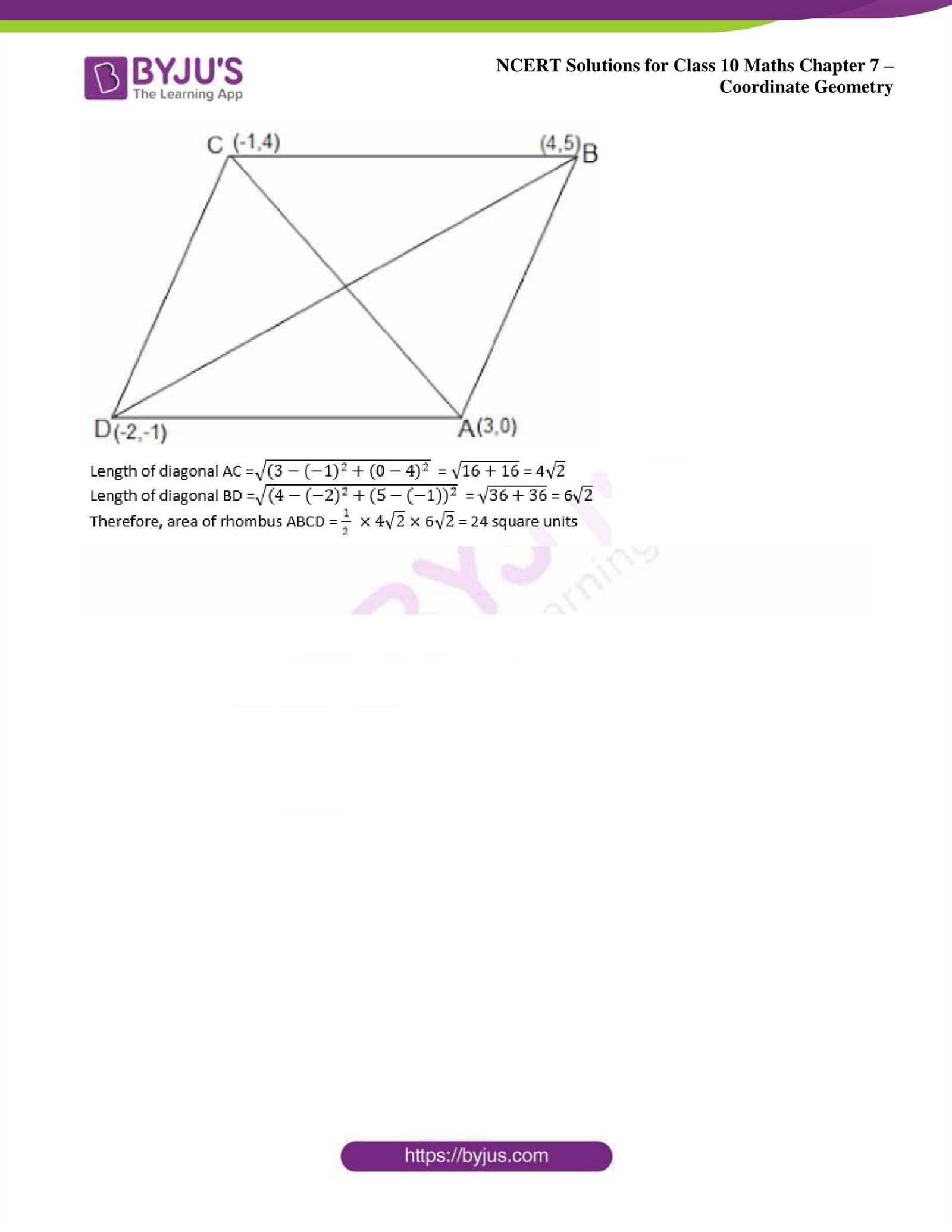
- Angle: The figure formed by two rays sharing a common endpoint, measured in degrees.
- Parallel Lines: Two lines in the same plane that never intersect, no matter how far they are extended.
- Perpendicular Lines: Two lines that intersect at a right angle (90 degrees).
- Congruent: When two figures have the same shape and size, they are congruent.
- Symmetry: A property where one part of a shape is a mirror image of another part.
Terms Related to Shapes and Figures
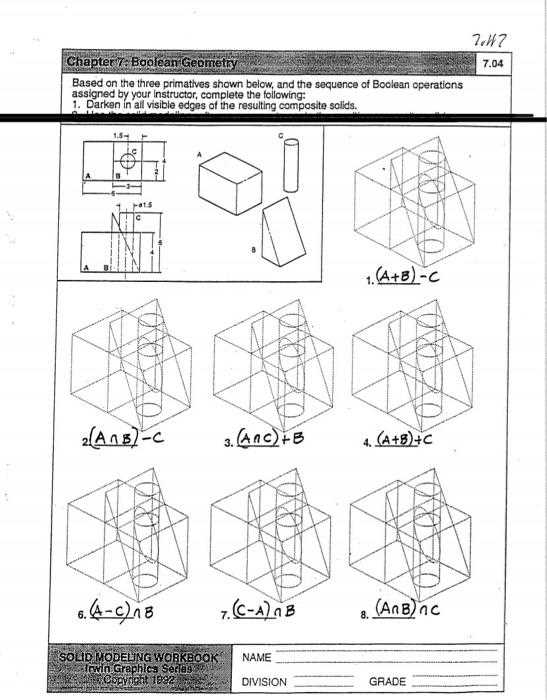
- Circle: A round shape where all points are equidistant from the center.
- Triangle: A three-sided polygon with three angles.
- Polygon: A closed shape with straight sides, such as squares, rectangles, or pentagons.
- Radius: The distance from the center of a circle to any point on its circumference.
- Area: The amount of space inside a shape, typically measured in square units.
Mastering these terms will provide you with the vocabulary needed to approach and solve problems with clarity and precision.
Real-World Applications of Geometry
Mathematics is not just a theoretical subject; it has practical applications that affect many aspects of our daily lives. The study of shapes, sizes, and the relationships between various objects is essential in various industries and fields. From architecture to engineering, the principles of spatial understanding play a critical role in problem-solving and design. By applying these concepts to real-world scenarios, we can solve everyday challenges with efficiency and accuracy.
In this section, we will explore some of the ways that concepts related to shapes and spatial reasoning are applied in real-world situations. Whether you’re designing a building, planning a road network, or simply calculating areas for a garden, these mathematical ideas are everywhere, helping to bring ideas into the physical world.
Applications in Architecture and Design
- Building Structures: Architects rely on principles of measurement, symmetry, and proportion to design safe, aesthetically pleasing buildings.
- Interior Design: Layouts, furniture placement, and spatial relationships are all designed using geometric concepts to optimize space and functionality.
- Urban Planning: When designing cities or towns, planners use geometry to determine road networks, public spaces, and the placement of utilities.
Applications in Technology and Engineering
- Engineering Projects: Engineers use geometry to design mechanical systems, bridges, and other structures that require precise measurements and balance.
- Computer Graphics: In digital imaging and 3D modeling, geometric algorithms are used to create realistic visual effects and simulations.
- Robotics: Geometric principles help engineers design robots with precise movements and interactions with the environment.
These examples show how vital the understanding of shapes and measurements is to creating the world around us. From the buildings we live in to the technology we use, these concepts provide the foundation for innovation and development in various industries.
Common Mistakes to Avoid in Geometry
When working with spatial problems, it’s easy to make mistakes that can throw off the entire solution process. Whether you’re calculating areas, working with angles, or dealing with shapes, a small misstep can lead to significant errors. Recognizing these common mistakes and understanding how to avoid them is key to mastering this subject. Below are some of the most frequent pitfalls and how to navigate around them to ensure accurate results.
Misunderstanding Key Concepts
- Confusing Types of Angles: Not distinguishing between acute, obtuse, and right angles can lead to incorrect calculations or assumptions.
- Forgetting Theorems: Skipping important geometric rules, such as the Pythagorean Theorem, can cause errors in solving problems involving triangles or distances.
- Misapplying Formulas: Using the wrong formula for a shape (e.g., using the area formula for a rectangle on a triangle) is a common mistake.
Calculation Errors
- Wrong Arithmetic: Simple addition or multiplication mistakes can add up and distort final answers.
- Incorrect Units: Forgetting to convert between different units of measurement (such as inches to centimeters) can lead to confusion and incorrect results.
- Neglecting Units in Final Answer: Omitting the units of measurement in the final result can make the answer incomplete and potentially confusing.
By being mindful of these common mistakes, students can greatly improve their accuracy and confidence in solving geometric problems. Understanding the right concepts, applying correct formulas, and double-checking calculations will ensure a smoother experience and more reliable outcomes.
Study Tips for Geometry Success
Achieving success in spatial reasoning requires more than just understanding formulas and theorems. It involves developing strong problem-solving skills, practicing consistently, and mastering core concepts. Whether you’re preparing for an exam or working on homework, using effective study strategies can significantly improve your performance. Below are some practical tips to help you excel in this subject.
- Practice Regularly: Consistent practice is crucial. Work through a variety of problems to strengthen your understanding and build confidence in solving different types of questions.
- Understand Key Concepts: Focus on mastering the foundational principles, such as shapes, angles, and measurement techniques. This knowledge will serve as the base for tackling more complex problems.
- Use Visual Aids: Drawing diagrams and sketching out problems can help you better understand the relationships between different components. Visualizing a problem often makes it easier to find a solution.
- Break Problems Into Steps: Avoid jumping straight into the solution. Break each problem down into smaller steps to make it more manageable and ensure that no detail is overlooked.
- Review Mistakes: Don’t just focus on the problems you get right. Review incorrect answers to understand where you went wrong and how you can avoid similar mistakes in the future.
By incorporating these tips into your study routine, you can improve both your understanding and your performance. The key is to stay consistent, focused, and willing to put in the effort to practice regularly and review your work.