In the study of mathematical principles, practice plays a crucial role in strengthening understanding and problem-solving abilities. By working through various exercises, learners can refine their skills and develop a deeper comprehension of core concepts. Whether you’re revisiting familiar ideas or tackling new challenges, consistent practice is key to mastering the material.
Engaging with problems that require analytical thinking and application of learned formulas helps build confidence and competence. By focusing on key topics, students can improve their ability to approach and solve complex questions with ease. This process not only enhances theoretical knowledge but also prepares individuals for practical situations where these concepts are applicable.
Through structured practice, individuals can identify patterns, make connections, and sharpen their critical thinking. As problems become progressively more challenging, a deeper insight into the underlying principles emerges, leading to greater academic success and personal growth in mathematics.
Key Concepts in Mathematics for Problem Solving
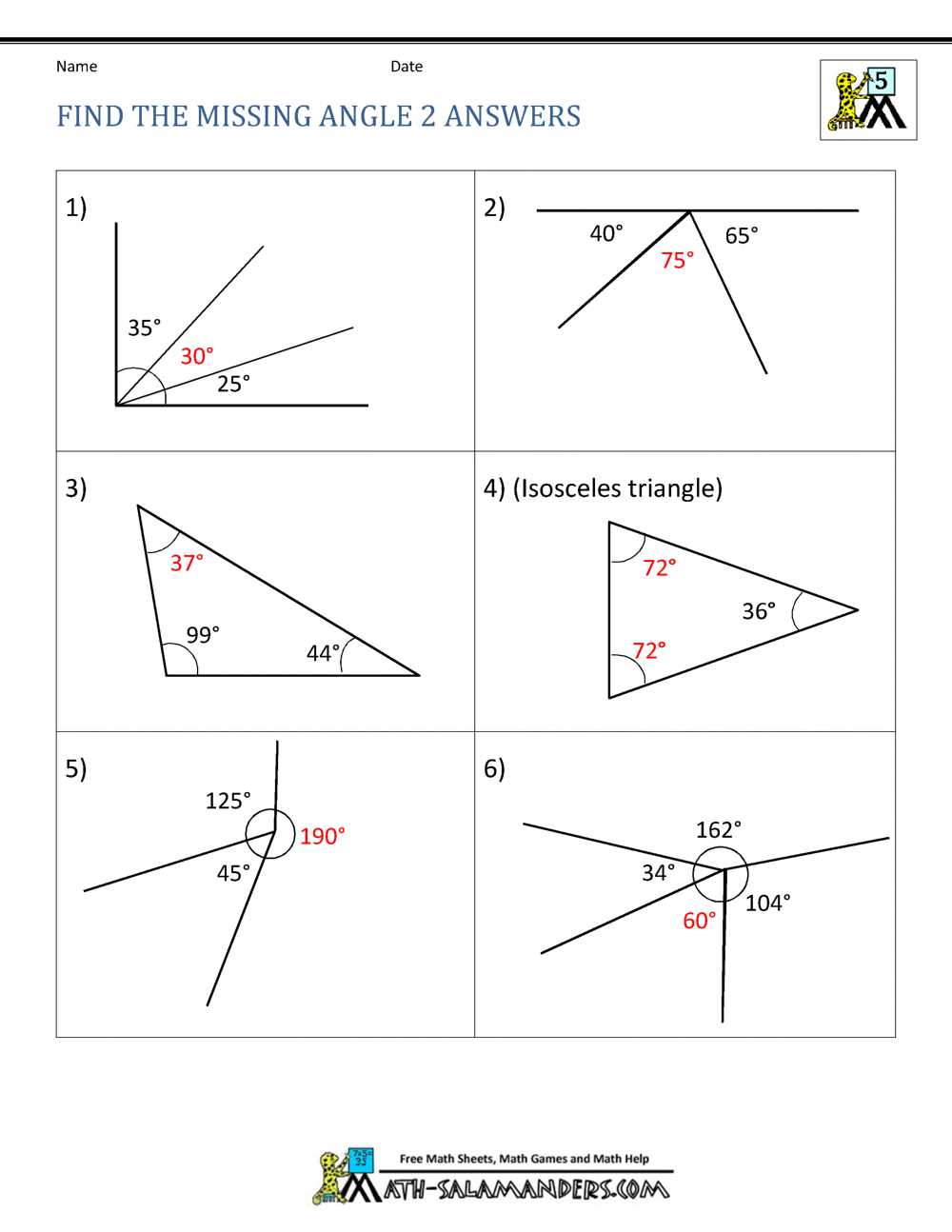
Understanding the core principles behind geometric shapes and spatial relationships is essential for solving various problems. Key ideas revolve around analyzing figures, recognizing patterns, and applying specific rules to determine unknown values. A solid grasp of these concepts forms the foundation for advancing in more complex mathematical tasks and calculations.
Essential Principles of Shapes and Their Properties
One of the fundamental aspects of mathematical studies is recognizing the properties of different shapes. Each type of figure has its own set of characteristics, such as angles, sides, and symmetry. These properties can be used to derive relationships between various elements within the figure, allowing for more efficient problem-solving. Understanding these relationships is crucial when determining unknown values or solving for specific conditions.
Applying Formulas and Theorems
Formulas and theorems provide structured methods for solving problems involving measurements and calculations. For example, knowing the right formula for the area of a triangle or the perimeter of a square allows one to quickly calculate key values. Mastering these tools enhances the ability to tackle a wide range of exercises efficiently, while also building a deeper understanding of mathematical structures and reasoning.
Understanding Mathematical Practice Problems
Mathematical exercises are designed to reinforce key concepts and develop problem-solving skills. These tasks require learners to apply theoretical knowledge to practical situations, strengthening their understanding through repetition and exploration. By systematically working through these challenges, students improve their ability to analyze and solve problems with accuracy.
Breaking Down Complex Problems
Each problem is structured to build upon previous knowledge, encouraging the development of critical thinking and analytical skills. Problems can vary in complexity, requiring different approaches and methods to solve. Breaking down these exercises into smaller, manageable steps is an effective way to tackle even the most difficult tasks. Understanding the logical flow behind each question helps to avoid confusion and ensures successful outcomes.
Common Problem Types and Techniques
Some problems focus on specific mathematical principles such as measurements, angles, and proportions, while others test the ability to apply formulas in various contexts. Recognizing the type of problem at hand is the first step towards selecting the correct solution method. The following table illustrates some common problem types and the strategies typically used to solve them:
| Problem Type | Strategy |
|---|---|
| Area and Perimeter | Use specific formulas based on shape properties |
| Angle Relationships | Apply angle theorems and complementary rules |
| Symmetry and Proportions | Identify symmetrical properties and use proportional reasoning |
| Volume Calculations | Use volume formulas for 3D shapes |
By familiarizing oneself with the types of problems and the corresponding techniques, learners can approach each exercise with confidence and clarity, enhancing their overall mathematical proficiency.
Step-by-Step Solutions for Practice
Working through problems systematically is key to building a strong foundation in mathematics. By breaking down each problem into smaller, manageable steps, students can better understand the logic behind each solution and avoid making errors. This approach encourages critical thinking and improves problem-solving efficiency over time.
Each exercise typically involves several stages, starting with analyzing the given information, followed by selecting the appropriate method, and finally solving for the unknowns. As the complexity of the tasks increases, the steps become more detailed, requiring a deeper understanding of the underlying principles. Practicing this method repeatedly helps solidify concepts and fosters greater confidence in tackling new challenges.
By taking a methodical approach, learners can track their progress, identify areas for improvement, and continuously refine their skills. Regularly practicing this step-by-step process ensures that each concept is thoroughly understood and applied accurately in various scenarios.
Common Mistakes in Mathematical Problem Solving
When solving mathematical problems, it’s easy to make mistakes, especially when concepts are complex or tasks require multiple steps. Recognizing and understanding common errors can help students avoid them and improve their accuracy. Many mistakes arise from misunderstandings of key principles or rushing through calculations without double-checking the work.
Frequent Errors to Watch Out For
- Misapplying formulas: Using the wrong formula for a specific type of problem or misinterpreting how to apply it is a common mistake.
- Forgetting units: Ignoring or incorrectly converting units can lead to incorrect results, especially in problems involving measurements.
- Not simplifying expressions: Sometimes, students skip simplifying intermediate steps, which can lead to confusion or incorrect final answers.
- Incorrectly labeling parts of a figure: Mistakes can occur when angles, sides, or points are misidentified, leading to errors in calculations.
- Overlooking details in the problem: Failing to account for all given information, such as constraints or relationships between elements, can cause students to miss important parts of the solution.
Tips for Avoiding Mistakes
- Always double-check the formulas you’re using and make sure they match the problem’s requirements.
- Carefully convert and include units in every step to avoid mistakes in measurements.
- Take your time to simplify expressions and ensure each step is logical and clear.
- Review the problem and its diagram thoroughly before starting calculations, and ensure you correctly identify key elements.
- Reread the problem after solving to ensure all given information has been used correctly.
By being mindful of these common mistakes, students can develop stronger problem-solving skills and improve their overall performance in mathematical tasks.
Tips for Solving Mathematical Problems
Effective problem-solving requires a combination of strategy, attention to detail, and practice. Whether tackling a straightforward question or a more complex challenge, following a structured approach can make the process easier and more efficient. By focusing on key principles and avoiding common mistakes, learners can improve their problem-solving skills and enhance their understanding of the subject.
Breaking Down the Problem
Before jumping into calculations, it’s important to carefully analyze the problem. Identify the known and unknown values, as well as any relationships between different elements. Drawing a diagram or visualizing the problem can help clarify the situation and make it easier to spot key information. A clear understanding of the problem will guide you in selecting the most appropriate method for solving it.
Organizing Your Work
Organizing each step of the solution is essential to avoid errors and ensure clarity. Write down the steps in a logical order, showing each calculation or decision as you go. This process will help you track your progress and make it easier to identify mistakes if they occur. Additionally, reviewing your work step-by-step allows you to catch any missing elements or miscalculations before finalizing the solution.
How to Approach Problem-Solving Tasks
When faced with a set of mathematical exercises, it’s essential to approach each question methodically. By taking the time to understand the problem fully and organizing your thoughts, you can solve challenges more effectively and with greater accuracy. Following a clear strategy from start to finish helps prevent confusion and ensures that no steps are overlooked.
Read the Problem Carefully
The first step in solving any problem is to read it thoroughly. Pay close attention to the given information and any instructions provided. Identifying the key details and understanding what is being asked allows you to determine the most effective way to tackle the question. Skipping this initial step can lead to mistakes or misunderstandings later on.
Choose the Right Method
Once you understand the problem, decide which method or formula is best suited to solve it. In some cases, the approach may be straightforward, but in others, you may need to draw diagrams, break the problem into smaller parts, or apply specific rules. Carefully consider all options before proceeding, as choosing the right method is crucial to obtaining the correct result.
Exploring Problem Types in Mathematical Exercises
Mathematical problems can vary widely in structure and complexity, each requiring different approaches and strategies. Understanding the different types of problems is essential for tackling them effectively. By recognizing the unique characteristics of each problem, learners can quickly identify the best methods for solving them, improving their efficiency and accuracy.
Problems may focus on different concepts, such as spatial relationships, measurements, angles, or calculations involving formulas. Some tasks might be more theoretical, requiring logical reasoning, while others may involve applying specific formulas or strategies to determine unknown values. Being familiar with the variety of problem types allows you to approach each challenge with confidence and clarity.
Visualizing Mathematical Concepts for Better Understanding
One of the most effective ways to grasp complex concepts is through visualization. By creating mental images or drawing diagrams, learners can see the relationships between different elements, which helps make abstract ideas more tangible. Visualizing problems not only aids in comprehension but also improves problem-solving skills by providing a clear structure to work with.
For many tasks, drawing a diagram or sketching a figure can simplify the process of solving the problem. Whether it’s a shape, a series of angles, or a measurement, a visual representation can highlight important details and guide the solution method. Using visuals helps to break down complicated ideas into more manageable parts, making them easier to understand and solve.
Benefits of Visualization
- Clarifies complex relationships between shapes and elements.
- Helps in identifying key information that may not be immediately obvious.
- Facilitates a deeper understanding of abstract concepts by providing a concrete reference.
- Improves accuracy by reducing errors in interpretation or calculation.
Practical Tips for Effective Visualization
- Always sketch the problem if possible, even if it seems simple.
- Label key parts of the diagram (angles, sides, points) to avoid confusion.
- Use color or shading to highlight important elements for better clarity.
- Work through the problem step by step, adjusting the diagram as needed.
By making visualization a regular part of your problem-solving approach, you can improve both your understanding and your ability to tackle a wide range of mathematical challenges with confidence.
Using Formulas to Solve Mathematical Problems
Formulas are essential tools in mathematics that provide a systematic approach to solving various types of problems. By using the right formula, you can simplify complex tasks and find solutions quickly and accurately. Understanding how and when to apply these formulas is key to mastering problem-solving techniques, whether you’re calculating areas, volumes, or other related measurements.
Each formula serves a specific purpose, depending on the type of problem you’re facing. Recognizing the appropriate formula for a given situation allows you to break down the task into manageable steps. Once you apply the formula correctly, solving the problem becomes a straightforward process of plugging in the known values and performing the necessary calculations.
Mastering the use of formulas also means understanding their derivation and how they relate to the concepts behind them. This deeper understanding helps reinforce your knowledge and improves your ability to adapt the formula to different scenarios. As you gain more experience, you will become more confident in recognizing which formulas to use and how to manipulate them for different types of problems.
Interactive Methods for Learning Mathematical Concepts
Interactive learning methods offer an engaging way to understand complex concepts by actively involving students in the learning process. Instead of passively absorbing information, learners can explore, experiment, and manipulate elements of the subject, which helps reinforce understanding and build stronger problem-solving skills. These hands-on approaches allow learners to connect theory with practice, making abstract ideas more accessible.
One effective method is through the use of digital tools that enable students to visualize concepts in real time. Interactive simulations and online platforms allow learners to experiment with shapes, angles, and other elements, providing immediate feedback that helps them identify mistakes and correct their understanding. Additionally, interactive exercises encourage active participation, which can significantly enhance retention of material.
Collaboration and Peer Learning is another interactive technique that fosters deeper understanding. Working together with classmates to solve problems not only promotes critical thinking but also encourages the sharing of ideas and strategies. Group discussions allow for the exploration of different methods, leading to a broader perspective and stronger comprehension.
Finally, real-world applications can make abstract concepts more relevant. By applying mathematical principles to real-life situations, students gain a deeper appreciation for the subject and can see how their knowledge is used outside of the classroom. Interactive methods bring these principles to life, making learning both enjoyable and effective.
Breaking Down Complex Problems
When faced with a challenging problem, it’s important to break it down into smaller, more manageable parts. Instead of trying to solve everything at once, focus on isolating individual components and addressing them step by step. This approach not only simplifies the problem but also ensures that nothing is overlooked and that the solution process remains clear and logical.
One effective way to break down complex tasks is to identify key elements such as known values, unknowns, and the relationships between different parts of the problem. By focusing on these elements, you can develop a structured plan for solving each aspect of the problem systematically.
Step-by-Step Problem Breakdown
| Step | Action | Explanation |
|---|---|---|
| 1 | Identify key information | List known values and relevant relationships within the problem. |
| 2 | Draw a diagram | Visualize the problem to better understand the spatial relationships. |
| 3 | Choose a method | Determine the most appropriate approach or formula for solving. |
| 4 | Solve step by step | Break the solution into smaller tasks to avoid mistakes. |
| 5 | Verify the solution | Check the final result to ensure it aligns with the problem’s conditions. |
By following this systematic approach, solving even the most complex problems becomes more structured and less overwhelming. It helps to clarify the steps and make the process more efficient, reducing the risk of errors and increasing confidence in the solution.
Benefits of Solving Mathematical Practice Problems
Engaging with practice exercises is an effective way to reinforce knowledge and improve problem-solving skills. These exercises provide an opportunity to apply theoretical concepts in a practical setting, making abstract ideas more tangible and easier to understand. By working through a variety of problems, learners can sharpen their reasoning abilities, gain confidence, and develop a deeper understanding of mathematical principles.
Here are some key benefits of regularly solving these types of problems:
- Improved Problem-Solving Skills: Practice helps to develop critical thinking and analytical skills, allowing learners to approach complex problems with confidence.
- Better Retention: Repetition strengthens memory, making it easier to recall formulas, theorems, and methods when needed.
- Increased Speed: As learners become more familiar with different types of problems, they can solve them more quickly and efficiently.
- Deeper Understanding: Applying concepts to real problems enhances comprehension and shows how different ideas are connected.
- Confidence Boost: Successfully solving problems increases self-assurance and motivates learners to tackle even more challenging questions.
Through consistent practice, students can turn theoretical knowledge into practical expertise, making it easier to master the material and succeed in future tasks. Whether it’s for classwork, exams, or personal development, solving these problems provides lasting benefits that extend far beyond the classroom.
Reviewing Key Points
When studying mathematical concepts, it’s essential to regularly revisit and review the most important ideas and techniques. This helps to reinforce understanding, fill in any gaps, and ensure that the concepts are applied correctly. By summarizing the core principles and steps involved, learners can strengthen their grasp on the material and prepare for more complex challenges.
Below are some of the key points to remember when working through mathematical exercises:
- Identifying Key Elements: Recognize important values such as variables, constants, and relationships that are crucial to solving problems.
- Understanding Relationships: Focus on how different aspects of a problem interact with each other and how they contribute to the overall solution.
- Using Formulas Correctly: Ensure that the right formulas are applied for each situation, and double-check the substitutions to avoid mistakes.
- Visualizing Problems: Diagrams and charts can significantly help in understanding the problem and simplifying the process of finding a solution.
- Practicing Regularly: Frequent practice with varied problems ensures better retention of concepts and greater ease when encountering new challenges.
By reviewing these critical concepts regularly, learners can not only enhance their problem-solving skills but also build the foundation for future mathematical success. Mastery of these points is essential for tackling progressively harder problems with greater ease and confidence.
Learning Strategies for Success
Achieving mastery in mathematical subjects requires effective study strategies that promote deeper understanding and efficient problem-solving. Successful learners not only focus on memorizing formulas but also engage with the material in ways that allow them to connect concepts and build a solid foundation. These strategies, when applied consistently, help students navigate complex topics with confidence and precision.
Here are some key learning strategies to help you succeed:
| Strategy | Description |
|---|---|
| Active Practice | Consistently working through problems solidifies understanding and enhances recall during exams. |
| Concept Mapping | Create visual diagrams to connect related ideas, helping to understand how different principles fit together. |
| Seek Clarification | Whenever a concept is unclear, ask for help from peers, teachers, or online resources to ensure comprehension. |
| Chunking Information | Break complex topics into smaller, manageable sections to avoid feeling overwhelmed and increase retention. |
| Test Yourself | Self-testing with practice questions boosts confidence and reveals areas where further study is needed. |
Incorporating these strategies into your study routine can significantly improve your learning efficiency and ability to solve complex problems. Remember, the key to success lies in not only working hard but also working smart, applying methods that best suit your learning style and goals.
Improving Problem-Solving Skills
Developing strong problem-solving skills is essential for mastering any challenging mathematical subject. By adopting effective techniques and approaches, students can enhance their ability to tackle complex questions with greater efficiency. It’s not just about finding the correct answer, but understanding the steps involved and learning how to approach problems from different angles.
Understanding the Problem
The first step in solving any problem is fully understanding what is being asked. Read the problem carefully and identify the key information. Break down the question into smaller, more manageable parts, and highlight the important details that will guide your solution. Clarifying the goal and recognizing what is given will help you approach the problem methodically.
Breaking Down the Solution
Once you understand the problem, break it into smaller steps. Don’t attempt to solve everything at once. Work through the logical progression of steps, one at a time, ensuring that each step follows from the previous one. By building the solution gradually, you reduce the risk of making errors and ensure a more thorough understanding of the process.
Furthermore, practice is a key element in developing problem-solving proficiency. The more problems you solve, the more strategies and techniques you will acquire. Eventually, your intuition for recognizing patterns and solutions will improve, allowing you to solve more challenging problems with ease.
Mathematical Concepts and Real-Life Applications
The study of mathematical principles goes beyond classroom exercises and problem-solving drills. In fact, the knowledge gained from mastering various mathematical techniques has numerous practical applications in real-world scenarios. From designing buildings to navigating complex technology, the ability to apply mathematical thinking is essential in many fields.
One area where these concepts are crucial is in architecture and construction. Understanding shapes, angles, and measurements allows engineers and architects to create safe and functional structures. Similarly, these skills are vital in other fields such as:
- Art and Design: Artists use principles of symmetry and proportion to create visually appealing compositions.
- Technology: Software developers and engineers rely on these concepts for creating algorithms, digital graphics, and user interfaces.
- Navigation: Pilots, sailors, and drivers utilize distance and angle calculations to determine routes and positions accurately.
- Sports: Coaches and athletes apply these principles to improve performance, whether it’s optimizing the angle of a throw or designing efficient sports equipment.
Moreover, the practice of solving mathematical problems helps individuals improve their critical thinking, decision-making, and analytical skills, all of which are invaluable in everyday life. By recognizing how these principles are used in the world around us, students can better appreciate the importance of their studies and how they contribute to various industries and professions.
How to Check Your Solutions
When completing any set of problems, it’s crucial to verify your work to ensure accuracy. Double-checking helps identify potential mistakes and reinforces your understanding of the material. Whether you’re solving mathematical equations or applying concepts to real-life situations, a systematic approach to reviewing your solutions can greatly improve your confidence and results.
Step 1: Review Your Work Methodically
Start by revisiting each step of your solution process. Ensure that every calculation was performed correctly and that each step logically follows from the previous one. Mistakes often arise from small oversights, so it’s important to check even the simplest calculations. Additionally, be sure that the problem was understood correctly before proceeding with the solution.
Step 2: Use Alternative Methods to Confirm Results
Whenever possible, try using a different approach to solve the same problem. For example, if you used algebraic manipulation to solve an equation, you could check your result with substitution or graphing. This provides a second layer of verification and helps catch any discrepancies. Using multiple methods to arrive at the same conclusion is a great way to confirm your results.
In cases where you’re solving problems involving formulas, consider plugging in values into the formula again to ensure consistency with your earlier work. If you’re dealing with geometric problems, drawing diagrams or visualizing the situation can also help confirm your answers.
By thoroughly checking your work and using multiple verification techniques, you increase the accuracy of your solutions and deepen your understanding of the material. This approach can make a significant difference in your performance and mastery of the subject.