In this section, we will dive into essential topics that form the foundation of advanced studies in shapes, figures, and their properties. A clear understanding of these principles is crucial for tackling complex mathematical problems and achieving a deeper comprehension of spatial relationships. Whether you’re solving equations or exploring figures, grasping these core ideas will enhance your problem-solving skills.
By examining a variety of exercises and strategies, this guide will help you navigate through the critical concepts with clarity. Expect to encounter different approaches to calculating distances, angles, and areas while refining your logical reasoning. With the right methods, you’ll be well-equipped to handle any challenge that comes your way in this area of study.
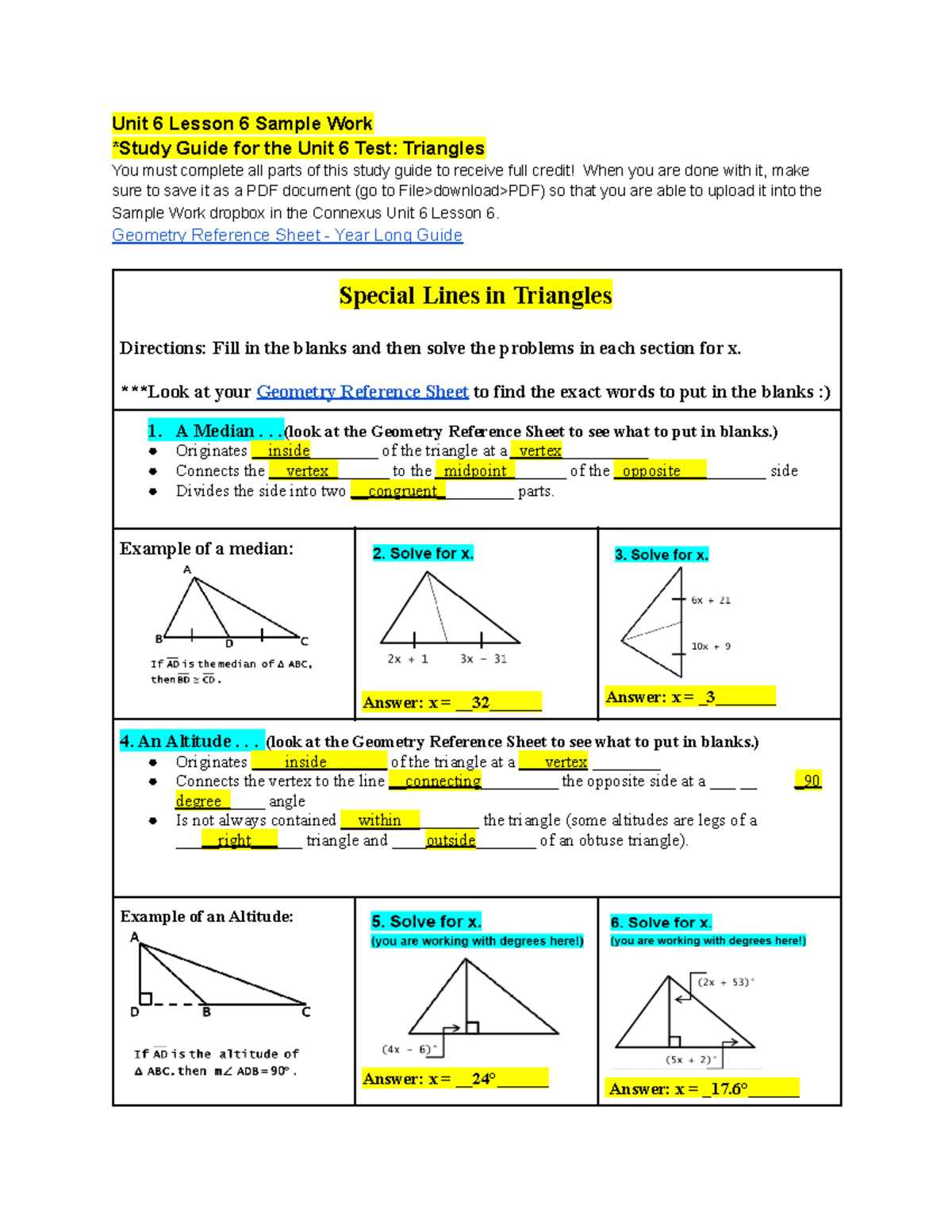
Geometry Chapter 6 Review Answers
In this section, we focus on refining your understanding of essential concepts and skills that are crucial for solving complex mathematical problems involving shapes and their properties. The exercises covered here aim to strengthen your ability to analyze and solve different types of geometric challenges. With a solid grasp of these key ideas, you’ll be prepared to tackle more advanced topics in the subject.
Key Problem-Solving Strategies
Mastering the right techniques is essential for approaching various types of problems. By practicing step-by-step solutions, you can sharpen your skills and improve accuracy in calculations. Focus on understanding the underlying principles rather than memorizing formulas, as this will allow for greater flexibility in problem-solving.
Common Missteps to Avoid
While working through these problems, it’s important to stay aware of common mistakes that can hinder progress. One frequent error is misinterpreting the relationships between different elements, such as angles and sides. Paying close attention to the details will help you avoid these pitfalls and ensure more reliable results in your calculations.
Key Concepts in Geometry Chapter 6
Understanding fundamental principles is crucial for mastering the subject. This section emphasizes the core ideas and methods needed to work with shapes, lines, and angles. Developing a clear comprehension of these topics allows for a better grasp of more complex problems in this field.
Essential Properties of Shapes

One of the key concepts in this area is the understanding of different types of figures and their properties. Whether dealing with triangles, quadrilaterals, or circles, recognizing the relationships between sides, angles, and areas is vital. These concepts are the building blocks for more intricate calculations and proofs.
Fundamentals of Proofs and Theorems
Another critical element involves the application of theorems and the construction of logical proofs. These are tools used to demonstrate the validity of certain properties or relationships. Mastery of proofs strengthens problem-solving abilities and helps in deriving correct conclusions based on established principles.
Understanding the Main Theorems

Theorems serve as the foundation for many mathematical principles. By studying and applying these key propositions, you can unlock the relationships between various elements within figures. Understanding how to prove and utilize theorems is essential for solving complex problems and drawing accurate conclusions in this field.
Each theorem provides a logical explanation of how different parts of a figure interact with one another. Whether it’s about the sum of angles in a polygon or the relationship between parallel lines and transversals, these rules guide the way we approach and resolve challenges. Mastery of theorems will greatly improve your ability to navigate through advanced topics and tackle a wide variety of problems.
Step-by-Step Review of Problems
Breaking down problems into smaller, manageable steps is a key approach to mastering this field. By following a structured process, you can better understand how to approach different situations and ensure accuracy in solving. This methodical approach allows you to focus on each part of the problem without becoming overwhelmed by the complexity of the task at hand.
Common Problem-Solving Strategy
Start by identifying the given information and what is being asked. Then, choose the best approach based on the type of problem. A typical process involves:
- Reading the problem carefully to highlight key details.
- Drawing a diagram or figure when necessary for better visualization.
- Applying the relevant formulas or theorems.
- Checking calculations and reviewing the logic to ensure correctness.
Key Examples to Practice
Now, let’s explore a few examples of problems and the step-by-step process used to solve them:
- Example 1: Solving for unknown angles in polygons.
- Example 2: Finding the area or perimeter of complex shapes.
- Example 3: Using the Pythagorean theorem to solve for missing sides.
By practicing this process with a variety of problems, you will strengthen your problem-solving skills and gain confidence in tackling more advanced exercises.
How to Approach Geometry Proofs
Proofs are a fundamental part of mathematical reasoning, requiring a logical sequence of steps to demonstrate the truth of a statement. When approaching a proof, it’s essential to focus on the relationships between different parts of a figure, applying known rules and theorems to logically justify each step. A clear, methodical approach will help you structure your work and avoid unnecessary confusion.
Step-by-Step Process for Proofs
Here’s a structured method to follow when working on a proof:
- Understand the Given Information: Carefully read the problem to identify the known facts and what is being asked to prove.
- Visualize the Problem: Draw diagrams or figures to better understand the situation. Label all known information and mark unknowns.
- Choose a Strategy: Determine which theorems, properties, or rules will help establish the relationships you need to prove.
- Break Down the Steps: Write each step logically, ensuring each follows from the previous one, using valid reasoning.
- Conclude with a Clear Statement: Finish by clearly stating the result that has been proven, ensuring that all steps are justified.
Common Proof Techniques
There are several methods commonly used to solve proofs. Familiarizing yourself with these techniques will make solving problems easier:
- Direct Proof: Start from known facts and build toward the conclusion in a straight path.
- Proof by Contradiction: Assume the opposite of what you want to prove and show that this assumption leads to a contradiction.
- Proof by Induction: Use a base case to prove a statement for all natural numbers by showing it holds for one case and then for the next.
By practicing these methods and following a clear structure, you will improve your ability to tackle and solve proofs efficiently.
Common Mistakes in Geometry Exercises
When working through mathematical problems, it’s easy to make errors that can affect the accuracy of your results. These mistakes often arise from misunderstandings of key concepts or overlooking important details. By recognizing these common pitfalls, you can take steps to avoid them and improve your problem-solving approach.
One frequent mistake is misinterpreting the relationships between different elements of a figure. For example, assuming certain angles or sides are congruent when they are not can lead to incorrect solutions. Another common issue is forgetting to apply relevant theorems or properties, which are crucial for solving many types of problems.
To minimize mistakes, it’s important to carefully read the problem, double-check calculations, and ensure that each step logically follows from the previous one. Taking the time to review and verify your work can help catch errors before they become major obstacles.
Mastering Geometric Transformations
Understanding how to manipulate figures through various transformations is an essential skill. These operations allow you to change the position, size, and orientation of shapes while preserving their fundamental properties. Mastering these transformations is key to solving a wide range of mathematical problems and creating accurate models.
Types of Transformations
There are several types of transformations, each with its unique characteristics. The most common include:
- Translation: Moving a figure without changing its shape or orientation.
- Rotation: Turning a figure around a fixed point by a certain angle.
- Reflection: Flipping a figure over a line to create a mirror image.
- Scaling: Changing the size of a figure while maintaining its proportions.
Applying Transformations to Solve Problems
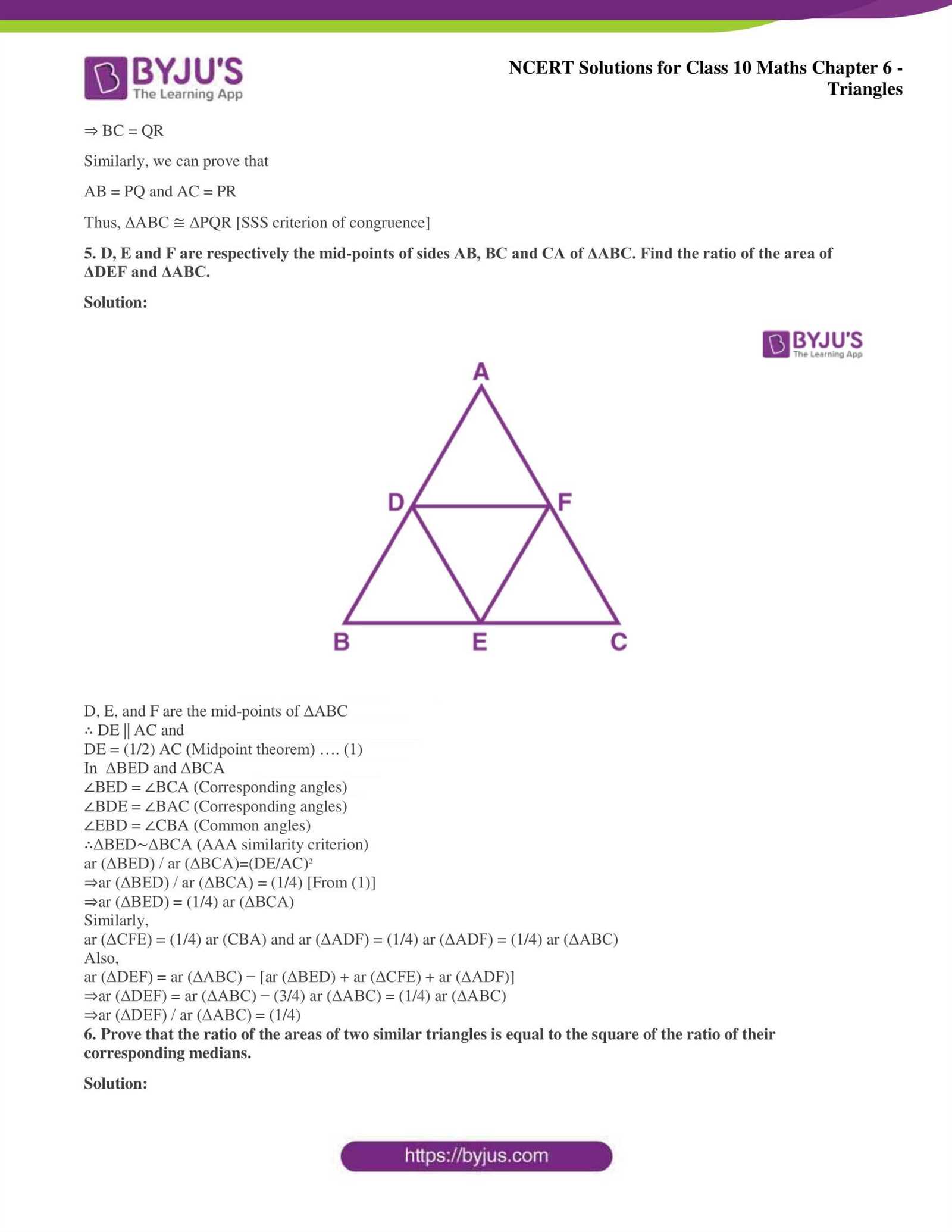
Once you understand the types of transformations, it’s important to practice applying them in different scenarios. Start by visualizing the transformations on a coordinate plane or diagram. Each transformation has a specific set of rules for determining the coordinates of the new figure. Familiarizing yourself with these rules and practicing with various examples will help you become proficient in using transformations to solve problems effectively.
Exploring Triangle Properties in Detail
Triangles are fundamental shapes in mathematics, and understanding their properties is essential for solving various problems. Each type of triangle has its unique characteristics that can be used to derive relationships between angles, sides, and other elements. By analyzing these properties, you gain a deeper insight into their behavior and applications in both theoretical and practical scenarios.
One important aspect of triangles is the relationship between their sides and angles. For example, the sum of the interior angles of any triangle always equals 180 degrees. Additionally, triangles can be classified based on side lengths and angle measures, which determine their specific properties.
| Type of Triangle | Properties |
|---|---|
| Equilateral | All sides and angles are equal. Each angle measures 60 degrees. |
| Isosceles | Two sides are equal, and the angles opposite these sides are congruent. |
| Scalene | All sides and angles are of different lengths and measures. |
| Right | One angle measures exactly 90 degrees, and the sides follow the Pythagorean theorem. |
By exploring these properties in detail, you will be better equipped to identify and solve various types of triangle-related problems, applying specific rules and theorems to find solutions with precision.
Working with Circles and Arcs
Understanding how to work with circular shapes and their segments is vital in solving various geometric problems. A circle, with its center and radius, can be analyzed to uncover relationships between angles, lengths, and areas. Arcs, which are portions of the circumference, play a crucial role in many applications, especially when calculating distances and angles related to sectors and segments.
Key Concepts Involving Circles
When studying circles, it is essential to be familiar with key concepts such as:
- Radius: The distance from the center of the circle to any point on the circumference.
- Diameter: The longest distance across the circle, passing through the center. It is twice the length of the radius.
- Circumference: The total distance around the circle, calculated using the formula C = 2πr.
Understanding Arcs and Their Properties
An arc is a section of the circle’s circumference, and its length depends on the central angle that subtends it. The formula to calculate the length of an arc is:
Arc length = (θ/360) * 2πr, where θ is the central angle in degrees and r is the radius of the circle.
Another important aspect of arcs is their relationship to sectors, which are portions of the area of the circle. The area of a sector can be calculated using the formula:
Area of sector = (θ/360) * πr².
Mastering these concepts will help you efficiently solve problems involving circular shapes and arcs, ensuring precise calculations and a deeper understanding of their properties.
Understanding Geometric Constructions
Geometric constructions involve creating shapes, angles, and lines using only a compass and straightedge. These fundamental techniques allow you to accurately replicate or design various geometric figures. Understanding how to perform these constructions helps develop a deeper understanding of geometric properties and relationships, which are essential for solving many types of problems.
There are several key tools and steps involved in performing precise constructions. By learning the basic methods, you can construct figures such as bisectors, perpendicular lines, and various types of polygons with confidence.
Essential Tools for Constructions
The two primary tools used in geometric constructions are:
- Compass: Used to draw arcs and circles, which help in measuring distances and creating equidistant points.
- Straightedge: Used to draw straight lines connecting points or forming line segments.
Common Constructions and Techniques
Some of the most common constructions that are often practiced include:
- Bisecting an Angle: Dividing an angle into two equal parts using a compass and straightedge.
- Constructing Perpendicular Lines: Creating a line that is at a 90-degree angle to a given line at a specific point.
- Constructing an Equilateral Triangle: Drawing a triangle with three equal sides, all angles measuring 60 degrees.
- Copying a Segment: Replicating the length of a given line segment using a compass.
By practicing these techniques, you can sharpen your skills and gain a clearer understanding of how geometric figures can be constructed precisely. Mastery of these constructions provides the foundation for more complex problems and mathematical reasoning.
Angles and Their Relationships Explained
Angles are fundamental components of many geometric figures, and understanding their relationships is key to solving a wide range of problems. Angles can be classified in various ways, and their interactions, whether complementary, supplementary, or vertical, reveal interesting properties that are useful for problem-solving. Recognizing how angles relate to one another can simplify the process of finding unknown angles and make complex geometric reasoning more intuitive.
Types of Angles
There are several types of angles, each with unique properties:
- Acute Angle: An angle smaller than 90 degrees.
- Right Angle: An angle that measures exactly 90 degrees.
- Obtuse Angle: An angle larger than 90 degrees but smaller than 180 degrees.
- Reflex Angle: An angle larger than 180 degrees but smaller than 360 degrees.
Key Angle Relationships
Understanding the relationships between angles helps to solve problems more efficiently. Some of the most common relationships include:
- Complementary Angles: Two angles that add up to 90 degrees.
- Supplementary Angles: Two angles that sum to 180 degrees.
- Vertical Angles: Angles that are opposite each other when two lines intersect. These angles are always equal.
- Adjacent Angles: Two angles that share a common side and vertex, but do not overlap.
By identifying and applying these relationships, it becomes easier to solve for unknown angles in a variety of geometric problems. Understanding how angles interact with one another not only simplifies calculations but also strengthens overall geometric reasoning.
Coordinate Geometry Insights
Coordinate geometry blends algebra and geometry, allowing you to explore the relationships between points, lines, and shapes on a plane using numerical coordinates. By working with coordinates, geometric figures can be described algebraically, and problems that would otherwise require complex constructions can be solved with simple equations. This approach is particularly useful for solving problems involving distances, midpoints, slopes, and other essential concepts in spatial relationships.
Understanding how to apply algebraic methods to geometric shapes enables you to tackle real-world problems involving positions and movements, such as navigation or modeling the layout of a city grid. The intersection of these two fields opens up powerful tools for analyzing and interpreting geometric figures mathematically.
Key Concepts in Coordinate Systems
The Cartesian coordinate system is the foundation of coordinate geometry, consisting of two perpendicular axes:
- x-axis: The horizontal axis, representing the first coordinate in an ordered pair.
- y-axis: The vertical axis, representing the second coordinate in an ordered pair.
Points on the plane are represented as ordered pairs (x, y), where x is the distance from the vertical axis and y is the distance from the horizontal axis.
Important Applications of Coordinate Geometry
Several key concepts in coordinate geometry can help solve various geometric problems:
- Distance Formula: The distance between two points (x₁, y₁) and (x₂, y₂) is given by the formula:
d = √((x₂ – x₁)² + (y₂ – y₁)²). - Midpoint Formula: The midpoint between two points (x₁, y₁) and (x₂, y₂) is calculated as:
Midpoint = ((x₁ + x₂)/2, (y₁ + y₂)/2). - Slope Formula: The slope of a line passing through points (x₁, y₁) and (x₂, y₂) is:
m = (y₂ – y₁) / (x₂ – x₁), which measures the steepness of the line.
These tools are invaluable for solving a wide range of problems involving lines, distances, and angles, and they form the core of coordinate geometry applications.
Practice Problems for Mastery
To truly master any mathematical concept, regular practice is essential. Working through problems not only reinforces key principles but also sharpens problem-solving skills. By applying theoretical knowledge to different types of exercises, learners can gain a deeper understanding and enhance their ability to tackle even the most complex challenges. Below are a series of practice problems designed to test various skills and concepts, helping you solidify your grasp on essential techniques.
Basic Problems
Start with these foundational problems to strengthen your understanding of core principles:
| Problem | Solution Method |
|---|---|
| Find the distance between points (2, 3) and (5, 7). | Apply the distance formula: d = √((x₂ – x₁)² + (y₂ – y₁)²). |
| Determine the midpoint of the points (4, -2) and (6, 2). | Use the midpoint formula: Midpoint = ((x₁ + x₂)/2, (y₁ + y₂)/2). |
| Find the slope of the line passing through (1, 1) and (3, 5). | Use the slope formula: m = (y₂ – y₁) / (x₂ – x₁). |
Advanced Problems
Once you’re comfortable with the basics, try these more advanced exercises to deepen your knowledge:
| Problem | Solution Method |
|---|---|
| Prove that the points (3, 4), (5, 6), and (7, 8) are collinear. | Calculate the slopes between each pair of points and check if they are equal. |
| Find the equation of the line passing through (2, 4) and (5, 10). | Use the slope formula to find the slope, then use the point-slope form of the equation. |
| Calculate the area of a triangle with vertices at (1, 2), (4, 6), and (7, 3). | Use the determinant formula for the area of a triangle based on its vertices. |
By regularly working through these practice problems, you can build the skills needed to excel in more complex challenges. Consistency is key in developing a strong mathematical foundation.
Tips for Solving Equations
Solving equations involves a series of logical steps and strategies that allow you to find the unknown values efficiently. Whether you’re working with simple expressions or more complex formulas, having a systematic approach can significantly improve your problem-solving skills. Below are some useful tips that can help you tackle equations with confidence and precision.
1. Understand the Problem Thoroughly
Before diving into any calculations, make sure to read the problem carefully. Identify the given values, what you are solving for, and any relationships between variables. Understanding the context of the equation will guide your approach and help prevent mistakes.
2. Organize Your Work
Keep your work neat and structured. Writing down each step clearly ensures you don’t miss important details and makes it easier to spot errors. Additionally, showing your work will allow you to revisit steps if you need to troubleshoot or verify your solution.
3. Isolate Variables
When solving for a specific variable, your goal is to isolate it on one side of the equation. Use basic algebraic operations such as addition, subtraction, multiplication, or division to manipulate the equation until the variable stands alone.
4. Check Your Work
Once you’ve arrived at a solution, it’s essential to check your work. Substitute your solution back into the original equation to see if it satisfies the given conditions. This step helps ensure your answer is correct and accurate.
5. Look for Patterns
Many equations follow established patterns or properties, such as symmetry or certain identities. Recognizing these patterns can simplify your process and help you solve equations more quickly.
6. Break Complex Problems into Smaller Steps
If you’re faced with a complicated equation, break it down into smaller, more manageable steps. Tackling the equation piece by piece will prevent you from feeling overwhelmed and increase your chances of success.
By incorporating these strategies into your approach, you’ll enhance your ability to solve equations efficiently and with greater accuracy. Practice these methods regularly, and you’ll gain more confidence in your problem-solving skills.
Visualizing 2D and 3D Shapes
Being able to visualize both two-dimensional and three-dimensional objects is an essential skill in solving problems and understanding spatial relationships. Whether you’re working with flat surfaces or complex structures, having a mental image of the shape in question can significantly improve your ability to analyze and manipulate it. This section will explore techniques and strategies for effectively visualizing these types of objects, enabling you to solve problems with greater accuracy.
Understanding 2D Figures

Two-dimensional shapes are flat and consist only of length and width. Some common 2D figures include squares, rectangles, triangles, and circles. To better visualize these shapes, consider their properties such as angles, sides, and symmetries. Drawing the shapes on paper and using graph paper can help you see their relationships and understand their dimensions more clearly. It’s also helpful to break down complex shapes into simpler components, making them easier to manipulate and understand.
Exploring 3D Shapes
Three-dimensional shapes, in contrast, have depth in addition to length and width. Examples include cubes, spheres, pyramids, and cones. Visualizing 3D objects can be more challenging because they require a deeper understanding of perspective and spatial relationships. One way to tackle this challenge is to draw the shape from multiple viewpoints, allowing you to see how its faces, edges, and vertices connect. Using models or virtual tools can also provide a clearer understanding of the object’s structure and help you mentally rotate the shape to view it from different angles.
With consistent practice, you can develop a stronger ability to visualize both 2D and 3D objects. This skill will enhance your problem-solving capabilities and improve your overall understanding of spatial concepts.
How to Check Your Solutions
Ensuring that your solutions are accurate is a critical part of solving mathematical problems. After completing a calculation or proof, it’s essential to review and verify each step to confirm the validity of your results. This process helps to identify potential mistakes, solidify your understanding, and refine your problem-solving skills. Below are some strategies for systematically checking your solutions to ensure their correctness.
Verification Techniques
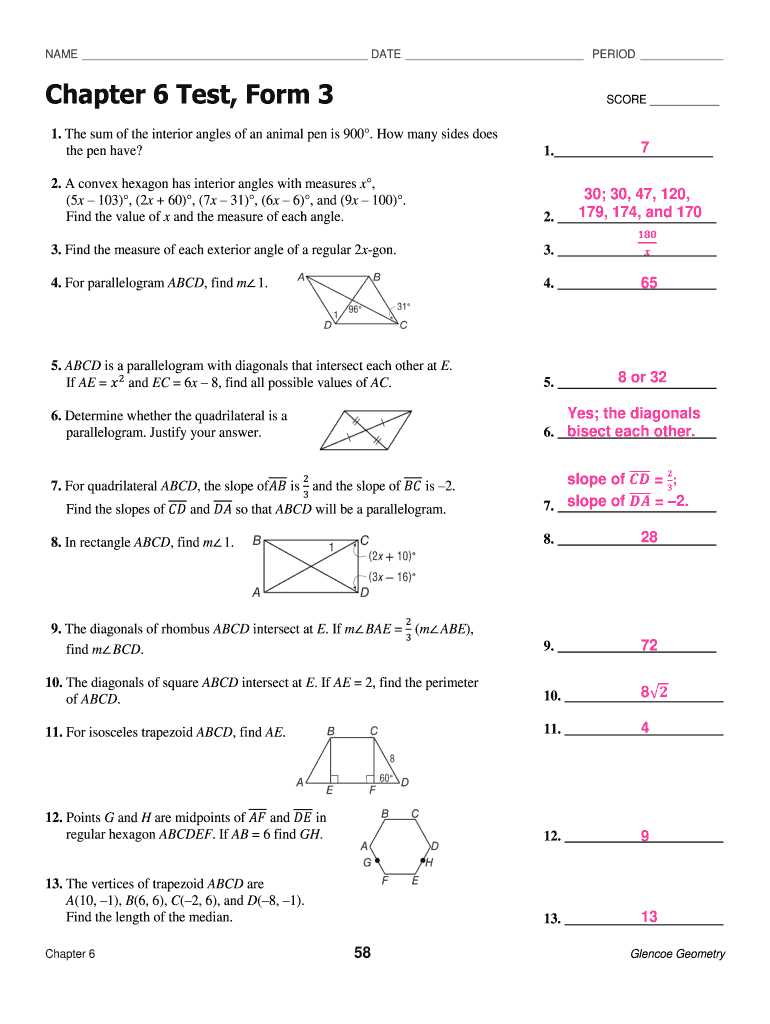
One of the best ways to check your solutions is through multiple approaches. For example, if you’ve solved for an unknown value, try plugging it back into the original equation or figure to verify if it satisfies all conditions. Alternatively, use a different method or formula to solve the same problem and compare the results.
Common Strategies for Verification
| Strategy | Description |
|---|---|
| Substitution | Substitute your solution back into the original expression to check if it satisfies the equation or conditions. |
| Estimation | Estimate the expected result to check if your solution is reasonable. If it’s far off from the estimate, recheck your steps. |
| Cross-Verification | Use an alternative method or formula to solve the problem and compare the two results for consistency. |
| Reviewing Assumptions | Ensure that your assumptions and conditions are correctly applied, and that no steps were skipped or misunderstood. |
By following these verification strategies, you can strengthen your problem-solving abilities and avoid common mistakes. This will help ensure that your solutions are both accurate and reliable.
Reviewing Vocabulary from Section 6
Understanding key terminology is essential for grasping the concepts covered in any mathematical section. Whether solving problems, constructing proofs, or interpreting diagrams, being familiar with specific terms helps streamline the process and improves accuracy. This section highlights some of the most important terms to remember, along with their definitions, to ensure a solid understanding of the material.
Familiarizing yourself with these terms allows you to approach problems with confidence and communicate your ideas clearly. As you continue practicing, reinforcing this vocabulary will support your ability to apply these concepts effectively in various scenarios.
Here are some key terms you should keep in mind:
- Angle Bisector – A line or segment that divides an angle into two equal parts.
- Complementary Angles – Two angles that add up to 90 degrees.
- Supplementary Angles – Two angles that add up to 180 degrees.
- Parallel Lines – Two lines that never intersect and are always the same distance apart.
- Perpendicular Lines – Lines that intersect at a right angle (90 degrees).
- Congruent – Having the same size and shape, typically used to describe angles or segments.
- Radius – The distance from the center of a circle to any point on its circumference.
- Diameter – A straight line passing through the center of a circle, touching both sides of the circumference.
- Chords – A line segment joining two points on the circumference of a circle.
- Circumference – The perimeter or boundary line of a circle.
By reviewing and mastering this vocabulary, you will be better equipped to navigate more advanced topics and apply the principles in different contexts. Understanding these terms will also improve your ability to solve related problems with greater ease and precision.