
In the early stages of elementary education, students encounter a variety of fundamental concepts that serve as building blocks for more complex topics. This section is designed to guide students through these critical ideas, providing clear steps for solving problems and understanding key principles. With the right tools, students can navigate these challenges with confidence and ease.
Step-by-step explanations and practice exercises are key components of this approach, offering a detailed breakdown of the most important lessons. By focusing on clarity and simplicity, learners can grasp essential concepts and apply them effectively in various situations.
As you explore this section, you’ll find various strategies and insights to enhance your understanding. Whether tackling basic principles or more advanced tasks, these solutions aim to foster deeper learning and build a strong foundation for future success.
Go Math Grade 5 Chapter 1 Guide

This section provides essential guidance for understanding the foundational concepts introduced early in the curriculum. By focusing on core skills, students can build confidence in solving problems and applying their knowledge. The goal is to establish a solid grasp of basic principles that will support more advanced learning in the future.
Each topic is broken down into manageable steps, helping learners comprehend the material through clear explanations. With practice, students will be able to tackle a variety of exercises and approach problems systematically, ensuring a deeper understanding of the content.
These solutions aim to make the learning process engaging and approachable, offering students the tools they need to succeed. By following this guide, learners will gain a comprehensive understanding of the key concepts necessary for mastering the material.
Key Concepts in Chapter 1
This section covers the essential ideas that serve as the foundation for students’ understanding of the subject. By focusing on the core principles introduced at the beginning of the curriculum, learners will develop a strong grasp of critical skills and techniques. These concepts are the building blocks for more complex topics, making it important to fully understand them before moving forward.
Fundamental Problem-Solving Skills
One of the key areas of focus is learning how to approach and solve problems systematically. This involves breaking down complex tasks into simpler steps and applying logical reasoning to find solutions. Students are encouraged to practice various strategies to enhance their problem-solving abilities.
Understanding Core Principles
Another essential concept in this section is the application of core principles that govern the subject. These foundational ideas are presented in a clear and straightforward manner, ensuring that students can easily grasp their significance and use them to solve problems effectively. By mastering these principles, students will be prepared for more challenging material ahead.
How to Solve Chapter 1 Problems
In this section, we focus on effective strategies for tackling problems that are introduced early in the course. Understanding how to break down each task into smaller, more manageable steps is key to solving these types of exercises successfully. By following a structured approach, students can methodically work through each problem with confidence and accuracy.
Step-by-step methods are crucial in ensuring that no important detail is overlooked. Start by carefully reading the problem to fully understand what is being asked. Once the objective is clear, identify the necessary information and determine the best approach to solve it. Breaking the task into smaller parts will often make it easier to solve, especially when dealing with multi-step problems.
Practice and repetition are vital for improving problem-solving skills. By consistently applying these strategies, students will not only master the concepts presented but also develop greater confidence in their ability to tackle similar challenges in the future.
Understanding Go Math Grade 5 Methods
This section explores the methods and strategies used in the curriculum to help students grasp important concepts. By applying a combination of visual aids, step-by-step instructions, and practice exercises, learners are encouraged to develop a deeper understanding of each topic. The focus is on making abstract ideas more accessible and practical for students to apply in various contexts.
Key Approaches for Learning
The method used focuses on presenting problems in a variety of ways, ensuring that students can approach each one from different angles. This includes using illustrations, manipulatives, and hands-on activities that make abstract concepts more concrete and understandable. Through repeated practice, students can strengthen their problem-solving skills and build confidence in their abilities.
Building a Strong Foundation

By following these methods, learners gradually build a strong foundation in core principles, allowing them to move on to more complex tasks with ease. The structured approach ensures that each student has the support they need to succeed while encouraging independent thinking and problem-solving.
Helpful Tips for Chapter 1 Success
To excel in the initial lessons, it’s important to approach each problem with a clear strategy and a positive mindset. Developing a strong understanding of basic concepts will help you build a solid foundation for future topics. Consistent practice and focused attention are key to mastering the skills introduced early on.
Stay Organized and Focused
One of the most effective ways to succeed is to stay organized. Break down each task into smaller steps and approach them one at a time. This will not only make each problem more manageable but also help ensure that no important details are missed. Staying focused will allow you to retain the information better and solve problems more efficiently.
Practice Regularly
Regular practice is essential for reinforcing what you’ve learned. The more you work with the material, the more comfortable you’ll become with it. Take time to review any concepts that are unclear and try additional problems to improve your skills. This repetition will help deepen your understanding and build confidence in your abilities.
Step-by-Step Solutions for Chapter 1
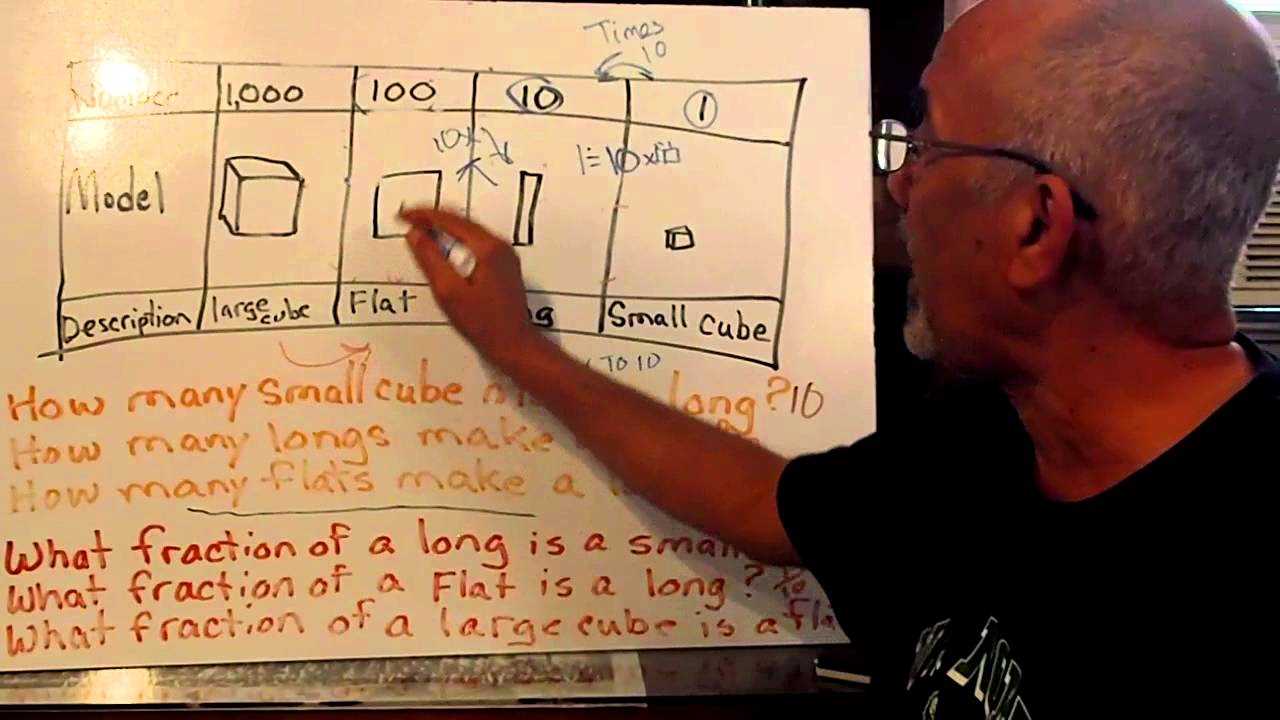
This section provides detailed, step-by-step solutions to help students fully understand the process of solving problems introduced at the beginning of the course. By breaking down each exercise into manageable steps, learners can follow a clear path to the solution and grasp the reasoning behind each step. The goal is to demystify the problem-solving process and ensure that every student has the tools to succeed.
Breaking Down the Problem
Each problem is first analyzed to identify the key elements and what needs to be solved. It’s important to clearly understand the goal of the question before proceeding. Once the task is understood, the necessary steps are outlined, ensuring a logical progression toward the solution. Taking this approach makes each problem more approachable and easier to handle.
Applying the Correct Strategy
In this section, you’ll also find the application of specific strategies that help solve different types of problems effectively. Whether it’s using a formula, working through visual aids, or applying estimation techniques, these strategies will guide you through each problem systematically. With practice, students will be able to approach similar tasks with confidence and speed.
Common Mistakes in Go Math Chapter 1
As students begin to work through early lessons, it’s common to make certain errors that can hinder progress. Recognizing these mistakes early on allows learners to correct them before they become habits. This section highlights the most frequent errors in the initial exercises, offering tips on how to avoid them and ensuring better understanding of the material.
Frequent Errors in Problem Solving
Many students encounter challenges when solving problems, often overlooking important details or misapplying concepts. Here are some of the most common mistakes:
- Misunderstanding the Problem: Skipping over key information or misinterpreting the question can lead to incorrect answers.
- Rushing Through Steps: Not taking the time to carefully break down each part of the task can result in errors in calculation or logic.
- Incorrectly Applying Formulas: Forgetting to follow the correct order of operations or misapplying a formula can lead to mistakes.
- Skipping Practice: Not practicing enough can lead to difficulty understanding more complex concepts that build on the basics.
How to Avoid These Mistakes
To avoid these pitfalls, take a methodical approach to each problem. Carefully read the instructions and highlight important information. Break the task into smaller steps and verify each one before proceeding. Regular practice is essential, as is reviewing any errors made to understand where things went wrong.
Strategies for Mastering Chapter 1

Mastering the initial lessons requires a focused approach, using a combination of effective strategies that help reinforce understanding and build confidence. By following a structured plan and applying proven techniques, students can strengthen their grasp of essential skills and improve their problem-solving abilities. Consistent practice and careful review are key elements in achieving success.
| Strategy | Description |
|---|---|
| Break Down Problems | Divide each problem into smaller, more manageable steps to ensure clarity and avoid confusion. |
| Practice Regularly | Set aside time each day to work through practice problems and reinforce what has been learned. |
| Visualize Concepts | Use diagrams or visual aids to help understand abstract ideas and make them more tangible. |
| Review Mistakes | After completing exercises, go over any errors to understand where and why mistakes were made. |
| Use Real-Life Examples | Relate problems to real-world situations to better understand their practical applications. |
By implementing these strategies, students can develop a deeper understanding of the material and be better prepared to tackle more advanced topics in the future.
How Chapter 1 Builds Math Skills
The first section of the course is designed to lay the groundwork for key abilities that students will build upon as they progress. It focuses on developing critical thinking and problem-solving strategies that are essential for mastering more complex topics. By mastering the concepts introduced early on, students strengthen their overall skills and become more confident in applying them to various situations.
| Skill Developed | How It Is Built |
|---|---|
| Logical Thinking | Students learn how to approach problems methodically, identifying patterns and using logical steps to find solutions. |
| Problem-Solving | Breaking down tasks into smaller, manageable parts helps learners develop strategies for solving complex problems. |
| Understanding Relationships | By studying how different elements relate to each other, students gain a deeper understanding of fundamental principles. |
| Attention to Detail | Students practice focusing on small details, ensuring that every part of a problem is addressed correctly. |
| Application of Knowledge | The section emphasizes how concepts can be applied to real-life situations, reinforcing the relevance of what is being learned. |
Through consistent practice and application of these skills, students build a strong foundation that will serve them well as they tackle more advanced material in future lessons.
Breaking Down Complex Problems
When faced with difficult tasks, it’s essential to break them down into smaller, more manageable steps. By doing this, the solution becomes clearer, and the problem seems less overwhelming. This approach not only simplifies the process but also helps in focusing on one element at a time, which ensures that no detail is overlooked. In this section, we’ll explore methods to tackle complex problems efficiently and systematically.
Step-by-Step Breakdown
One of the most effective ways to solve a challenging problem is to divide it into smaller parts. This method allows you to focus on solving each part individually before combining them for the final solution. Below is a table that outlines a common approach to breaking down complex tasks.
| Step | Action |
|---|---|
| 1 | Read the problem carefully and identify the main question. |
| 2 | Highlight or underline key information that will help solve the problem. |
| 3 | Break the problem into smaller tasks, solving each part individually. |
| 4 | Check each step for accuracy before moving on to the next. |
| 5 | Combine the results of each step to reach the final solution. |
Visualizing the Problem
Another powerful method for tackling complex problems is to visualize the task. Creating diagrams, charts, or other visual aids can help you see connections between different parts of the problem, making it easier to understand the relationships and work toward a solution. Visualizing the process also helps retain information and makes the task more engaging.
Essential Skills from Chapter 1
In the first section of the course, students are introduced to key abilities that are essential for success in more advanced topics. Mastering these skills provides a solid foundation for future learning and helps students develop a deeper understanding of core concepts. These essential skills include problem-solving techniques, logical reasoning, and the ability to apply learned principles to real-world scenarios.
Core Skills Developed
- Logical Thinking: Learning how to approach problems with a clear and structured method.
- Problem Decomposition: Breaking down complex tasks into smaller, more manageable steps.
- Pattern Recognition: Identifying patterns and relationships between numbers or elements in a problem.
- Attention to Detail: Focusing on each part of a problem to avoid missing critical information.
- Application of Concepts: Using learned principles to solve real-life problems and make connections between theory and practice.
How These Skills Support Future Learning
Developing these skills is crucial because they lay the groundwork for tackling more difficult topics later on. By mastering logical thinking and problem-solving, students can approach more complex challenges with confidence and precision. These skills also enhance critical thinking and help learners become more independent, allowing them to handle new material more effectively.
How to Use the Answer Key Effectively

Using solutions effectively is an important part of the learning process. It can guide students toward understanding where they went wrong, improve their problem-solving skills, and reinforce the correct methods. However, it’s crucial to use these resources as tools for learning rather than just for checking answers. By following a strategic approach, students can maximize their understanding and avoid relying on solutions too heavily.
Steps to Use Solutions for Better Understanding
- Attempt Problems Independently: Always try to solve problems on your own first before referring to the solutions. This helps develop critical thinking and problem-solving skills.
- Check Step-by-Step: If your solution differs from the provided one, carefully compare each step to identify where you made a mistake.
- Understand the Process: Focus on understanding the reasoning behind each step of the solution rather than just memorizing the final result.
- Learn from Mistakes: Use the differences between your work and the solution to understand why you made a mistake and how to avoid it in the future.
- Review Regularly: Going over your mistakes periodically will help reinforce the correct methods and strategies.
Benefits of Using Solutions Wisely

- Boosts Self-Confidence: Successfully applying the right strategies after reviewing solutions builds confidence.
- Improves Problem-Solving Skills: By learning the correct approach through step-by-step analysis, students improve their ability to solve similar problems in the future.
- Enhances Understanding: Using solutions as a learning tool helps reinforce concepts and clarifies any confusion from previous attempts.
Practice Exercises for Chapter 1
Engaging in regular practice is essential for mastering the concepts introduced in the initial section of the course. These exercises provide an opportunity to reinforce learning, build confidence, and identify areas that need improvement. By working through a variety of problems, students can strengthen their understanding and improve their problem-solving abilities.
Recommended Practice Problems
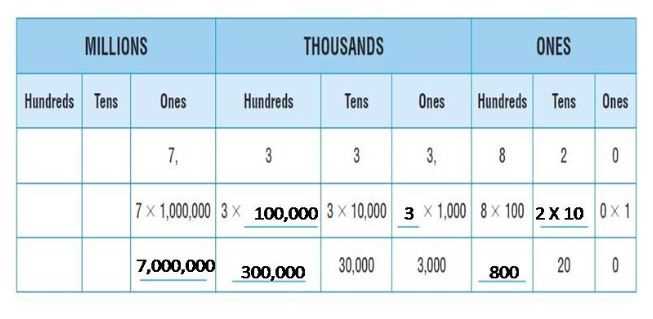
- Problem 1: Solve a set of basic operations involving whole numbers. Focus on accuracy and understanding the methods used.
- Problem 2: Complete a series of word problems that require applying learned concepts to real-life scenarios.
- Problem 3: Practice with multiple-step problems to improve problem-solving skills and increase mental flexibility.
- Problem 4: Review patterns and relationships in numerical problems to identify connections between different concepts.
- Problem 5: Apply the learned strategies to solve problems involving basic algebraic thinking.
Tips for Effective Practice
- Start Small: Begin with simpler problems to build a solid foundation before progressing to more complex ones.
- Work Consistently: Make practice a daily habit to reinforce learning and retain information over time.
- Check Your Work: After completing an exercise, review your steps and identify any mistakes to learn from them.
- Ask for Help: If you encounter difficulties, don’t hesitate to seek help from teachers or peers to clarify your understanding.
Key Formulas from Chapter 1

In the first section of the course, several important formulas and principles are introduced to help solve a variety of problems. These formulas are fundamental tools that students will rely on throughout their studies. Understanding and memorizing these key expressions is essential for building a strong foundation in problem-solving and logical reasoning.
Below are some of the most crucial formulas introduced in this section, which students should familiarize themselves with and apply regularly.
- Formula 1: Area of a Rectangle – The area of a rectangle can be calculated by multiplying the length by the width.
Area = Length × Width - Formula 2: Perimeter of a Rectangle – The perimeter of a rectangle is the sum of all its sides.
Perimeter = 2 × (Length + Width) - Formula 3: Volume of a Rectangular Prism – The volume of a rectangular prism is found by multiplying its length, width, and height.
Volume = Length × Width × Height - Formula 4: Average – The average of a set of numbers is calculated by adding them together and dividing by the total number of values.
Average = (Sum of all values) ÷ (Number of values) - Formula 5: Simple Ratio – Ratios represent the relationship between two numbers. To simplify a ratio, divide both terms by their greatest common divisor.
Ratio = (Value 1) ÷ (Value 2)
Mastering these formulas is key to progressing through the course with confidence. Regular practice will help reinforce their application in solving various types of problems, and they will form the basis for more advanced topics in future lessons.
Go Math Approach to Problem Solving
The approach introduced in the first section focuses on breaking down complex problems into manageable steps. This method emphasizes understanding the problem, strategizing an approach, and applying basic principles to find solutions. By focusing on clarity and systematic reasoning, students are encouraged to think critically and work through challenges step by step.
Here are the key strategies emphasized for solving problems efficiently:
- Read Carefully: Always begin by thoroughly reading the problem to understand what is being asked. Identify the important information and variables.
- Plan the Solution: Before jumping into calculations, decide on the best approach. Consider what tools or formulas will help solve the problem.
- Work Step-by-Step: Break the problem into smaller, more manageable steps. This makes it easier to identify and correct mistakes early.
- Check Your Work: After finding a solution, review each step to ensure the calculations are correct and make logical sense in the context of the problem.
- Use Visuals: Sometimes drawing diagrams, charts, or graphs can help make the problem clearer and provide a visual representation of the solution.
- Practice Regularly: The more problems you solve, the better you will understand the methods and develop your problem-solving skills.
Following this structured approach not only helps in solving problems but also builds a strong foundation for tackling more advanced topics. Regular practice and application of these strategies will foster confidence and mastery in problem-solving.
Review of Chapter 1 Solutions
This section offers a comprehensive review of the solutions covered in the first part of the curriculum. By revisiting each problem and its corresponding solution, students can better understand the methods used and how to apply them in similar situations. The goal is to reinforce learning by identifying key steps and ensuring that the approach is clear and effective.
Key Steps in Problem-Solving
Each problem presented in the initial section involves a structured approach that starts with understanding the question and ends with a solution verification. It’s important to break down each problem into smaller parts and solve step-by-step. This allows for clearer thinking and reduces the chances of making mistakes. Below are some of the common techniques applied:
- Identify the goal: What is being asked? Establish a clear understanding of the outcome required.
- Gather relevant information: Collect all necessary data from the problem statement before proceeding.
- Apply relevant methods: Use the correct approach to process the information and arrive at a solution.
- Review your work: Double-check your calculations and logic to ensure accuracy.
Common Challenges and Solutions

While solving problems in this section, students may encounter difficulties in understanding the process or applying the correct strategy. It’s essential to recognize these challenges and approach them systematically:
- Misreading the problem: Always reread the problem and underline key information.
- Incorrect application of formulas: Ensure that the right formulas are used and check that each variable is properly identified.
- Skipping steps: Never skip any part of the process–each step contributes to the overall solution.
By addressing these challenges and reviewing the solutions, students can enhance their understanding and confidence in applying problem-solving techniques in future exercises.