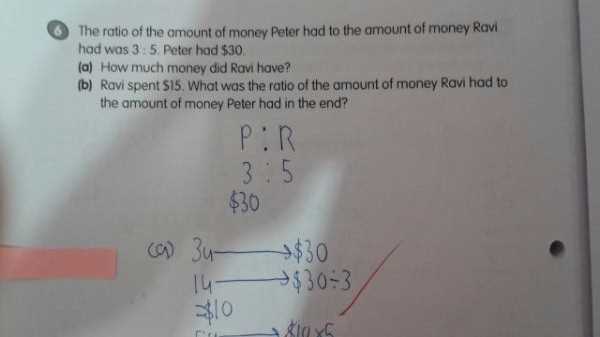
In this section, students will explore essential concepts that lay the foundation for more complex problem-solving. The focus is on developing a solid understanding of numerical relationships and logical reasoning. Through various exercises, learners will practice techniques that help them approach tasks with confidence and clarity.
As the exercises progress, it’s important to build accuracy and speed in solving challenges. This is achieved by applying learned strategies to both familiar and new scenarios. By reinforcing the basics, students prepare themselves for more advanced topics, while ensuring a comprehensive grasp of key principles.
Clear understanding of these core topics will enhance students’ ability to tackle problems efficiently. Practicing step-by-step solutions and checking work carefully are critical steps in mastering the material.
Success in these activities requires persistence and attention to detail, but with the right approach, students will find themselves more confident and capable in handling increasingly challenging tasks.
Go Math Grade 5 Chapter 3 Overview
This section introduces foundational concepts that are essential for solving more complex numerical problems. It focuses on developing an understanding of relationships between different values and applying various strategies to work through challenges. By breaking down the material into manageable steps, learners are encouraged to approach each task with both accuracy and confidence.
Students will explore several key areas, including operations with fractions, understanding patterns, and the ability to apply learned methods to real-world scenarios. Emphasis is placed on building both conceptual knowledge and procedural skills, ensuring that students are well-equipped to solve problems and strengthen their reasoning abilities.
Through structured practice and careful review, learners are encouraged to identify common pitfalls and reinforce their understanding. By mastering these core skills, students are better prepared for more advanced topics and confident in their ability to tackle future challenges.
Understanding Key Concepts in Chapter 3
This section focuses on essential principles that are crucial for tackling various numerical challenges. It covers important techniques and problem-solving strategies, encouraging students to build a deep understanding of each topic. Mastering these fundamental concepts will help learners apply their knowledge to a variety of tasks with greater ease and accuracy.
Key Skills to Master
In this section, students will learn how to manipulate numbers, interpret patterns, and solve complex problems involving fractions, decimals, and other essential calculations. Developing a solid grasp of these skills will allow students to handle progressively more difficult exercises and real-life applications with confidence.
Applying Concepts to Real-World Situations
It’s crucial to recognize how the learned strategies can be applied beyond the classroom. By solving real-world problems, students can see the relevance of their studies and how these concepts are used in everyday life. Understanding these connections strengthens their ability to reason through challenges and make well-informed decisions.
Step-by-Step Guide to Questions
To tackle any problem effectively, it’s important to break down each question into smaller, manageable parts. This approach helps students stay organized and focused, allowing them to apply the right strategies and solve challenges with precision. Following a step-by-step process ensures clarity in thought and reduces the chances of making mistakes.
Breaking Down the Problem
Begin by reading the problem carefully. Identify the key information provided and the specific question being asked. Highlight important numbers or terms that will guide you through the solution. This first step is crucial in setting the stage for the entire process.
Solving and Verifying the Solution
Once the problem is understood, proceed by applying the appropriate methods. Work through each step logically and ensure that all calculations are performed correctly. After completing the solution, always check your work. Reviewing the steps will help identify any errors and confirm that the solution is accurate.
Common Mistakes in Chapter 3 Tests
When solving numerical problems, it’s easy to make small errors that can lead to incorrect results. These mistakes often stem from misunderstanding the instructions, misapplying a method, or overlooking key details. Identifying and addressing these common pitfalls can greatly improve performance and accuracy in solving future challenges.
Common Errors to Watch For
Below are some of the most frequent mistakes that students make when working through questions in this section. Understanding these can help avoid confusion and enhance problem-solving skills.
| Type of Mistake | Example | How to Avoid |
|---|---|---|
| Misreading Instructions | Skipping crucial steps due to unclear understanding of the problem | Carefully read the instructions and underline important details |
| Calculation Errors | Incorrectly adding or subtracting numbers | Double-check each calculation before finalizing the answer |
| Overlooking Units | Forgetting to convert units or misinterpreting them | Pay attention to units and always convert them where necessary |
| Rushing | Making simple mistakes from working too quickly | Take your time, review your work, and avoid hasty decisions |
How to Improve Accuracy
The best way to avoid these common mistakes is through practice and careful review. By understanding where errors typically occur, students can take proactive steps to prevent them. Consistently checking each step in the process helps ensure more reliable results.
How to Approach Word Problems
Word problems often seem intimidating because they require translating a real-life scenario into a mathematical solution. However, by breaking the problem into smaller steps, it becomes easier to understand what is being asked and how to find the answer. The key is to approach these problems methodically, focusing on extracting the relevant information and applying the appropriate strategies.
Start by reading the problem carefully and identifying the main question. Look for keywords that suggest what operations are needed, such as “total,” “difference,” or “product.” Next, highlight any numbers or values given in the problem and think about how they relate to one another. After that, choose the right method to solve the problem, whether it’s addition, subtraction, multiplication, or division, based on the situation presented.
Once the initial approach is clear, solve the problem step-by-step, and always double-check your work. Don’t forget to interpret the answer in the context of the problem, ensuring it makes sense with the information provided.
Practice Questions for Chapter 3
To fully grasp the concepts covered in this section, it is essential to practice with a variety of problems. By working through different types of exercises, students can strengthen their problem-solving skills and increase their confidence in applying the techniques learned. Below are some sample problems that mirror the kinds of questions you might encounter, helping to reinforce the key ideas.
Problem Set 1
- Calculate the sum of 3/4 and 5/8. Simplify the result.
- Subtract 1/5 from 2/3. What is the difference?
- Multiply 4/7 by 3/5. What is the product?
- Divide 3/4 by 2/3. Simplify the answer.
Problem Set 2
- Jane has 3/4 of a cup of flour. She uses 1/2 of it for a recipe. How much flour does she have left?
- If a rectangle has a length of 3/5 meters and a width of 2/3 meters, what is the area of the rectangle?
- A garden has 7/10 of an acre planted with flowers. If 3/5 of that area is shaded, how much of the garden is shaded?
- A car travels 3/4 of a mile in 1/2 hour. How far will it travel in 1 hour at the same speed?
By solving these practice questions, students can deepen their understanding of key concepts and prepare for more challenging problems ahead. It’s important to work through each step methodically and check the results to ensure accuracy.
Tips for Preparing for Chapter 3 Test
Effective preparation is key to performing well in any assessment. To succeed, it’s important to focus on mastering the essential skills and strategies that will be tested. By reviewing core concepts, practicing regularly, and refining problem-solving techniques, students can approach the challenge with confidence. Below are several tips to help you prepare efficiently.
Review Key Concepts

- Go over all the main ideas discussed in the section, focusing on areas where you feel less confident.
- Revisit any practice problems you struggled with, ensuring you understand the correct methods to solve them.
- Make sure you understand how to apply the concepts in different contexts, not just in familiar scenarios.
Practice Consistently
- Work through sample problems regularly to reinforce your understanding.
- Challenge yourself with problems that test different aspects of the material to ensure you are prepared for any variation.
- Time yourself while practicing to improve your speed and ensure you can solve problems within the required timeframe.
Ask for Help
- If you’re stuck on a particular concept or problem, don’t hesitate to ask for clarification from a teacher or peer.
- Join study groups to discuss tricky questions and share different approaches to solving them.
Stay Organized
- Keep all notes and practice materials organized, so you can easily review them when needed.
- Create a study schedule to ensure you are covering all the material before the assessment.
By following these strategies and staying consistent in your preparation, you’ll be well-equipped to succeed when it’s time to tackle the assessment. Remember to stay calm and confident, knowing that you’ve put in the work to be prepared.
Reviewing Core Skills for Grade 5
To build a strong foundation in this subject, it’s essential to review and reinforce the core abilities that are fundamental at this level. These skills are not only critical for excelling in assessments but also for applying knowledge in everyday situations. A thorough review of the basics will ensure greater confidence and understanding in more advanced topics.
Essential Skills to Focus On
Key concepts that should be revisited include operations with fractions, decimals, and understanding measurements. By practicing these skills, students can strengthen their ability to solve a variety of problems efficiently and accurately.
| Skill Area | Focus | Practice Examples |
|---|---|---|
| Fractions | Adding, subtracting, multiplying, and dividing fractions | 1/4 + 3/8, 5/6 – 2/3, 3/4 x 2/5 |
| Decimals | Converting decimals to fractions and performing operations | 0.75 + 0.25, 1.4 x 0.5 |
| Measurement | Understanding units of length, weight, and volume | Converting between units (cm to meters), finding the area of simple shapes |
Practical Application of Concepts
It’s crucial to not only practice these basic skills but also apply them to real-world scenarios. For example, converting fractions when measuring ingredients for a recipe, or using decimals when shopping or budgeting. This approach helps solidify understanding and prepares students for practical problem-solving.
How to Solve Multi-Step Problems

Multi-step problems require a strategic approach, as they involve more than one operation or calculation. These types of problems can seem overwhelming at first, but by breaking them down into manageable parts, the solution becomes clearer. The key to solving them is to approach each step methodically, ensuring that all necessary information is used at every stage.
To successfully solve multi-step problems, follow a structured process that includes identifying the operations needed, organizing the information, and solving step-by-step. Start by carefully reading the problem and highlighting important details. Then, identify the first operation required, solve it, and move on to the next until the problem is fully resolved.
| Step | Action | Example |
|---|---|---|
| Step 1 | Read and understand the problem | Identify what the problem is asking and the key information |
| Step 2 | Identify operations | Determine if addition, subtraction, multiplication, or division is needed |
| Step 3 | Break into smaller parts | Divide the problem into parts that can be solved individually |
| Step 4 | Solve each part | Start with the first calculation and continue solving step-by-step |
| Step 5 | Double-check your work | Ensure that each step is correct before moving to the next one |
By following these steps, you can approach multi-step problems with confidence, ensuring accuracy and efficiency in finding the solution. Consistent practice will improve your ability to break down complex problems and solve them effectively.
Strategies for Improving Accuracy
Improving accuracy in solving problems is a crucial skill that enhances overall performance. It involves paying attention to detail, avoiding careless errors, and applying a systematic approach. With the right strategies, students can boost their precision and confidence, ensuring that each problem is solved correctly the first time.
One effective method for improving accuracy is to always double-check each step of the process. By reviewing work, students can catch small mistakes before they affect the final result. Additionally, understanding the logic behind each step helps reinforce the correct approach and minimizes the chance of overlooking important information.
Another strategy is to break complex problems into smaller, more manageable parts. This approach helps students focus on one calculation or operation at a time, reducing the risk of confusion or errors. Writing out each step also provides a clear path to follow, making it easier to track progress and identify any mistakes along the way.
Key Techniques for Accuracy
- Read the problem carefully before starting. Ensure you understand what is being asked.
- Work slowly and methodically, especially when dealing with multiple operations.
- Check your work after each step, not just at the end.
- Use estimation to verify if your final answer makes sense.
- Practice regularly to build familiarity with different types of problems.
Consistency is the key to mastering accuracy. The more frequently these techniques are practiced, the more natural they will become, ultimately improving overall problem-solving efficiency.
Mastering Fractions and Decimals
Understanding fractions and decimals is essential for solving a wide range of problems in everyday life. These two concepts form the foundation for many advanced mathematical skills and are key to improving problem-solving abilities. By mastering the rules and relationships between fractions and decimals, students can enhance their overall ability to perform calculations efficiently and accurately.
To become proficient, it’s important to practice converting between fractions and decimals, as well as performing operations like addition, subtraction, multiplication, and division. Once these core operations are understood, applying them in different contexts becomes much easier. A strong grasp of these concepts will not only improve performance in exams but also build confidence when facing real-world situations that require numerical reasoning.
Converting Between Fractions and Decimals
Converting between fractions and decimals is a valuable skill that can be applied to various types of problems. To convert a fraction to a decimal, divide the numerator by the denominator. For example, converting 3/4 to a decimal would involve dividing 3 by 4, resulting in 0.75. Conversely, to convert a decimal into a fraction, place the decimal number over its place value and simplify if needed. For instance, 0.5 can be written as 1/2.
Operations with Fractions and Decimals
Performing operations with fractions and decimals requires different techniques. When adding or subtracting fractions, it’s important to have a common denominator. With decimals, align the decimal points before performing the operation. Practice with both types of numbers can help solidify the understanding of their behavior in various mathematical operations.
Using Visual Aids in Chapter 3

Visual aids play a crucial role in enhancing understanding and retention of complex concepts. They provide a clear and engaging way to illustrate abstract ideas, making it easier for learners to grasp difficult material. By using diagrams, charts, and other visual tools, students can break down information into more manageable parts and better understand how different elements of a problem are related.
Incorporating visual aids into the learning process can help students make connections between numbers and concepts, especially when dealing with topics that require visual representation, such as comparing quantities or identifying patterns. These tools serve not only as instructional aids but also as reminders of key concepts, helping learners recall critical information when solving problems.
Types of Visual Aids
There are various types of visual aids that can be used to enhance learning. Some of the most effective include:
- Bar graphs and line graphs – These are useful for representing data and understanding relationships between variables.
- Pie charts – Great for illustrating parts of a whole, helping to visualize proportions and percentages.
- Number lines – Excellent for comparing and ordering numbers, especially when working with fractions or decimals.
- Tables – Useful for organizing information systematically and making it easier to analyze and compare different sets of data.
Benefits of Using Visual Aids
Using visual aids not only makes learning more engaging but also reinforces concepts through multiple modes of understanding. When students see the information visually, it helps them make connections between abstract numbers and real-world applications. This multi-sensory approach supports different learning styles, especially for visual learners, making it easier for them to retain and apply new knowledge.
Time Management Tips for Math Tests
Effective time management is essential when preparing for and taking any type of evaluation. It helps ensure that you can complete all the questions accurately while maintaining a steady pace throughout the process. By managing your time wisely, you can reduce anxiety, avoid rushing, and give yourself the best chance for success.
Developing a strategy for allocating time to each section of the assessment and knowing when to move on from a question can make all the difference. With careful planning and practice, students can improve both their speed and accuracy during evaluations. Below are some practical time management tips to help maximize performance.
Prioritize the Easier Questions
Start by answering the questions that you find easiest. This will help you build confidence and ensure that you don’t run out of time on simpler tasks. Tackling the more straightforward problems first allows you to secure points before moving on to the more challenging ones.
Use Time Wisely for Each Section

Break down the available time according to the sections of the evaluation. Here are a few steps you can follow:
- Divide the total time: Estimate how long to spend on each section based on its difficulty and the number of questions.
- Keep track of time: Regularly check the clock to ensure that you are staying within your allotted time for each section.
- Don’t get stuck: If you’re stuck on a difficult question, move on and return to it later. Spending too much time on one question can lead to missing easier ones.
Practice Under Timed Conditions
One of the best ways to improve your time management skills is by practicing under timed conditions. Use sample questions or past evaluations to simulate the actual testing experience. This will help you get a feel for pacing and make you more comfortable with the time constraints.
By mastering these strategies, you’ll be able to manage your time more effectively and perform your best during any assessment.
How to Check Your Answers Effectively

Reviewing your work before submitting it is crucial for catching mistakes and ensuring accuracy. Effective checking helps prevent minor errors from affecting your overall performance. By following a clear and structured approach, you can make sure that each step has been completed correctly and that you haven’t overlooked any important details.
To maximize your chances of identifying mistakes, it’s important to approach the review process systematically. Here are some strategies to consider when double-checking your responses.
Step-by-Step Review Process
One of the best ways to check your work is to follow a step-by-step review process. This ensures that you are not skipping over any part of the problem-solving method and allows you to catch any overlooked errors.
- Start with the simplest: Begin by reviewing the easiest questions first to confirm their correctness quickly.
- Check your calculations: Recalculate any operations or figures that are central to your solution. Verify your logic and ensure that the numbers you’ve written are accurate.
- Look for common errors: Check for misread questions, skipped steps, or calculation mistakes.
Using a Checklist to Verify Your Work
Having a checklist can be a helpful way to ensure you are covering all the necessary points. Below is a table outlining some common aspects to verify during your review.
| Verification Step | What to Look For |
|---|---|
| Read the Problem Carefully | Ensure you understand the question and all its requirements. |
| Recheck Calculations | Verify all operations, including addition, subtraction, multiplication, and division. |
| Look for Consistency | Ensure that the units, decimal places, and formats are consistent throughout your work. |
| Double-Check Your Final Answer | Ensure that the final answer makes sense in the context of the problem. |
By using this methodical approach, you’ll be able to effectively catch mistakes and improve the accuracy of your work before finalizing it.
Real-Life Applications of Chapter 3 Math
The concepts learned in this section are not just theoretical–they have real-world applications that can be observed in everyday life. By understanding these principles, you can approach tasks with more precision and insight, from managing finances to solving everyday problems. These skills are used by professionals across various fields and can help make smarter decisions in daily activities.
Here are some practical ways the topics explored in this section are applied in real life:
Budgeting and Financial Planning
One of the most common uses of these concepts is in personal finance. Whether you’re managing your allowance, planning a family budget, or saving for a big purchase, the ability to calculate and track money is invaluable. Here’s how the knowledge from this section comes into play:
- Calculating Discounts: Understanding how percentages work helps in determining discounts during sales or finding out the total price after a discount.
- Budgeting Expenses: Breaking down a budget involves adding and subtracting amounts in a clear and accurate way.
- Saving for Goals: Setting up savings plans often involves calculating the total amount needed and figuring out how to allocate money efficiently.
Construction and Design Projects

Another area where these concepts are put to practical use is in construction and design. Builders, architects, and engineers often need to use measurements, ratios, and proportions to create structures that are both functional and safe. For instance:
- Measuring Spaces: Understanding dimensions and converting between units is crucial when measuring areas for flooring or furniture placement.
- Designing Scaled Models: Proportions are used when creating scaled models for building projects, ensuring that the model accurately represents the real structure.
These are just a few examples, but the practical applications are endless. By mastering these principles, you can navigate various aspects of life with greater confidence and efficiency.
Improving Test-Taking Confidence
Building confidence before an evaluation can significantly improve your performance. When you feel prepared and assured, it becomes easier to approach each question with clarity and focus. This section provides strategies to boost your confidence and enhance your ability to tackle challenges calmly and efficiently. By following these tips, you can reduce anxiety and perform at your best.
Preparation is Key

The first step in boosting confidence is thorough preparation. When you are well-prepared, you will feel less worried about facing unfamiliar material. Consider the following strategies:
- Practice Regularly: Consistent practice helps reinforce your understanding and make you comfortable with the concepts.
- Break Down Difficult Topics: Identify areas that seem more challenging and focus on breaking them down into smaller, manageable sections.
- Use Review Materials: Go over study guides, notes, and online resources to reinforce your knowledge before any evaluation.
Staying Calm and Focused
It’s not just about what you know, but also how you approach the situation. Developing the right mindset can make a huge difference. Here are some tips for maintaining focus during the actual experience:
- Stay Positive: Remind yourself that you are prepared and capable. Positive self-talk can counter any doubts you may have.
- Read Each Question Carefully: Take your time to understand what is being asked before you begin answering. This will prevent mistakes due to hasty responses.
- Take Deep Breaths: If you feel nervous, pause for a moment to breathe deeply and calm your mind. This can help reduce stress and refocus your attention.
By practicing these strategies, you’ll build a solid foundation of confidence that will help you tackle any challenge with greater ease and poise.