
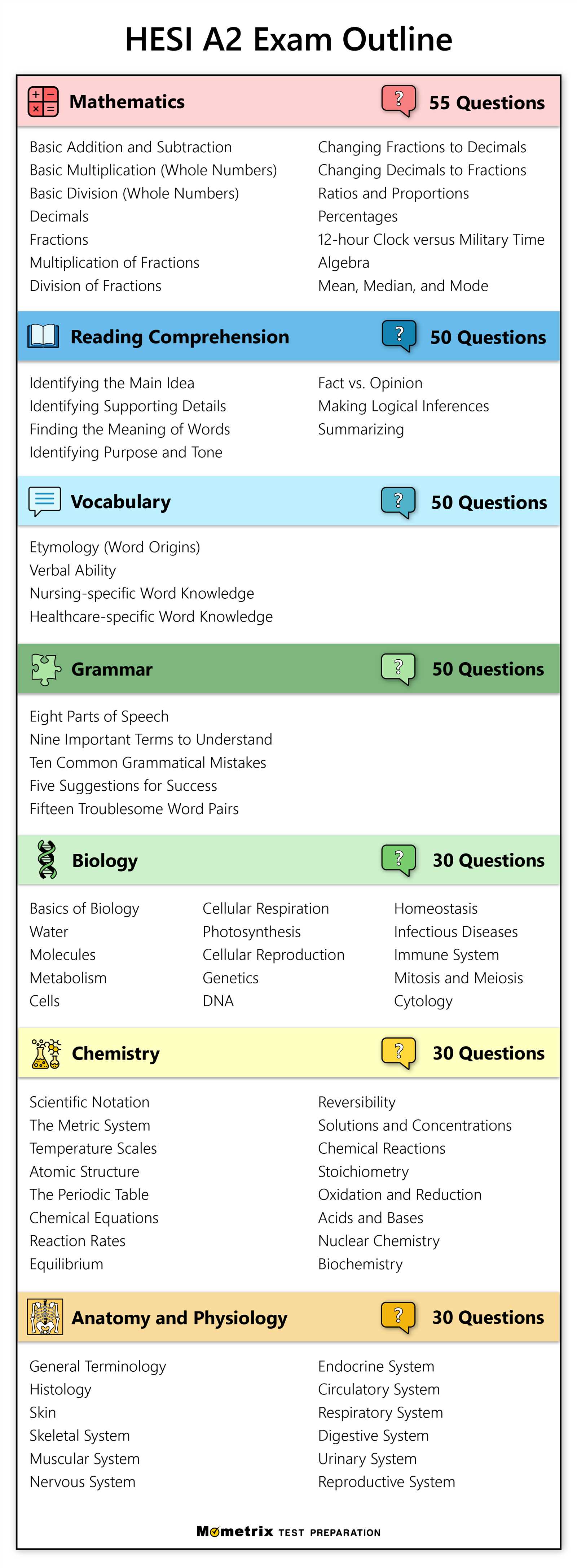
Preparing for any assessment that involves numerical reasoning can be a challenging yet rewarding process. With the right approach and focused study plan, you can significantly improve your ability to solve problems efficiently and accurately. Understanding the key areas that will be tested and regularly reinforcing your skills is crucial for achieving a strong performance.
In this guide, we will explore effective techniques to enhance your readiness for an upcoming test that assesses mathematical and analytical abilities. By breaking down the content into manageable sections, you’ll be able to tackle even the most complex questions with confidence. Emphasizing strategic review and proper preparation can help turn nervousness into focused energy on the day of the assessment.
Effective preparation involves practicing core topics, reviewing fundamental principles, and mastering calculation methods. This approach will not only prepare you for the types of questions you will encounter but also allow you to manage your time effectively during the actual test. Whether you are new to the material or looking to sharpen your skills, a systematic study plan can make all the difference.
Effective Strategies for Success
Achieving strong results in any test involving numerical reasoning requires more than just reviewing content; it demands a well-structured approach to both studying and test-taking. Adopting proven strategies can help you approach questions with clarity and confidence, making the process more manageable and efficient. By incorporating specific techniques into your preparation, you can maximize your potential to perform at your best.
Here are some strategies that can significantly improve your performance:
- Understand the Test Format: Familiarizing yourself with the structure of the assessment will give you an edge. Knowing the types of questions and the time limits allows you to plan your approach accordingly.
- Break Down Complex Problems: When faced with difficult questions, take a moment to break them down into smaller, more manageable parts. This helps you focus on one step at a time, reducing the chance of mistakes.
- Practice Consistently: Regularly working through problems helps solidify your understanding of key concepts and builds muscle memory for solving problems quickly and accurately.
In addition to these techniques, there are other aspects of preparation that can make a significant difference:
- Focus on Time Management: Practice under timed conditions to build your ability to pace yourself. This ensures that you can complete the test within the allotted time.
- Review Past Mistakes: Analyze incorrect answers to understand where you went wrong. This helps to identify patterns and avoid making the same mistakes in the future.
- Stay Calm and Confident: Test anxiety can hinder your ability to think clearly. Practice relaxation techniques and remain confident in your preparation.
By combining these strategies, you can improve both your understanding of the material and your ability to perform under pressure. With consistent effort and focused practice, you’ll be well on your way to success.
How to Manage Time During Testing
Efficient time management is a critical skill for successfully completing assessments that involve solving numerous problems within a limited timeframe. Balancing speed with accuracy ensures that you can answer all questions without feeling rushed or overwhelmed. With proper planning and awareness, you can use the allotted time wisely and avoid common pitfalls.
Understand the Timing Structure
Before starting the test, familiarize yourself with the overall time limit and how it is divided among sections. Knowing how much time to allocate to each question allows you to pace yourself appropriately and ensures you don’t spend too long on any single problem.
To manage time effectively:
- Read Instructions Carefully: Spend the first minute reviewing the test instructions to avoid misunderstandings that could lead to wasted time.
- Prioritize Easy Questions: Begin with problems you find straightforward to build momentum. Mark more complex ones to revisit later if time allows.
Strategies for Staying on Track
Maintaining a steady rhythm throughout the test is essential. Use these strategies to avoid running out of time:
- Set Mini-Deadlines: Divide the total time by the number of questions and aim to stay within that pace. For example, if there are 40 questions in 60 minutes, spend no more than 90 seconds per question.
- Avoid Perfectionism: If a problem is taking too long, make an educated guess and move on. It’s better to attempt all questions than to leave some unanswered.
- Keep Track of Time: Regularly glance at the clock to ensure you’re on schedule. Adjust your speed if you find yourself lagging behind.
By mastering these techniques, you can confidently navigate time constraints and improve your overall performance. Effective time management not only helps you complete the test but also reduces stress, allowing you to focus fully on each question.
Understanding Common Mathematical Concepts
Having a solid grasp of foundational numerical and analytical concepts is essential for tackling assessments that test problem-solving abilities. These core principles often form the basis for many of the questions, so understanding them deeply will improve both accuracy and speed when answering. A clear understanding of these concepts can help you recognize patterns, simplify problems, and avoid common errors during the test.
Key concepts that are frequently tested include:
- Fractions and Decimals: The ability to work with fractions, decimals, and their conversions is fundamental. Practice simplifying fractions and performing basic operations with both fractions and decimals to build confidence.
- Percentages: Understanding percentages is crucial for solving problems involving increases, decreases, and ratios. Mastering the percentage formula and knowing how to apply it to various scenarios is essential.
- Basic Algebra: Knowledge of variables, equations, and expressions is a must. You should be comfortable solving for unknowns and simplifying algebraic expressions.
Additionally, grasping geometric concepts such as area, volume, and perimeter, as well as interpreting data from graphs, tables, and charts, can significantly impact your test performance. The more familiar you become with these topics, the quicker and more accurately you’ll be able to approach each problem.
Quick Ways to Improve Calculation Speed
Enhancing your ability to perform calculations quickly is a crucial skill when facing assessments with time constraints. Speed is not just about knowing the correct answers; it’s about efficiently applying your knowledge and minimizing the time spent on each question. With practice and a few targeted techniques, you can significantly improve your calculation speed and boost your overall performance.
Here are some methods to help you speed up your calculations:
- Master Mental Math: Develop the habit of performing basic operations, like addition, subtraction, multiplication, and division, without relying on a calculator. The more you practice mentally, the faster you’ll become.
- Use Estimation: For complex problems, start by estimating the answer. This can help you quickly eliminate obviously incorrect options and focus on the most reasonable ones.
- Memorize Key Formulas: Having key mathematical formulas at your fingertips can save you time. This includes things like the area of geometric shapes or percentage calculations, which are common on many assessments.
In addition to these techniques, using shortcuts and simplifying problems can also lead to faster calculations:
- Break Down Complex Problems: If a problem seems complicated, break it down into smaller steps. Simplifying the process makes it easier to handle quickly and accurately.
- Practice with Timed Drills: Set time limits when working through practice problems. This will help you develop a sense of urgency and improve your speed without sacrificing accuracy.
By integrating these strategies into your preparation routine, you’ll not only increase your calculation speed but also gain more confidence in handling problems under pressure.
Breaking Down Word Problems Step by Step
Word problems often seem overwhelming at first glance, especially when they involve multiple pieces of information. However, approaching them systematically can make even the most complex questions more manageable. By breaking down each problem into smaller, more understandable steps, you can easily identify the key components and find the solution efficiently.
Step 1: Read the Problem Carefully
The first step in solving a word problem is to thoroughly read the question. Highlight or underline important details such as numbers, units, and keywords that indicate the operations you need to perform. Identifying these elements early on will help you focus on the right approach.
Step 2: Identify What Is Being Asked
Before jumping into calculations, determine exactly what the problem is asking for. Are you solving for a total, a difference, or a ratio? Knowing what to find helps you decide the right method to use.
| Example Problem | Key Information | What to Find |
|---|---|---|
| A store sells 3 pens for $5. How much would 9 pens cost? | 3 pens = $5, 9 pens | Total cost for 9 pens |
| A car travels 60 miles in 1 hour. How far will it travel in 3 hours? | 60 miles per hour, 3 hours | Total distance in 3 hours |
By following this structured approach, you can break down any word problem into a series of logical steps, ensuring you don’t miss any crucial details while solving. This technique not only saves time but also improves accuracy in problem-solving.
Importance of Memorizing Formulas
Memorizing key formulas is an essential part of preparation for any assessment involving quantitative reasoning. When you have the most important equations and principles committed to memory, you can solve problems more quickly and efficiently, without needing to stop and reference materials. This saves valuable time and helps you maintain focus throughout the test.
Speed and Efficiency
Having formulas readily available in your mind allows you to spend more time solving the problem rather than looking up equations. Whether it’s for calculating areas, volumes, or percentages, the ability to recall the necessary formulas instantly can drastically improve your speed during the assessment.
Building Confidence
Knowing that you have a solid foundation of formulas to draw from can boost your confidence when facing challenging questions. You’ll be able to tackle problems with greater assurance, knowing you have the tools to approach them effectively.
In addition to memorizing formulas, understanding how and when to apply them is equally important. With consistent practice, these equations will become second nature, making complex problems easier to navigate.
Practical Tips for Practicing Equations
Consistent practice is key to mastering any set of equations. The more you work with them, the more intuitive and efficient you’ll become at solving them. While it’s important to understand the theory behind each equation, applying them through hands-on exercises is what truly solidifies your knowledge and skills.
Start with Simple Problems
When beginning your practice, start with simpler problems to build your confidence. Gradually increase the complexity of the equations as you become more comfortable. This incremental approach helps you establish a solid foundation before tackling more challenging questions.
Use a Variety of Resources
Don’t rely on just one source for your practice. Use a mix of textbooks, online resources, and practice worksheets to expose yourself to different types of equations. This variety ensures you encounter a wide range of problems, helping you become adaptable and prepared for anything that may appear on the test.
Additionally, regularly review and repeat problems you’ve already solved. Repetition reinforces your understanding and speeds up your ability to identify the best solution methods quickly.
Mastering Fractions and Decimals Easily
Fractions and decimals are fundamental concepts that often appear in various problem types. Understanding how to work with them efficiently is crucial for achieving accuracy and speed when solving related questions. With the right strategies and consistent practice, you can simplify these concepts and approach them with confidence.
Here are some key tips to help you master fractions and decimals:
- Convert Between Fractions and Decimals: Learning to switch between fractions and decimals can save time and make calculations easier. For example, 1/4 is equal to 0.25, and 3/5 equals 0.6. Understanding these conversions ensures you can handle any type of problem that involves either form.
- Simplify Fractions: Always simplify fractions to their lowest terms. For instance, 4/8 can be reduced to 1/2. This not only makes calculations easier but also helps you avoid errors when performing operations.
- Work with Decimal Places: When dealing with decimals, make sure to align decimal points correctly in addition or subtraction problems. For multiplication and division, remember that the number of decimal places in the final answer depends on the numbers you are working with.
By practicing these steps regularly, you’ll improve both your understanding and speed in handling fractions and decimals. With enough repetition, these concepts will become second nature, allowing you to tackle them quickly and efficiently during assessments.
Handling Percentages in Complex Questions
Working with percentages in more complicated problems can seem intimidating at first, but breaking down the steps into manageable parts can help simplify the process. By understanding the core principles behind percentage calculations and applying effective strategies, you can tackle even the most challenging percentage-related questions with confidence.
Step 1: Understand the Problem
Before diving into calculations, carefully read through the problem and identify key information. Look for words or phrases that indicate percentage changes, such as “increase,” “decrease,” “of,” or “what percent.” Knowing what you’re being asked to find will guide you through the solution process.
Step 2: Use the Percentage Formula
The general formula for calculating percentages is:
- Percentage = (Part / Whole) * 100
Apply this formula to find the desired percentage. For example, if the problem asks for 25% of 200, multiply 200 by 0.25 to get 50. For more complex questions, break the steps into smaller calculations to maintain accuracy.
Step 3: Handling Percentage Increase or Decrease

When dealing with percentage increases or decreases, follow these steps:
- Increase: Add the percentage increase to the original number. For example, a 20% increase on 50 would be 50 + (50 * 0.20) = 60.
- Decrease: Subtract the percentage decrease from the original number. For example, a 30% decrease on 80 would be 80 – (80 * 0.30) = 56.
By practicing these steps and understanding the core principles of percentage calculations, you’ll be able to handle even the most complex questions with ease and precision.
Developing Logical Reasoning for Problem Solving
Effective problem-solving relies heavily on strong logical reasoning skills. Being able to break down complex scenarios into smaller, more manageable parts is essential for identifying patterns, finding solutions, and eliminating potential errors. The key to mastering problem-solving is developing a systematic approach that you can apply consistently, no matter the complexity of the question.
Step-by-Step Approach
When faced with a problem, it is helpful to approach it step-by-step. This method allows you to systematically examine each component of the problem and build your solution incrementally. Here are some strategies:
- Identify the problem: Understand exactly what is being asked. Clarifying the question ensures you’re focusing on the right objective.
- Break it down: Divide the problem into smaller, more manageable parts. This makes it easier to identify the most effective solution strategy.
- Evaluate the steps: Consider the potential methods of solving each part. Choose the most efficient approach to ensure you solve the problem accurately.
Using Deductive Reasoning
Deductive reasoning is an important tool when approaching complex problems. This method involves making logical inferences based on known facts or premises. Start by identifying what you already know and use this information to eliminate possibilities and narrow down the solution. By practicing deductive reasoning, you can sharpen your ability to make informed decisions based on solid logic.
By honing your logical reasoning skills and applying a structured approach, you’ll improve your ability to solve problems quickly and accurately, even under pressure.
Using Online Resources for Study Preparation
In today’s digital age, online resources offer an incredible advantage when preparing for any type of assessment. From interactive tutorials to practice sets, the internet provides a vast array of tools that can help reinforce concepts, test your skills, and track your progress. Leveraging these resources effectively can enhance your study sessions and improve overall performance.
Here are some key online tools to help you prepare:
- Interactive Practice Tests: Many websites offer simulated tests that mirror the format of real assessments. These practice tests provide an excellent opportunity to familiarize yourself with the structure and timing, ensuring you’re well-prepared for the actual experience.
- Video Tutorials: Platforms like YouTube and educational websites feature instructional videos that break down complex topics. Watching these can provide a different perspective and make challenging concepts easier to grasp.
- Study Apps: Mobile apps designed for exam preparation allow you to study on the go. These apps often include features like flashcards, quizzes, and progress tracking, helping you maximize your study time efficiently.
Using these online tools in conjunction with traditional study methods ensures that you’re well-rounded in your approach. By taking advantage of what the internet offers, you can stay on track and cover all necessary areas before your assessment.
Benefits of Taking Practice Exams Regularly
Engaging in regular assessments is a powerful strategy to enhance your preparation. By simulating the actual testing environment, you gain insights into your strengths and areas for improvement. These mock assessments help in managing time, identifying patterns in question types, and refining your approach to problem-solving.
Here are several key benefits of incorporating these assessments into your routine:
| Benefit | Description |
|---|---|
| Builds Confidence | Frequent testing allows you to become familiar with the content and format, boosting your confidence for the actual assessment. |
| Improves Time Management | Regular assessments help you get used to the time constraints, allowing you to pace yourself effectively during the actual test. |
| Highlights Weak Areas | By analyzing your performance, you can pinpoint areas where you need more attention and focus your studies accordingly. |
| Tracks Progress | By taking mock assessments regularly, you can track how much you’ve improved, which helps in staying motivated. |
Integrating regular assessments into your study routine helps create a more structured and focused preparation plan, ensuring you’re as ready as possible when it matters most.
Common Mistakes Students Make in Arithmetic
When working with numerical problems, students often encounter obstacles that hinder their ability to perform accurately. These errors can range from simple miscalculations to more complex misunderstandings of the concepts involved. Identifying and addressing these mistakes is key to improving problem-solving skills and achieving better results.
Here are some of the most frequent errors students make in basic numerical tasks:
- Overlooking Order of Operations: Failing to follow the correct sequence of operations can lead to incorrect results. It’s essential to remember the order: parentheses, exponents, multiplication and division (from left to right), and addition and subtraction (from left to right).
- Misplacing Decimal Points: Decimal point errors are common, especially when multiplying or dividing decimals. A small shift can drastically change the answer.
- Incorrectly Simplifying Fractions: When reducing fractions, students sometimes fail to divide by the greatest common divisor, leading to inaccurate results.
- Forgetting to Carry or Borrow in Addition/Subtraction: In operations involving larger numbers, students may forget to carry over or borrow, which can cause cascading mistakes in the final answer.
- Rushing Through Word Problems: When solving word problems, students often skip important details or rush through the process, missing crucial steps or making assumptions that aren’t supported by the data.
By becoming aware of these common errors and taking steps to avoid them, students can significantly improve their accuracy and efficiency in solving numerical problems.
How to Stay Calm Under Pressure
Maintaining composure during high-stakes situations can make a significant difference in performance. When faced with challenging tasks, staying calm allows you to think more clearly, make better decisions, and avoid unnecessary mistakes. Developing strategies to manage stress and anxiety effectively is essential for success.
Here are several strategies to help you stay calm when pressure mounts:
- Practice Deep Breathing: Deep, slow breaths can activate the body’s relaxation response. Taking a moment to breathe deeply can lower your heart rate and clear your mind.
- Break Tasks into Smaller Steps: Instead of feeling overwhelmed by the entire task, break it down into smaller, manageable steps. Focus on one part at a time to prevent feeling swamped.
- Stay Positive: Focus on positive self-talk. Remind yourself of your preparation and abilities. Confidence in your skills can help reduce anxiety.
- Visualize Success: Imagine yourself successfully completing the task. Visualization helps reduce stress and reinforces a positive mindset.
- Control Your Environment: If possible, create a calm, quiet space for yourself. Minimizing distractions can help maintain focus and reduce external stressors.
- Take Breaks: If you begin to feel overwhelmed, step back for a moment. A short break can refresh your mind and improve concentration when you return to the task.
By adopting these techniques, you can reduce stress, improve focus, and enhance your overall performance under pressure.
Creating a Personalized Study Schedule
Developing an effective study routine is crucial for maximizing learning and retaining information. A well-structured schedule helps you stay organized, manage time efficiently, and ensures you cover all necessary topics. Personalizing your study plan based on your strengths, weaknesses, and available time can make a significant difference in your preparation process.
Assess Your Available Time
Before creating your schedule, evaluate how much time you can realistically dedicate to studying each day. Consider your other commitments, such as work or personal activities, and allocate specific time slots for focused study. Prioritize your weaker areas, but ensure you give attention to all topics, even if they come easier to you.
Set Realistic Goals
Break down your study material into manageable sections. Set specific, achievable goals for each study session. For example, instead of saying “study all week,” aim to complete certain chapters or practice a particular set of problems within each designated time frame. This helps track your progress and keeps you motivated.
By following these steps and customizing your study plan to suit your personal needs, you can maintain consistency and enhance the effectiveness of your preparation.
Importance of Reviewing Practice Test Answers
Reviewing your answers after completing mock assessments is a vital part of the learning process. This step allows you to identify your strengths and weaknesses, learn from your mistakes, and improve your understanding of the material. Simply completing a test without reflecting on the results misses the opportunity to reinforce learning and gain insight into areas that need more focus.
Identify Areas of Improvement
By carefully analyzing your responses, you can pinpoint which topics or concepts caused confusion. Reviewing incorrect answers helps you understand why a particular approach failed and how to correct it moving forward. This reflective practice ensures you don’t repeat the same errors in future challenges.
Track Progress Over Time
Consistently reviewing your answers allows you to track improvements as you progress through your preparation. This not only boosts your confidence but also gives you a clear picture of where additional study is needed. It is crucial for staying on track and adjusting your study plan as required.
| Area of Focus | Improvement Actions |
|---|---|
| Algebra | Review fundamental equations and practice problem-solving techniques. |
| Word Problems | Break down each problem into smaller, manageable steps for clarity. |
| Fractions | Review conversion methods and practice with a variety of fraction problems. |
Incorporating this habit into your study routine helps reinforce key concepts and ensures you’re prepared for future assessments.
Key Focus Areas for Exam Preparation
When preparing for any assessment, it’s essential to concentrate on areas that will have the most significant impact on your performance. Focusing your efforts on key topics and building a strong foundation in those areas will help you maximize your chances of success. Understanding where you need the most improvement allows for efficient and effective use of study time.
One of the most crucial aspects of preparation is identifying the core concepts and skills that are most likely to be tested. These areas often serve as the foundation for more complex questions and require a deep understanding. Strengthening your knowledge in these focus areas not only improves your confidence but also enhances your problem-solving abilities.
It’s equally important to balance your time across a variety of topics. While some subjects may need more attention, others can be reinforced through quick reviews. This balanced approach ensures that you’re well-prepared for every section of the assessment without feeling overwhelmed.
Motivational Tips for Consistent Study Progress
Maintaining steady momentum in your learning journey is essential for achieving success. Staying motivated and focused helps you tackle each study session with a sense of purpose and determination. By developing positive habits and staying committed to your goals, you can make meaningful progress and avoid falling behind.
Set Clear and Achievable Goals

One of the best ways to keep yourself motivated is by setting clear and achievable targets. Breaking down your overall goals into smaller, manageable steps gives you a clear path to follow and creates a sense of accomplishment as you reach each milestone. This approach keeps you engaged and encourages continuous progress.
Reward Yourself for Milestones
Celebrating small victories along the way can be a powerful motivator. Whether it’s taking a short break, enjoying a favorite snack, or indulging in an activity you love, rewarding yourself after achieving study goals reinforces positive behavior. These rewards serve as reminders of your progress and keep you energized for the next challenge.
Consistency is the key to long-term success. Incorporating study into your daily routine helps build momentum, and even small, consistent efforts can lead to significant improvements over time. Stay dedicated, trust the process, and remember that each study session is one step closer to reaching your desired outcome.