Achieving success in standardized assessments requires not only understanding key concepts but also being able to apply them efficiently under timed conditions. The numerical reasoning portion of these exams is often one of the most challenging yet rewarding areas to tackle. By focusing on specific strategies and familiarizing yourself with common problem types, you can boost your overall performance and feel confident during the exam.
While preparation is essential, knowing how to approach questions strategically can make a significant difference. From solving algebraic expressions to navigating geometric problems, each section presents unique challenges. With targeted practice and a deep understanding of fundamental techniques, you can enhance your ability to solve problems quickly and accurately.
Focusing on core skills and understanding question formats are key to improving efficiency. Whether dealing with complex equations or interpreting word-based scenarios, knowing how to break down a problem is crucial. Consistent practice with the right resources will not only help you become familiar with question types but also train you to manage your time effectively.
By mastering the skills needed for tackling this portion, you’ll have a clear advantage when it comes to test day. With the right mindset and preparation, success in this challenging segment becomes not just possible, but highly achievable.
Understanding the Mathematics Section

The numerical reasoning part of standardized exams evaluates your ability to solve problems efficiently under time constraints. This segment covers a wide range of topics, from basic arithmetic to more advanced concepts like algebra and geometry. A strong grasp of core principles and the ability to approach questions strategically is essential to succeeding in this section.
The questions are designed to assess not only your knowledge of mathematical concepts but also how well you can apply these concepts to solve real-world problems. With the right preparation, you can improve your ability to navigate these questions quickly and accurately, leading to better results.
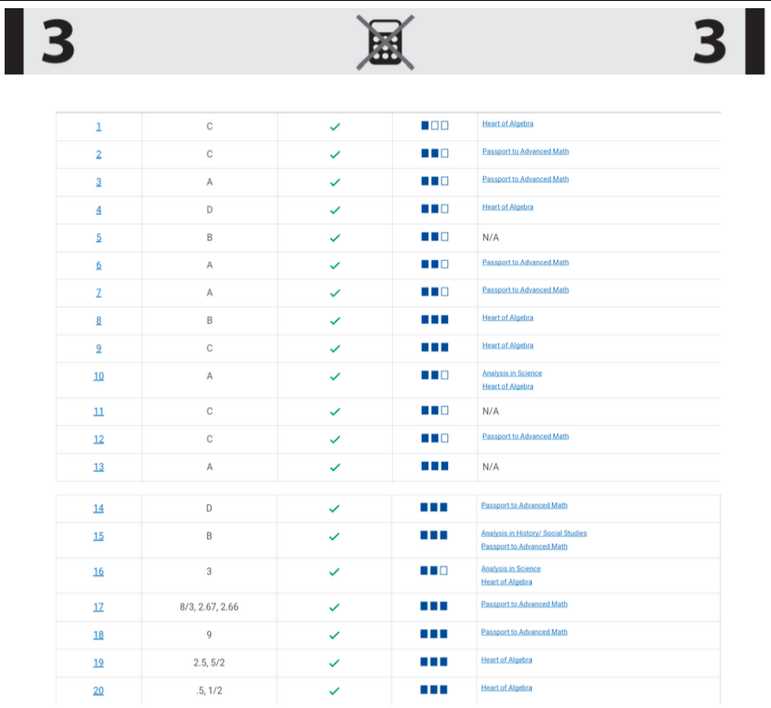
| Topic | Question Types | Key Skills |
|---|---|---|
| Algebra | Linear equations, inequalities, systems of equations | Simplifying expressions, solving for unknowns |
| Geometry | Angles, circles, area, perimeter | Understanding shapes and spatial reasoning |
| Trigonometry | Sine, cosine, tangent, right triangles | Identifying functions, solving for unknown sides |
| Word Problems | Real-life scenarios requiring calculations | Translating words into mathematical equations |
Familiarity with the structure and variety of problems is key. By practicing different types of questions and refining your problem-solving skills, you’ll be better prepared for the challenges of this section. Understanding how to allocate time for each question is just as important as knowing the material itself.
Overview of Mathematics Test 2
This section is designed to evaluate your proficiency in solving a variety of numerical and logical problems. It consists of several different question types, each focusing on specific skills and concepts. These problems are crafted to assess how well you can apply your knowledge to both straightforward calculations and more complex scenarios.
The format of the section requires both speed and accuracy. Understanding the types of questions you’ll face and the areas that are emphasized can give you a distinct advantage. The main goal is to assess your ability to reason mathematically, solve problems efficiently, and interpret information correctly.
- Basic Algebra – Expect to solve equations, inequalities, and work with polynomials.
- Geometry – Questions may involve angles, areas, perimeters, and properties of shapes.
- Advanced Functions – Problems on manipulating functions and understanding graphs are common.
- Word Problems – Real-world scenarios where you’ll need to translate textual information into mathematical expressions.
- Data Interpretation – Graphs, tables, and charts will be used to test your ability to interpret and draw conclusions from data.
While each question tests a different aspect of your mathematical understanding, the key to success lies in your ability to apply learned strategies quickly. Regular practice with these different problem types will help you become familiar with the format and enhance your problem-solving speed.
Key Strategies for ACT Math Success
Succeeding in the numerical reasoning section of any standardized exam requires more than just knowledge of mathematical concepts; it demands efficient problem-solving skills and the ability to apply strategies under time pressure. The most successful candidates are those who not only understand the material but also know how to approach questions strategically, ensuring they can maximize their time and accuracy.
One of the most effective strategies is to familiarize yourself with the most common types of questions. This allows you to quickly identify the type of problem you’re dealing with and apply the most appropriate method to solve it. Additionally, understanding the structure of the section helps you allocate your time more effectively, ensuring you have enough to tackle the more challenging questions.
Another key approach is to practice mental math whenever possible. Being able to solve simple calculations quickly in your head can save valuable time during the exam, allowing you to focus on more complex problems. Furthermore, practicing estimation techniques can help you eliminate clearly incorrect answers, narrowing down your choices and increasing your chances of selecting the right one.
Lastly, it’s important to stay calm and avoid getting stuck on any one question. If a problem seems too difficult, it’s often best to move on and return to it later if time permits. This prevents you from wasting valuable minutes and helps maintain your focus throughout the section.
How to Approach Multiple-Choice Questions
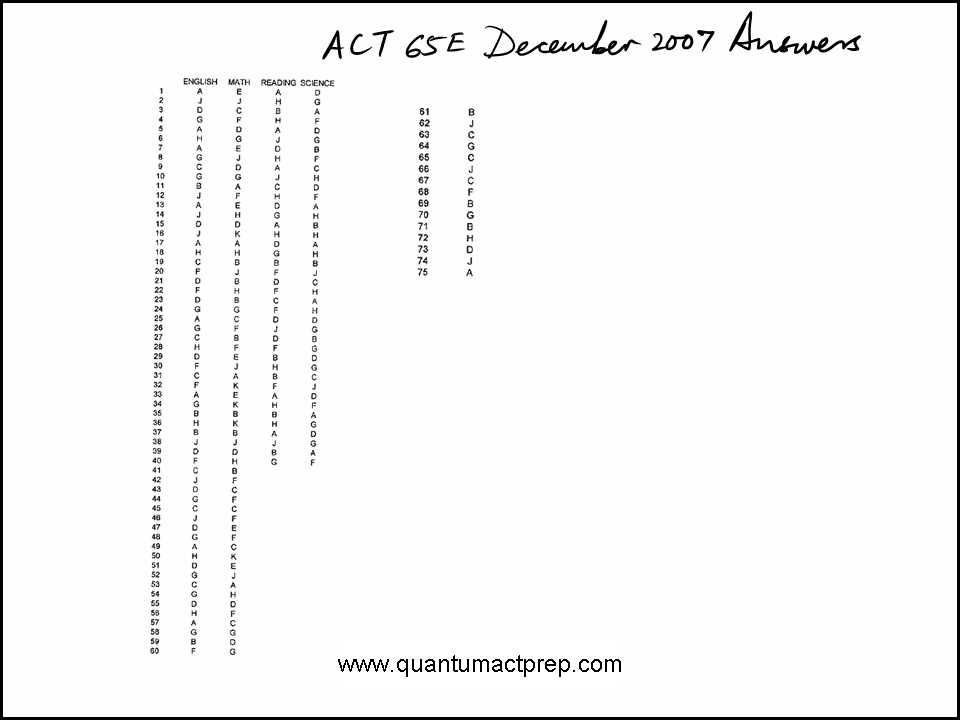
Multiple-choice questions are a common feature in standardized assessments and can often feel overwhelming due to the variety of possible answers. However, with the right strategies, you can approach them more confidently and efficiently. The key is to understand how to evaluate the options critically and use logical reasoning to eliminate incorrect choices.
Start by carefully reading the question to ensure you understand what is being asked. Often, test-makers include extra details designed to distract you. Focus on the core of the question and identify exactly what information is needed to solve the problem. This helps prevent misinterpretations and sets you up for success.
Once you have a clear understanding of the question, look at all of the answer choices before attempting to solve the problem. Even if you know the solution immediately, it’s often helpful to review all the options to see if there’s a subtle difference or trick that could make one of them stand out. If you’re unsure of the answer, use the process of elimination to rule out obviously incorrect choices.
It’s also important to stay mindful of time. While careful consideration is necessary, avoid overthinking. If you’re stuck, move on and return to the question later if possible. This way, you don’t lose valuable time on a single question.
By practicing these strategies, you can approach multiple-choice problems with more confidence, increasing your chances of selecting the correct answer efficiently.
Mastering Algebra for ACT Test
Algebra forms a significant part of any standardized exam, and mastering it is crucial to achieving a high score. This section focuses on your ability to manipulate equations, solve for unknowns, and understand mathematical relationships. By refining your skills in these areas, you’ll be well-equipped to tackle a wide range of problems efficiently.
To excel in algebra, it’s essential to practice the following key concepts:
- Solving Linear Equations: Be comfortable solving single-variable equations and inequalities.
- Factoring Expressions: Learn how to factor quadratic and higher-order polynomials to simplify problems.
- Working with Exponents: Understand the rules of exponents and apply them to simplify expressions.
- Systems of Equations: Practice solving systems of equations using substitution and elimination methods.
- Manipulating Fractions: Master operations with fractions, including addition, subtraction, multiplication, and division.
Alongside these core concepts, it’s also important to be familiar with word problems that require algebraic reasoning. In such problems, translating the text into equations and solving them is a key skill. Breaking down the problem into smaller, more manageable steps will help you stay organized and improve your chances of finding the correct solution.
With consistent practice and a solid understanding of algebraic principles, you’ll increase your ability to handle algebra-related questions quickly and accurately, boosting your overall performance in the exam.
Top Tips for Solving Equations
Solving equations is a fundamental skill in any standardized exam, and mastering it can significantly enhance your performance. The key to success lies in developing a systematic approach, which ensures that you can quickly identify the best method for solving a variety of problems. By focusing on clarity and organization, you can solve equations more efficiently and with greater accuracy.
1. Simplify the Equation First

Before attempting to solve any equation, always start by simplifying both sides as much as possible. This includes removing parentheses, combining like terms, and reducing fractions. A simpler equation is easier to work with and increases the likelihood of finding the correct solution.
2. Use Logical Steps
Once simplified, apply the appropriate algebraic techniques based on the equation’s structure. Whether you need to isolate the variable, apply the distributive property, or solve for multiple unknowns, ensure that each step logically follows the previous one. Keeping track of each action will help you avoid mistakes and stay on the right track.
| Equation Type | Key Strategy | Example |
|---|---|---|
| Linear Equations | Isolate the variable using inverse operations. | 2x + 5 = 15, subtract 5, then divide by 2. |
| Quadratic Equations | Use factoring, the quadratic formula, or completing the square. | x² – 5x + 6 = 0, factor as (x – 2)(x – 3). |
| Systems of Equations | Use substitution or elimination methods to solve. | 2x + y = 8, x – y = 4, solve for x and y. |
By focusing on these strategies and practicing regularly, you will build confidence in solving equations quickly and accurately. Clear thinking and methodical steps are the keys to mastering this skill, helping you succeed in the numerical reasoning section of any exam.
Geometry Concepts You Must Know
Geometry plays a significant role in problem-solving scenarios that require a clear understanding of shapes, sizes, and spatial relationships. To excel in this section, it’s important to familiarize yourself with the essential concepts and formulas that are commonly tested. Whether you are dealing with angles, areas, or volumes, having a solid grasp of geometry fundamentals is key to quickly and accurately answering questions.
Here are some crucial topics you should master to tackle geometry problems effectively:
- Properties of Triangles: Be comfortable with different types of triangles (equilateral, isosceles, scalene) and understand important properties like the Pythagorean theorem.
- Circles: Know the relationships between radius, diameter, circumference, and area. Familiarize yourself with important theorems, such as those involving inscribed angles.
- Angles: Understand the various angle types–acute, obtuse, right–and be able to work with complementary, supplementary, and vertical angles.
- Polygons: Study the properties of different polygons, including quadrilaterals, pentagons, hexagons, and more. Know how to calculate their interior and exterior angles.
- Volume and Surface Area: Be able to calculate the volume and surface area of common 3D shapes, such as cylinders, spheres, and pyramids.
Familiarizing yourself with these concepts will help you break down problems more effectively. By practicing the application of these ideas in various scenarios, you’ll become more confident in your ability to solve geometry-related questions under time constraints.
Solving Geometry Problems Efficiently
When faced with spatial reasoning problems, efficiency is crucial to achieving success. The ability to quickly identify the most relevant information, apply the right formula, and eliminate unnecessary steps can significantly improve your problem-solving speed and accuracy. Mastering this approach not only helps save valuable time but also reduces the risk of errors.
Here are some strategies to solve geometry problems more efficiently:
- Understand Key Formulas: Familiarize yourself with essential formulas, such as those for area, perimeter, volume, and surface area. Having these formulas at your fingertips allows you to tackle problems without spending unnecessary time on calculations.
- Visualize the Problem: Draw diagrams whenever possible. A clear sketch can reveal hidden relationships between shapes and make it easier to spot patterns or apply geometric principles.
- Look for Symmetry: Identify symmetry in figures, as it can simplify the problem. Symmetrical shapes often allow you to divide a problem into smaller, more manageable sections.
- Break Complex Problems into Parts: For complex shapes or multi-step problems, divide the problem into smaller sections. Solve each part step by step before combining the results to get the final answer.
- Eliminate Unnecessary Information: Not all details in a problem are essential. Identify key pieces of information, and disregard anything that doesn’t contribute directly to solving the question.
By implementing these techniques and practicing regularly, you’ll develop the skills needed to approach geometry problems with confidence, saving time and increasing accuracy in your solutions.
Advanced Topics in ACT Math
For those looking to excel in the more challenging portions of the exam, it is essential to grasp advanced mathematical concepts. These topics go beyond basic problem-solving and require a deeper understanding of algebraic structures, complex functions, and higher-level geometry. Familiarity with these areas can set you apart, enabling you to tackle even the most difficult questions with confidence.
1. Complex Functions and Graphs
Understanding the properties of advanced functions–such as quadratic, exponential, and logarithmic functions–is vital for solving higher-level problems. In addition to recognizing the standard form of these functions, you should be comfortable with graphing them and analyzing key features like intercepts, asymptotes, and behavior at infinity.
2. Advanced Probability and Statistics
Probability and statistics extend far beyond basic concepts of mean, median, and mode. You’ll need to be adept at calculating combinations and permutations, understanding distributions, and applying concepts like expected value and standard deviation. Additionally, interpreting data presented in various forms (tables, graphs, and charts) is essential for success in this area.
Mastering these advanced topics requires not only memorization of key concepts but also practice in applying them to complex problems. A strong foundation in these areas can help you navigate through the most challenging parts of the exam, increasing both speed and accuracy.
Working with Functions and Graphs

In many mathematical problems, working with functions and their graphical representations is essential. Understanding the relationship between equations and graphs can simplify complex problems and provide quick insights into the behavior of different functions. By mastering these concepts, you can easily analyze and solve questions involving rates of change, intercepts, and other critical features of graphs.
Understanding Function Basics
Before diving into specific types of functions, it’s important to understand the basic principles that govern them. Functions describe the relationship between two variables, typically written as f(x). Knowing how to read and manipulate these relationships allows for easier graph interpretation and problem-solving.
- Domain and Range: Know how to identify the set of possible input values (domain) and output values (range) for any given function.
- Intercepts: Be able to find where a function crosses the x-axis (x-intercepts) and y-axis (y-intercepts), which is often critical for solving problems.
- Inverses: Understand how to find the inverse of a function and the relationship between a function and its inverse on a graph.
Graphing Techniques
Graphing functions is a key skill for solving complex equations. A solid grasp of how to sketch various types of graphs quickly can save valuable time in problem-solving scenarios.
- Linear Functions: Straightforward to graph, these functions have constant slopes and form straight lines. Practice identifying the slope and y-intercept to graph them accurately.
- Quadratic Functions: These functions form parabolas. Know how to identify key features like the vertex, axis of symmetry, and direction of opening (upward or downward).
- Exponential Functions: Exponential graphs grow or decay rapidly. Learn how to recognize the asymptotes and behavior of these curves.
Mastering the art of working with functions and graphs enhances your ability to quickly analyze and interpret problems, enabling you to approach even the most complex equations with confidence.
Trigonometry on the ACT
Trigonometric concepts are a key component of the exam, covering a range of topics that require a strong understanding of angles, ratios, and functions. These problems often challenge students to apply their knowledge of sine, cosine, and tangent to solve real-world and abstract problems. Knowing how to efficiently work with these concepts will significantly improve performance and reduce time spent on tricky questions.
Here are some key areas of focus when preparing for trigonometry-related questions:
- Understanding Trigonometric Ratios: The fundamental ratios–sine, cosine, and tangent–are essential for solving problems involving right triangles. Be familiar with the definitions of these ratios and how to apply them in various scenarios.
- Unit Circle: Familiarity with the unit circle and its key values can save time during the exam. Knowing the sine and cosine values for common angles (30°, 45°, 60°, etc.) is especially helpful.
- Angles and Their Measures: You must be comfortable working with both degrees and radians. Knowing how to convert between the two and understanding their application in trigonometric functions is important.
- Graphs of Trigonometric Functions: Understanding how sine, cosine, and tangent functions behave on a graph–such as their period, amplitude, and shifts–is crucial for solving more complex problems.
- Solving Trigonometric Equations: The ability to solve equations that involve trigonometric functions is vital. This includes using inverse functions and manipulating equations to isolate the unknown variable.
By practicing these concepts and becoming familiar with how trigonometric functions interact with other areas of mathematics, you’ll be better prepared to approach these types of questions confidently and efficiently.
Key Trigonometric Identities to Remember
In any exam that involves trigonometry, understanding and memorizing essential identities is crucial for solving problems efficiently. These identities serve as fundamental tools for manipulating trigonometric functions and simplifying equations. By mastering the most important identities, you can solve complex problems quickly and with confidence.
Here are some of the key trigonometric identities you should be familiar with:
- Pythagorean Identity: This is one of the most important identities in trigonometry. It relates the sine and cosine of an angle in a right triangle:
- Reciprocal Identities: These identities express the reciprocal relationships between the basic trigonometric functions:
- csc(θ) = 1/sin(θ)
- sec(θ) = 1/cos(θ)
- cot(θ) = 1/tan(θ)
- Quotient Identities: These identities express the relationships between sine, cosine, and tangent:
- tan(θ) = sin(θ)/cos(θ)
- cot(θ) = cos(θ)/sin(θ)
- Angle Sum and Difference Identities: These identities are useful for finding the sine, cosine, or tangent of a sum or difference of two angles:
- sin(A ± B) = sin(A)cos(B) ± cos(A)sin(B)
- cos(A ± B) = cos(A)cos(B) ∓ sin(A)sin(B)
- tan(A ± B) = (tan(A) ± tan(B)) / (1 ∓ tan(A)tan(B))
sin²(θ) + cos²(θ) = 1
Familiarity with these identities will help you simplify complex expressions, solve equations, and analyze graphs more effectively. Practicing their use in various problems will make them second nature during the exam.
Preparing for Word Problems

Word problems often present a challenge as they require you to translate written information into mathematical operations. These problems test your ability to analyze a situation, extract relevant data, and apply the right methods to find a solution. Developing strategies for tackling word problems can greatly improve your efficiency and accuracy during the exam.
Here are some essential strategies to help you approach word problems effectively:
- Read Carefully: Always read the problem at least twice to understand what is being asked. Focus on the key details and numbers that will guide your solution.
- Identify the Variables: Break the problem into components by identifying what each variable represents. Assign a variable to each unknown quantity.
- Write Down Known Information: Organize the information provided in the problem. This will help you see patterns or relationships between the variables and avoid overlooking important details.
- Choose the Right Strategy: Depending on the problem, decide whether to use algebra, geometry, or another method. Ensure that the approach you select aligns with the data you’ve identified.
- Set Up an Equation: Translate the problem into a mathematical expression or equation that reflects the relationships between the variables. This is often the most crucial step in solving word problems.
- Check Your Work: After solving, take the time to review your solution. Ensure that your answer makes sense in the context of the problem, and double-check your calculations for any errors.
By practicing these strategies, you will be better prepared to handle word problems with confidence, reducing the likelihood of making mistakes under time pressure.
Breaking Down Complex Word Problems
Complex word problems often seem overwhelming at first glance due to the amount of information provided and the multiple steps required to find the solution. The key to solving these problems lies in breaking them down into smaller, manageable parts. By systematically analyzing each component of the problem, you can tackle it more confidently and efficiently.
Here are some effective strategies to approach intricate problems:
Step-by-Step Breakdown
Instead of trying to solve the problem all at once, approach it step by step. Identify the following components:
- What is the question? Determine exactly what is being asked. Highlight or underline the question in the problem to focus on the goal.
- What information is given? Organize the details provided, including numbers, relationships, and any additional context that might help in finding the solution.
- What do you need to find? Identify the unknowns and determine how they relate to the known information. Assign variables to represent these unknowns if necessary.
Using a Table to Organize Information
One effective way to visualize the problem is by organizing the data into a table. This can help you make connections between different pieces of information and spot patterns. Below is an example of how a table can simplify a complex word problem:
| Item | Amount | Calculation |
|---|---|---|
| Price per item | $15 | |
| Number of items | 10 | |
| Total cost | $15 * 10 = $150 |
By structuring your work this way, you can keep track of your progress and reduce errors. It also makes the problem feel less intimidating.
By taking these steps, you can effectively manage complex problems, making the process less stressful and more systematic. Remember to check your work after each step to ensure accuracy and clarity in your solution.
Time Management During the Test

Effective time management is crucial when tackling an exam with multiple sections and a limited time frame. The ability to allocate time wisely can significantly impact your overall performance. By approaching the test methodically and setting clear time goals, you can avoid feeling rushed and ensure that you have enough time to address every question carefully.
Here are some strategies to help you manage your time effectively:
- Know the time limits: Familiarize yourself with the total duration of the exam and the number of questions. This allows you to calculate how much time you can spend on each section and question.
- Set time checkpoints: Break the exam into manageable intervals. For example, set a goal to complete a certain number of questions every 10-15 minutes. Keep track of time so that you don’t get stuck on one question for too long.
- Prioritize easier questions: Start with the questions you find easiest to answer. This ensures that you can rack up points quickly before tackling the more difficult problems.
- Don’t get stuck: If you find yourself spending too much time on a single question, move on. Mark it and come back later if time permits. The goal is to answer as many questions as possible, not to dwell on one.
- Review your work: If you have extra time at the end, use it to review your answers. Check for any mistakes or questions you may have missed. This final check can make a big difference in your score.
By practicing these strategies, you can reduce stress, increase efficiency, and improve your chances of performing well on the exam. Time management is not just about rushing through the questions; it’s about making the most of the time available to showcase your abilities.
How to Maximize Test Time
Efficient use of time during an examination is key to ensuring that you can complete all the sections thoroughly. It’s not just about working quickly but also about knowing when to move on and when to pause and reflect. With proper strategies, you can maximize every minute, ensuring you address as many questions as possible while still maintaining accuracy.
Here are some techniques to help you make the most out of your allotted time:
1. Plan and Prioritize
Before you dive into the questions, take a few moments to plan your approach. Skim through the entire section to identify the more straightforward questions. Start with those to build confidence and score easy points quickly. Once you have a rhythm, tackle the more challenging ones without spending too much time on any single question.
2. Stay Focused and Avoid Distractions
During the exam, it’s crucial to stay focused on the task at hand. Avoid distractions and resist the urge to second-guess yourself on questions you’ve already answered. Trust your first instinct unless you’re certain about a mistake. This helps prevent unnecessary delays in decision-making and keeps you on track.
Incorporating these strategies into your preparation routine can help you develop a better sense of timing and efficiency. Practice under timed conditions regularly to train your mind to respond swiftly while staying accurate. With experience, you’ll be able to approach each question methodically, without rushing, and complete the exam with confidence.
ACT Mathematics Test Format
The structure of the numerical reasoning section is designed to assess your problem-solving abilities across a wide range of topics. It includes questions that vary in difficulty, testing both basic skills and more complex concepts. This format challenges test-takers to demonstrate their understanding of mathematical principles, as well as their ability to apply these concepts efficiently under time constraints.
The section is divided into different categories of questions, each focused on distinct areas of mathematical knowledge. These typically involve topics such as algebra, geometry, and data analysis. The questions are multiple-choice, providing four options, where only one choice is correct.
Understanding the layout of this section and becoming familiar with the types of problems asked can greatly improve your performance. This will allow you to allocate your time wisely and approach each question with confidence. Regular practice with similar formats helps develop the skills necessary to navigate through the section quickly and accurately.
What to Expect in Test 2
In this section, you’ll face a variety of questions designed to assess your proficiency in core problem-solving areas. The problems will range from straightforward to more complex, testing not only your foundational skills but also your ability to apply more advanced techniques. Each question will focus on a specific concept, allowing you to demonstrate your understanding in different mathematical domains.
Here’s a breakdown of the types of questions you can expect:
Question Types and Topics

The section covers a variety of subjects, including but not limited to:
- Basic arithmetic and number operations
- Algebraic expressions and equations
- Data analysis and interpretation
- Geometrical shapes and properties
Difficulty and Time Management
The problems will increase in difficulty as you progress through the section. You’ll need to manage your time carefully to ensure you can answer all the questions within the allotted time. Efficient problem-solving strategies and practice will help you approach the questions with confidence and clarity.